Moment of Inertia of Torus

Question

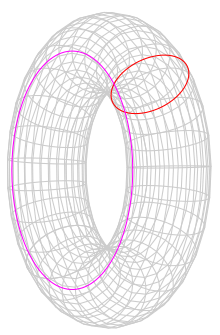
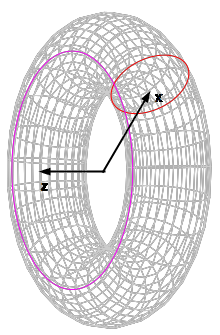
I was trying to find the moment of inertia of the Torus with respect to the axis in the center perpendicular to the bigger circle's plane; see image:

So: the radius of the magenta circle is $\rho$, the radius of the red circle is $R$, and I want $I_z$, $z$ being the axis that touches the center point of the magenta circle and is perpendicular to the plane of the magenta circle.

What have I done?

Well, first I integrated the moment of inertia of the disk with respect to a axis perpendicular to it's center, integrating small rings. My answer matched the tabled result: $\frac{1}{2} MR^2$. In this image, the $Z'$ axis:

Then, I used the perpendicular axis theorem to get the moment of inertia with respect to one axis that is a diameter of the circle (for example, $I_x'$ in the image). I got another expected result: $\frac{1}{4} MR^2$.

Ok, so now I use the parallel axis theorem to get the moment of inertia of the red disk in the torus with respect to the original $I_z$ axis. This result is obviously not tabled but the math is very simple and it gave me: $M(\rho^2+R^2/4)$.

Ok. That's for a disk of mass $M$. But if the disk has mass $\mathrm{d} m$, and they are all the same distance from the center axis, I just integrate all the disks in the torus to get the final moment. As you can see, this integral will just be a simple sum, and the result will be the same:

$\mathrm{d} I = \mathrm{d} M\left(\rho^2+R^2/4\right)$

But $\mathrm{d} M = \left(\mathrm{d} V / V\right) \cdot M$, the volume of the disk over the total volume times the total mass.

But the volume of the red disk is $\mathrm{d} r' \cdot A$, and the total volume is $2\pi\rho A$, so $\mathrm{d} M = \dfrac{M}{2 \pi \rho}\cdot \mathrm{d} r'$.

This depends on a new variable $\mathrm{d} r'$ that is independent from $R$ and $\rho$; see, those are fixed now. So when I integrate $\mathrm{d} r'$ form $0$ to $2 \pi \rho$, as expected, I get the same result: $I = M \left(\rho^2+\dfrac{R^2}{4}\right)$.

That seems good, but again that Wikipedia table says it should be $M \left(\rho^2+\dfrac{3R^2}{4}\right)$. And I have no idea where this $3$ factor is coming from.

Seems like a simple problem, but it's bugging me a lot.

Answer 1

A point on the surface of the torus is described by a rotated circle

$$ {\bf r}(\varphi,\theta) =(x,y,z) =\begin{bmatrix} \cos \theta & -\sin \theta & 0 \\ \sin\theta & \cos\theta & 0 \\ 0 & 0 & 1 \end{bmatrix} \begin{pmatrix} \rho + R \cos \varphi \\ 0 \\ R \sin \varphi \end{pmatrix} $$

A surface element on the torus is defined by

$$ {\rm d}A = \left\| \frac{\partial {\bf r}}{\partial \varphi} \times \frac{\partial {\bf r}}{\partial \theta} \right\|\; {\rm d}\varphi\, {\rm d}\theta = \left( R ( R \cos\varphi+\rho) \right) \; {\rm d}\varphi\, {\rm d}\theta$$

By scaling the small radius $R$ between $0 \ldots R$ we create a volume element

$$ {\rm d}V = {\rm d}A \,{\rm d}R = \left( R ( R \cos\varphi+\rho) \right) \; {\rm d}\varphi\, {\rm d}\theta\, {\rm d}R$$

The mass of the torus is

$$ m = ({\rm density}) \iiint {\rm d}V \\ m =({\rm density}) \int \limits_{0}^{R} \int \limits_{-\pi}^{\pi} \int \limits_{-\pi}^{\pi} \left( R ( R \cos\varphi+\rho) \right) \; {\rm d}\varphi\, {\rm d}\theta\, {\rm d}R $$

$$({\rm density}) = \frac{m}{2 \pi^2 \rho R^2} $$

The mass moment inertia tensor of the torus is

$$ I = ({\rm density}) \iiint -[{\bf r}\times][{\bf r}\times] {\rm d}V \\ I =({\rm density}) \int \limits_{0}^{R} \int \limits_{-\pi}^{\pi} \int \limits_{-\pi}^{\pi} \begin{bmatrix} y^2+z^2 & -x y & -x z \\ -x y & x^2+z^2 & -y z \\ -x z & -y z & x^2+y^2 \end{bmatrix} \left( R ( R \cos\varphi+\rho) \right) \; {\rm d}\varphi\, {\rm d}\theta\, {\rm d}R $$

---

$$ I = \begin{bmatrix} \frac{m}{8} \left( 5 R^2 + 4 \rho^2 \right) & 0 & 0 \\ 0 & \frac{m}{8} \left( 5 R^2 + 4 \rho^2 \right) & 0 \\ 0 & 0 & \frac{m}{4} \left( 3 R^2 + 4 \rho^2 \right) \end{bmatrix} $$

---

Confirmation

$$ \begin{align} R & = 5 \\ \rho & = 20 \\ m & = 77.67379 \end{align} $$

$$ I = \begin{bmatrix} 16,748.410 & 0 & 0 \\ 0 & 16,748.410 & 0 \\ 0 & 0 & 32,525.900 \end{bmatrix} \; \color{Green} \checkmark$$

Answer 2

Moment of inertia depends on the distribution of mass about the axis of rotation. If the mass of an object is rearranged in any way that keeps every element of mass at the same distance from the axis, the moment of inertia does not change.

So you can cut the torus along the red circle, unfold it into a cylinder, and then squash it to make a flat disk of radius $R$, with the red circle as its circumference. You found the MI of this disk about an axis through its centre and parallel to the z axis. Then you used the parallel axis theorem to find the MI about the z axis, which is distance $\rho$ from the centre of the disc.

The above calculation is plausible but flawed. The mistake is that you assumed the red disk has a uniform density. It does not. In the torus the amount of mass increases with radius from the z axis. When the torus is opened and straightened to make a cylinder, the inner side must be stretched, reducing density, while the outer side has to be compressed, increasing density. So the density of the red disk increases with distance from the z axis.

This also affected your use of the parallel axis theorem, because this theorem uses the distance of the centre of mass of the disk from the z axis, not the distance of the geometrical centre of the disk from the z axis.