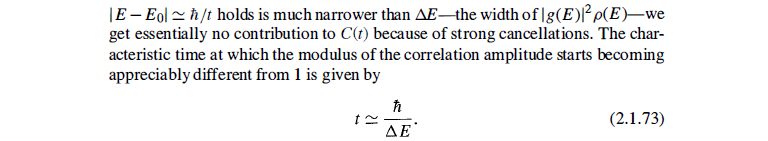1
$\begingroup$

The following is a section "Correlation Amplitude and the Energy-Time Uncertainty Relation" from Sakurai's Modern Quantum Mechanics book page 79:

enter image description here enter image description here

Question:

  • Why does it state that the oscillations are rapid unless $|E-E_0|$ is small compared to $\frac{\hbar}{t}$? Also, what is the particular importance of the interval $|E-E_0| \approx \frac{\hbar}{t}$ being narrow compared to $\Delta E$ (defined as the width of $|g(E)|^2\rho(E)$) which I think allows us to assume that $|g(E)|^2 \rho(E)$ is approximately constant (which then implies that $C(t)$ is zero by allowing us to take $|g(E)|^2 \rho(E)$ out of the integral (2.1.72) and hence an integral on an oscillating function of that kind is zero)?
Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
0
$\begingroup$

For the first question, I like to think of things this way: if you evolve long enough you will begin to notice that the integrand oscillates and is not monotonic this means you will begin picking up positive and negative areas under your integral causing cancellations. In order to avoid this, either do not evolve long enough or have exceedingly low energies so that your period is very large.

Now you could ask at what scale will one being to notice this cancellations? It will be roughly $|E-E_0| \approx \hbar/t $. Now we know that our integrand is peaked at $E_0$ with width $\Delta E$, but if this scale is much larger the our $|E-E_o|$ scale i.e contains the domain of massive oscillations then when integrate we will be getting the scale where we have massive cancellations and therefore getting something close to zero.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks I understand much of your answer, but could you elaborate on why if $|E - E_0| << \frac{\hbar}{t}$ that we would not get rapid oscillations? How does it imply long period mathematically? $\endgroup$
    – user100411
    Commented Apr 11, 2017 at 14:18
  • $\begingroup$ I think I understand, if we consider a simple plane periodic wave: $\sin(\omega t)$ then in analogy with this example, in the integrand $\omega := \frac{t}{\hbar}$ and $t := (E - E_0)$ (with this is the $t$ from $\sin (\omega t)$). Hence $\omega = 2 \pi f$ implies $\frac{t}{\hbar} = 2 \pi f$ implies that the period $T = \frac{2 \pi \hbar}{t}$. Hence if the period $T = \frac{2 \pi \hbar}{ t}$ is very large compared to $E - E_0$ then the wave has not traversed sufficiently to have enough oscillations to cancel sufficiently. Is this basically the idea? $\endgroup$
    – user100411
    Commented Apr 11, 2017 at 14:34
  • $\begingroup$ @JohnDoe, $\omega:= \frac{E-E_0}{\hbar}$ This makes the units work out. Now $\omega$ is related to the period of your oscillations for $ \sin \omega t$. For times of the order $ \frac{1}{\omega} \approx T $ you are beginning to see the function oscillate and so when you integrate you begin to pick up negative and positive areas under the graph. So either don't evolve all the way to times of order the period (T) or make $\omega$ small or your period large $\endgroup$
    – Amara
    Commented Apr 11, 2017 at 20:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.