Resultant moment of forces in four directions?

Question

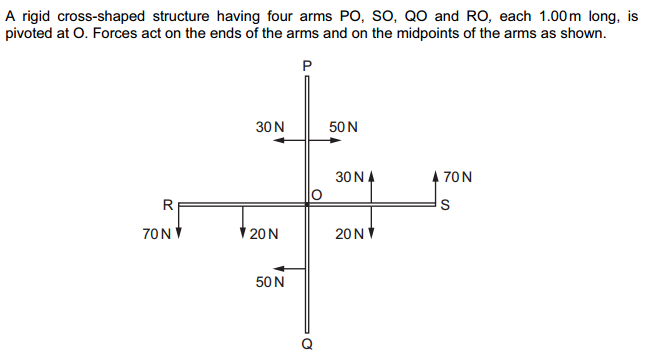
Finding the resultant moment for two directions is simple enough. However when there are forces in four directions (at right angles) how do you add the moments for the two axises?

For instance in the following example there is a moment of 5Nm downwards (or anticlockwise) and of 15Nm to the left.

I thought as both these moments were anticlockwise you could add them but the correct answer is 120Nm (a far cry from my 20Nm).

So how do you add moments in more than two directions?

Answer 1

You should add all the moments separately.

You are summing all the forces together when you shouldn't (based on the little amount of info you provided on how you answered this). If you do sum them, you have to account for the couple moments (the forces may be equal and opposite, but the moments add up to a bigger one, not cancel since the are on different sides of the origin). Try finding the moment for each force on it's own then adding them after.

When forces aren't applied on the centre of a body, you can cancel the forces, but it can still result in a moment about the centre.

Answer 2

Let us denote clockwise as positive moment and counterclockwise as negative. And let us be a little bit smart such that we do not need to calculate a whole lot.

As JMac already told you, you should add all torques individually, here is the full method:

• Forces at the ends of the rods: There are only two forces at the end of the rods and they both push in the - direction given rise to a total torque: $-70N\cdot1m-70N\cdot1m=-140Nm$
• Forces at the middle of the rods: Adding all these up with a - for the ones that are pushing counterclockwise and a + for the ones that are pushing clockwize gives us: $(50+20+50-30-30-20)N = 40N$ giving rise to a total torque $40N*0.5m = 20Nm$

Therefore the total torque is $-120 Nm = 120 Nm "counterclockwise torque"$

I hope this helps you find your mistake :)