Angular velocity of a rigid object about an axis outside of the body

Question

I know that for a rigid body rotating around a fixed axis, the angular velocity of any point with respect to any other point is the same. As a result, the angular velocity is the same for any choice of axis attached to the body as long as that axis is perpendicular to the rotational plane. But how about the angular velocity about an axis outside of (or not atttached to) the body? It is still the same as that of the axis inside of the body? If not, is there a general equation relating the two?

Answer 1

Imagine that the rigid body is fixed in the $Oxy$ co-ordinate plane. The rigid body need not overlap the origin $O$. If the co-ordinate plane is rotated (eg about the origin $O$) then every line in the plane rotates through the same angle, regardless of its position. Each of the two points at the ends of any line rotates through the same angle about the other. This is true whether the two points both lie inside the rigid body, or one inside and one outside, or both outside.

Angular velocity is the rate of rotation, so what is true about angles applies also for angular velocity.

Consequently, when an axis is chosen outside of a rigid body, the angular velocity is only the same as that measured within the rigid body if the axis rotates as though it were part of the rigid body. For example, the whole co-ordinate plane could be regarded as a rigid body which has finite density inside the region occupied by the object of interest and zero density outside of the object in the rest of the plane.

---

The constant angular velocity $\omega$ between two points within the rigid body is intrinsic to the rigid body. It does not hold between two points one inside and one outside of the rigid body.

If the external axis (origin $O$) rotates relative to the rigid body this is equivalent to the rigid body rotating relative to the co-ordinate system $Oxy$. In this case, in general the angular velocity $\Omega_P$ of a point $P$ within the rigid body relative to $O$ is not only different from the intrinsic angular velocity $\omega$ within the rigid body, it is also not constant.

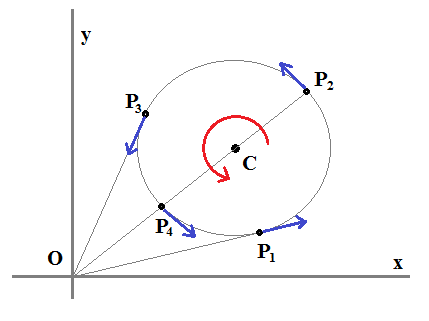
Suppose some point $C$ within the rigid body is at rest (perhaps instantaneously) in the $Oxy$ frame. Any point $P$ within the rigid body rotates about $C$ with constant angular velocity $\omega$. The angular velocity $\Omega_P$ of $P$ about $O$ varies as $P$ rotates about $C$. At $P_1, P_3$ the velocity of $P$ is aligned with the vector $OP$, so $\Omega_P$ is zero. At $P_2, P_4$ the point $P$ is aligned with vector $OC$ and $\Omega_P$ reaches a local maximum.

If the centre of rotation $C$ is rotating about $O$ then $\Omega_P$ is even more difficult to calculate except in special cases. One special case is when $C$ rotates about $O$ with the same angular velocity $\omega$ with which $P$ rotates about $C$. Then the angular velocity $\Omega_P=\omega$.