Time dilation of distant cosmic events. What is it?

Question

I was reading the Wikipedia's page about "tired light", where we read that any alternative explanation to the observed redshift (described by Hubble's law) should be able to overcome several objections, among which "the time dilation associated to cosmologically distant events".

Cosmologically speaking, I only know the Shapiro delay, i.e. nothing to do with Hubble's law. And of course the time which light needs to reach us and to let us detect a given cosmic event. Moreover, in Einstein's relativity light is not itself affected by time dilation (only clocks are and, broadly, any macro/micro mechanical phenomenon). Even the gravitational redshift deals with the clocks used to measure light's frequency (different gravity on different clocks), not with light itself. So, what is the time dilation associated to "distant" cosmic events and how do we measure it?

Answer 1

The cosmological redshift can (and probably should be) interpreted as time dilation due to the expansion of the universe. Photons that are emitted in one part of the universe travel across space, but as they do so, they are subject to time dilation, manifested as a decrease in their frequency, caused by the expansion of the universe. That is, the ticks of a clock in the frame of reference of the emitter are observed to be dilated in a different co-moving reference frame that is some distance from the emitting frame. [Here you may be misunderstanding something - the frequency of light is a clock; the period between maxima in an electromagnetic wave act like ticks of a clock.] This cosmological redshift looks like a Doppler shift and is not observationally distinguishable from a Doppler shift.

Proponents of the tired light hypothesis claim that this cosmological redshift could be reproduced if photons somehow lose energy as they cross the universe and hence have a decreased frequency in the observers frame. Among the fatal problems for this class of hypotheses is that if there exists a class of events in the universe that have a fixed duration, the tired light hypothesis predicts that their duration should be unaffected when observed at great distances. On the contrary, in the expanding universe one expects the observed duration to be stretched by a factor $1+z$ (where $z$ is the redshift); exactly the same factor by which wavelengths are redshifted for light observed from the the same events.

This method was proposed by Wilson (1939) as a test of the expanding universe theory rather than the "gradual dissipation of photonic energy" (aka "tired light").

The test has been passed with flying colours using the light curves of type Ia supernovae. These events, caused by exploding white dwarfs can be seen right across the universe. They are classed as standard candles, in that the explosion occurs in a very standardised way with a "bomb" of more-or-less fixed mass. The luminosity of the event appears to rise and fall in a very consistent manner, such that the width (there are varying definitions of this) occupies a fixed length of time with a very small dispersion that is closely related to the peak luminosity of the supernova. A typical set of light curves are shown below (from Perlmutter 2003).

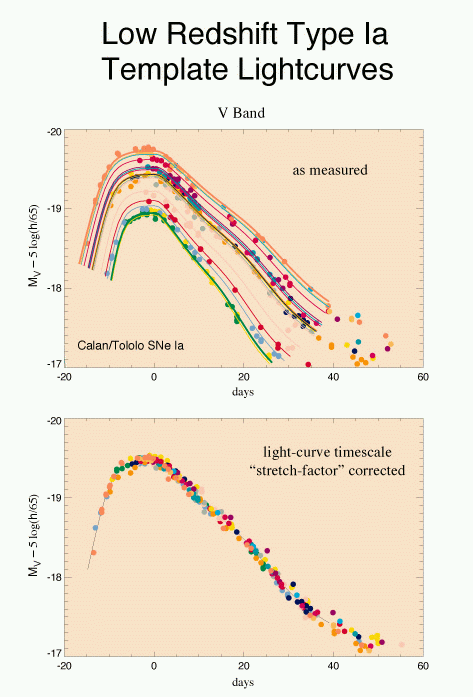
However, it is observed that the light curve peaks of Type Ia supernova become broader at higher redshifts. The amount of broadening is exactly in proportion to the amount of redshift $(1+z)$ observed in the spectra of the same supernovae. i.e the cosmological time dilation works as expected for an expanding universe, see for example Blondin et al. (2008). A "gradual redshifting" of light as it travelled a distance cannot explain this time dilation.

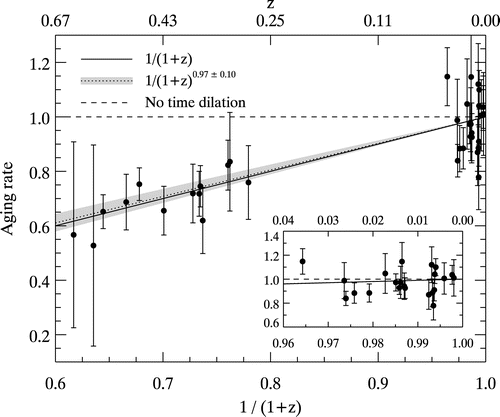
The plot below shows how the measured "de-dilation factor" (what you need to correct the widths of high redshift supernovae to match the widths of local supernovae) from supernova light curves depends on their redshift (from Blondin et al. 2008). It goes as $1/(1+z)$, exactly as expected (well to within 10%). The horizontal dashed line is what would be expected from "tired light" and is rejected at 10 sigma.

Any alternative hypothesis for why the redshift of galaxies is proportional to their distance (for small $z$; it is a more complicated relationship at high $z$) must also explain why the duration of type Ia supernovae is also stretched by the same factor $(1+z)$. Or, if it is not to be some arbitrary change in the properties of type Ia supernovae with time, then an alternative theory must explain why photons emitted at the start of a supernova explosion appear to take take less time to get to us than those emitted towards the end of the supernova.

Edit: It has to be said there has been little activity in this area recently. Blondin et al.'s results have been questioned by a couple of authors (e.g. Crawford 2017). The dispute appears to be how light curves taken at different redshifts, and therefore seen at different intrinsic wavelengths if viewed with same filter, can be calibrated onto the same system.

Another piece of evidence comes from Gamma Ray bursts. Zhang et al. (2013) find that the duration of "long" GRBs at known redshifts (and measured in the same rest-frame energy) varies as $(1+z)^{0.94\pm 0.26}$, consistent with cosmological time dilation. See also Littlejohns & Butler (2014).

More support for cosmological time dilation has now emerged from looking at the timescale for quasar variability as a function of redshift. Lewis & Brewer (2023) surveyed nearly 200 quasars out to $z=4$ and considered their variability in bins of similar rest wavelength and bolometric luminosity. They found the observed characteristic timescale varied as $(1+z)^n$, with $n=1.28^{+0.28}_{-0.29}$.

Answer 2

In the standard cosmological model the cause of red shift in light from distant cosmoligical objects is relativistic time dilation due to the theorised expansion of the universe.

The observed phenomena that theories must explain is red shift. Time dilation due to cosmological expansiion is the existing most widely accepted theory, with which any such theories compete. Also when you say light is not impacted by time dilation, that is wrong, in relativity it is red shifted due to time dilation, (the oscilations appear to the observer to slow down).

"Tired Light" is new to me but it looks simple like a fairly simple theory that red-shift, which is a reduction/loss of energy of photons, (energy is proportional to frequency) could be explained by light colliding with particles it encounters on its journey and then being re-emitted. That theory has (at least one) unexplained flaw, which is that the light would be scattered, and would not travel in a straight line.

A light clock is a good example to ilustrate it, and the light operating the clock behaves just like any other light that may be observed

Answer 3

I think the other answers haven't adequately explained this.

The "time dilation" that the Wikipedia article talks about is a universal property of Doppler shifts, even Doppler shifting of sound. If someone shouts something at you while moving toward you so quickly that their voice sounds an octave higher, they will also seem to be talking twice as quickly. The same phenomenon that doubles the received oscillation speed of a simple sine wave will just as happily double the speed of a wave of any shape. It is not normally called "time dilation", but I'll use that term in this answer.

The frequency shifts in cosmology are Doppler shifts. There is no well defined difference in general relativity between so-called cosmological, gravitational, and special-relativistic redshifts. They're all special cases of the same phenomenon, namely time dilation as defined above.

Non-expanding steady-state cosmological models can't reproduce the observed time dilation because they are homogeneous in both space and time.

  time --->

----EEEE-------
    \   \
     \   \
      \   \
-------DDDD----

In this diagram, ---EEEE--- is the worldline of a clock that determines the time at which two signals are emitted (for example, two characteristic moments in the evolution of a type Ia supernova, which are separated by weeks), and ---DDDD--- is a clock on Earth that measures the time between detection of the signals. In a steady-state model, the symmetries guarantee that you can translate the EEEE part of the first clock's worldline diagonally in the spacetime until it overlaps DDDD, showing that they must be the same length, i.e., that there is no time dilation.

In the standard expanding cosmology, although you can draw a similar diagram, the argument doesn't go through because there is a scaling factor that varies with time, so you can translate the worldline vertically but not horizontally in the diagram.

Answer 4

mfc: Neither the accelerated expansion of the universe nor gravitational time dilation (ie general relativity) are ‘hypotheses’: these are two of the most confirmed and corroborated facts about the universe that have ever been established. You can even do (and do do) the verification/ ‘measurement’ of GR at home, by using GPS http://www.scielo.org.za/pdf/sajs/v104n5-6/a1410406.pdf

Likewise, the accelerated expansion of the universe is endlessly measured and corroborated scientific fact. (cosmological redshift, cf Brian Schmidt et al).

The point is that, with an acceleratingly expanding universe general relativistic universe, cosmological redshifting can only consistently be understood as a result of cosmological time-dilation. This is given the constancy of the speed of light (and, therefore of distance, as speed is distance/ time). Given these constants, time is the only variable. (Obviously this is not true on all reference frames, because of special relativity, but is true with enough collated reference frames from all directions, ie across spacetime, because of general relativity). Cosmological Redshift, therefore, must understood as the effect of cosmological time-dilation caused by an (accelerating) expansion of space itself. In fact, we can (and have) measure(d) time dilation by comparing the redshifting of wavelengths of photons emitted from known distances(such as type 1a supernovae). If you have another theory of cosmological redshifting consistent with relativity that doesn’t involve cosmological time dilation, I’m all ears (as, I’m sure, is everyone).

Answer 5

Rob, you say "the frequency of light is a clock". I cannot agree. In physics we only deal with things we can "measure". Everytime and everywhere you measure the frequency of light you do that thanks to an instrument. This instrument has an internal clock which is subject to gravity. The same light ray has different frequencies when measured in points where gravity is different. This is the gravitational redshift (or Einstein's redshift). But I think to have an answer to my question after having read more. Where Wikipedia says that a theory for the cosmological redshift has to explain the time dilation associated to this phenomenon it is wrong, and they should correct that, since time-dilation is "a hypothesis" to explain the cosmological redshift of light coming from distant galaxies, "not a matter of fact". I mean, time delation is bound to the hypothesis of expansion: we cannot say (as on Wikipedia) that a new hypothesis has to confirm the previous (mainstream) hypothesis (!). Again, what we can trust is what we can measure. We simply measure a redshift. We don't have definitive (proven) theory to explain that though the mainstream is the most effective considering the observations. But it is not immune from criticism, especially when we try to apply time dilation to photons (not to clocks), for the reasons above. Zwicky's tired light based on occasional Compton scattering photon-electron during light's travel doesn't work for several reasons. But if we hypothesize, for instance, a non-zero viscosity of the intergalactical medium (e.g. dark energy) we can as well explain Hubble's law (in the form z=DH_0/c), we'd have a stronger redshift the further a galaxy is, without accelerated expansion.