"Unaccounted for" vertices in certain Feynman diagrams?

Question

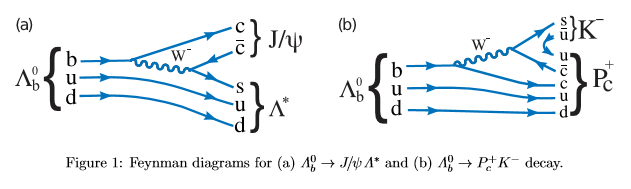
I'm looking to understand where certain "unexplained" vertices in some Feynman diagrams come from, in a physical sense. For example, in the top figure (Aaij et al. 2015), in diagram (b), there is an "unaccounted for" $u\bar{u}$ pair that seems to come from nowhere.

Are these quarks similar to the spectator quarks from the initial $\Lambda_0^b$, or do they arise from the virtual quark/gluon sea somehow? If they come from the quark sea, how can they come to comprise real, observable particles like the kaon and $P_c^+$?

^{Image source: Observation of $J/\psi p$ resonances consistent with pentaquark states in $Λ^0_b→J/\psi K^−p$ decays, LHCb collaboration. Phys. Rev. Lett. 115, 072001 (2015), arXiv:1507.03414.}

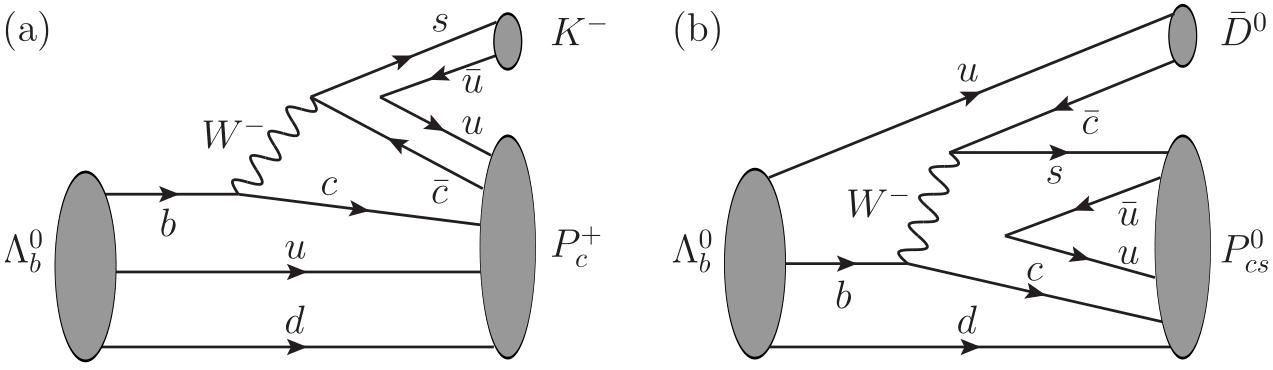
^{Image source: Hidden-charm molecular pentaquarks and their charm-strange partners, R. Chen et al. Nucl. Phys. A 954, 406-421 (2016), arXiv:1601.03233.}

Answer 1

Once you have quarks in a Feynman diagram, the strong interaction enters, and note, the strong, it means Quantum Chronodynamics . It means from that point on the usual perturbative expansion with the nice fixed rules for calculating the total integral with electromagnetic and weak interactions can only be used with approximations for the QCD part, which are highly divergent using the usual tools. QCD calculations use different tools, like lattice QCD.

In the diagram with the u u_bar vertex, what is implied is a gluon connecting the two quark lines, (a number of possible combinations) as for example shown in this link. The fact that the authors do not use a gluon line is because that line is not really a single particle exchange simply under the integrals, but a complicated approximation on strong force exchanges that are allowed within the kinematics. A gluon giving the vertex would make it simple, but would not stress the real complexity of the mathematical situation.

In the top diagrams, the spectator approximation is implied. As far as stron interactions go there should be innumerable gluon-quark vertices between the quarks, keeping them within a hadron.