Are decay Feynman diagrams really Feynman diagrams too? Or just vertices?

Question

So I was wondering, the vertex diagrams for the standard Model, can they also be feynman diagrams with on shell particles? For instance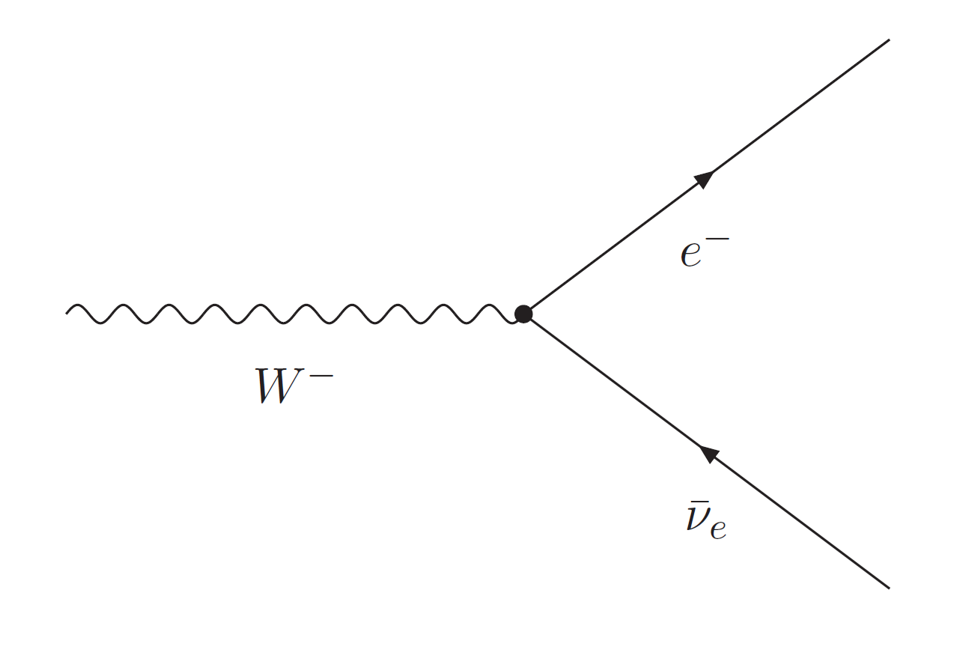 here is the W - boson vertex which decays into electron and neutrino. But since all particles are external that means they would all have to be on shell. Yet how does this work if there is no propagator?

Answer 1

Any diagram that is allowed by the Standard Model Feynman rules which has an external $W$, $e$, and $\nu$ is an allowed diagram in the $W^{-}\to e^{-}\overline{\nu}_e$ decay. This is true whether or not the corresponding diagram has an internal propagator.

This is true whether or not the external particles can be put on-shell. For instance, if we consider the decay process $e^-\to e^-\gamma$, it is known that this decay cannot be put on shell. Regardless, we can define an amplitude $\mathcal{M}$ for such a process. The fact that this process cannot be put on-shell, however, manifests itself whenever we try to calculate the decay rate. We have

$$\Gamma\propto\int\mathrm{d}(\text{phase space})|\mathcal{M}|^2\,\delta^{(4)}(\text{momentum}).$$

Since the delta-function $\delta^{(4)}(\text{momentum})$ that conserves momentum is always zero, the corresponding decay rate vanishes (this is modified when we consider QED in a dielectric medium, in which case the decay process corresponds to Chereknov radiation).

Back to your example, it is perfectly valid to construct (a perturbative approximation to) an amplitude $\mathcal{M}$, and simply use it to calculate the decay rate, regardless of whether or not the process is kinematically allowed. Fortunately, in this case, the process is kinematically allowed.

Answer 2

This diagram is the lowest order contribution to the amplitude $\mathcal{M}$. With that amplitude you can compute decay rates in the usual way, using $|\mathcal{M}|^2$. Propagators are related to intermediate particles. They add higher order corrections to the black dot. You will often see in books that the black dot is then changed into a grey circle to show that it includes these higher order corrections. These higher order terms make the computation more difficult, but fundamentally not different.