Discrepancy in center of mass of a uniform hemispherical shell of zero thickness

Question

This came up in my physics class.

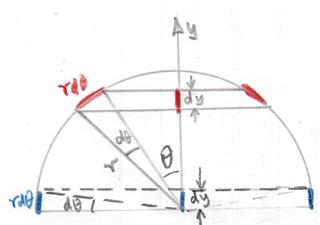
Suppose a uniform hemispherical shell has a thickness of 0 and a mass of M and a surface density of $\sigma =M/(2\pi r^2)$. My class derived two ways to integrate it: the first way was to horizontally slice the shell into concentric circles with radius of $(r^2 - y^2)^.5$ and integrate as: $$ y(com) = ((\int_0^r \sigma 2\pi ((r^2-y^2)^.5)dy)y)/M $$ Doing this method gives an answer of $y(com) = r/3$. However, doing the other method of integrating as a function of $\theta$ is: $$ y(com) = ((\int_0^(\pi/2) \sigma 2 \pi (rcos\theta ) rd\theta)rsin\theta)/M $$ Doing this method gives an answer of $y(com) = r/2$

My entire class combined could not find any math errors in either method. What is the discrepancy?

Answer 1

The second method is the correct one.
You will note that the first method places the centre of mass of the shell closer to the centre of the open end of the hemisphere than the second method.
It does that because in the first method you are underestimating the masses of the elements which are remote from the open end as you can see in the diagram below.

Update

When $\theta$ is near $90^\circ$ the blue heights of the sections are approximately the same $dy \approx r \;d\theta$ and so the masses of the elements are the same for both methods.

As $\theta$ becomes smaller (red elements) then $r\; d\theta$ becomes progressive much larger than $dy$.
So your first method underestimates the mass of the elements when $\theta$ becomes smaller.