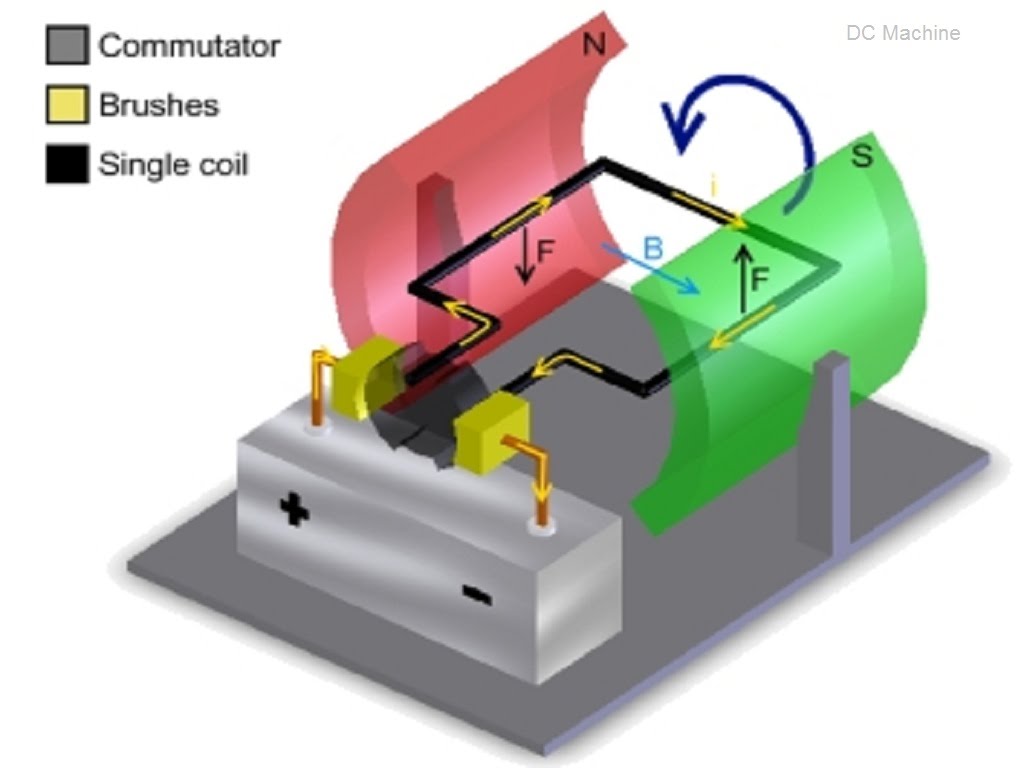
What you have illustrated in the link you provided is the conceptual model of a DC Brush Motor where the commutation of current to supply the armature field is accomplished by a concentric series of contacts on the armature and brushes that remain stationary with the stator windings.
I'll break down, and answer your questions one by one...
...but how does the motor mange to achieve approximately constant
angular velocity some time after start despite having angular
acceleration due to the torque?
Your guess was correct. Friction in the bearings and windage (drag) create a torque in opposition to the electromagnetic torque imposed by current between the stator and armature windings. The motor, upon accelerating eventually reach an angular velocity where an equilibrium is achieved between these torques.
If it is due to friction, then how can we make standard rpm DC motors
(without gearboxes)?
The operating speed of brush motors, as specified by the manufacturers is an approximate speed, determined at specific operating conditions. To achieve a more precise operating speed, feedback is often used: encoders or Hall sensors sense the rotational speed and feedback into a controller that modulates the size or timing of current to approach the desired speed.
Also, is the effect of torque (or the angular velocity achieved)
countered by the momentary equilibrium established at the point when
the armature is perpendicular to the magnetic field (moment parallel),
before the current direction is switched by the commutator ?
Because of commutation (switching) of the current and the characteristic impedance of the windings (mostly inductive resistive) the actual torque is not uniform over a full rotation of the motor, but rather has a cyclic low and high torque. So what you really have coming to an equilibrium between friction and electromagnetic torque is an average torque over the full rotation. Maximum torque is achieved when the fields are in full quadrature with one another. And that has allot to do with the specific geometry of the windings.