14
$\begingroup$

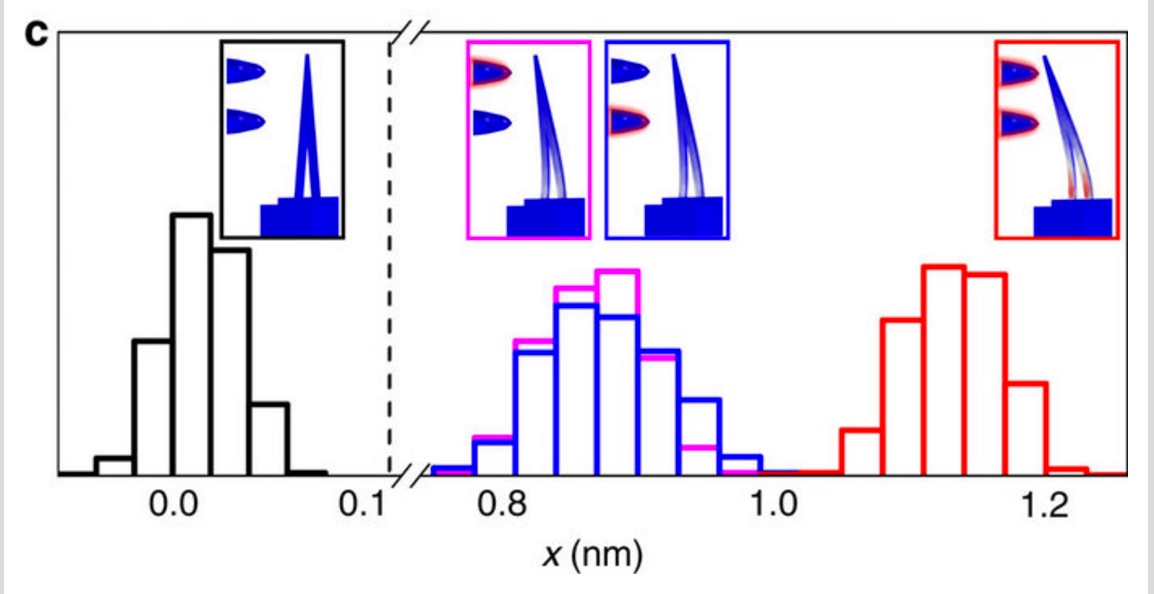
This news, summarizing results from

M. López-Suárez et al. Sub-$k_B T$ micro-electromechanical irreversible logic gate, Nature Commun. 7, 12068 (2016).

Makes the claim that

It clearly shows that there is no such minimum energy limit and that a logically irreversible gate can be operated with an arbitrarily small energy expenditure. Simply put, it is not true that logical reversibility implies physical irreversibility, as Landauer wrote.

The results of this experiment by the scientists of NiPS Laboratory at the University of Perugia are published today in Nature Communications. They measured the amount of energy dissipated during the operation of an "OR" gate (that is clearly a logically irreversible gate) and showed that the logic operation can be performed with an energy toll as small as 5 percent of the expected limit of kBT ln2. The conclusion of the Nature Communications article is that there is no fundamental limit and reversible logic is not required to operate computers with zero energy expenditure.

First of all, is this for real?

If so, what was wrong with Landauer’s analysis?

Improve this question
$\endgroup$
5
  • $\begingroup$ Landauer's ideas on the connection between logical and thermodynamic reversibility are controversial. See for example dx.doi.org/10.1016/j.shpsb.2004.11.006 $\endgroup$
    – Ján Lalinský
    Commented Sep 3, 2016 at 10:57
  • $\begingroup$ I think there is a problem with the notion of energy needed to operate the gate. I agree that this can be done with arbitrarily low energy. However, as far as I understand, this is not what Landauer is about: it's the fact that the "forgotten" gate state must wind up somehow encoded in the quantum state of the system, thus increasing the latter's entropy that is the crux of the matter. One then has to input work to throw this excess entropy out of the system and restore the original system macrostate. $\endgroup$
    – Selene Routley
    Commented Sep 7, 2016 at 5:58
  • $\begingroup$ @WetSavannaAnimalakaRodVance so what’s the problem? Will it “fill up” somehow if operated repeatedly? $\endgroup$
    – JDługosz
    Commented Sep 7, 2016 at 6:17
  • $\begingroup$ @JDługosz The system's macrostate has changed subtly with each operation of the gate. Ultimately, the system would be so thermalized (overheated) that it wouldn't work anymore. The situation is somewhat analogous to my answer here, where one seems to be able to access the whole enthalpy of a reaction as free energy. One can do so, but not repeatedly, because the reaction leaves the macrostate of the system used to pull the trick off subtly changed. $\endgroup$
    – Selene Routley
    Commented Sep 7, 2016 at 6:32
  • $\begingroup$ BTW, I think this is quite an interesting question that hasn't gotten as much attention as it really ought to- I would encourage you to put a bounty on it if you aren't satisfied with the current answers. $\endgroup$
    – Rococo
    Commented Sep 7, 2016 at 22:10

3 Answers 3

Reset to default
6
$\begingroup$

I'm not completely confident that I am understanding their claim, but I will risk a comment anyway. There is one obvious question that comes to me and doesn't appear to be directly addressed in the paper.

Here's the key figure showing their system:

enter image description here

It is a little cantilever which can be pulled about the same amount by two electrodes, which are the two inputs to the gate, and when both electrodes are on it is pulled around 1.5x as much. Then they say that if you set the digital logic threshold just above the position when it's not being pulled, it is an OR gate.

Formally this is true, but in reality you can't just tell your circuit "this is the threshold, and you are to regard these two signals as identical." You have to have something else that actually takes those two different outputs and has the same reaction to either of them. This is the step that would require some sort of erasure and be subject to Landauer heating.

As such, it seems to be that if you tried to scale this type of gate up you would end up with a dilemma:

  1. Either you maintain the condition of having many physical states correspond to a logical state, which seems like it would quickly become infeasible because your range of inputs that you accept as a "1" would have to grow (in this case, roughly linearly) with the number of bits. For a modern microprocessor, this would mean accepting a signal that varied over, say, 9 orders of magnitude.

  2. Or, at some point you consolidate all of the physical states corresponding to a logical "1" to the same output, and pay the cost of Landauer heating for erasure.

Of course, this does not preclude this sort of reversible digital scheme from having some sort of specialized application at a smaller scale. not to mention its utility in clarifying exactly what the Landauer limit really does and does not say. But for a scalable computer, I'm not sure that the claims they make are really justified.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Did you look at the electrostatic-deflection-logic examples of the inverter and the full adder? It seems that threshhold of 0 being not deflected and 1 be deflected (whether a little or a lot) propagates directly to the downstream elements. $\endgroup$
    – JDługosz
    Commented Sep 7, 2016 at 6:25
  • $\begingroup$ @JDługosz I did- I agree with this but my concern is whether over many gates the '0' (in the case of the NOR gate) might spread over more and more physical states and become in some cases arbitrarily close to the threshold, unless an erasure step was preformed. $\endgroup$
    – Rococo
    Commented Sep 7, 2016 at 22:09
4
$\begingroup$

I think I am agreeing with the content of Cort Ammon's answer.

I think the paper's "error" is to focus on the notion of energy needed to operate the gate. I agree that this can be done with arbitrarily low energy - that is very clear.

However, as far as I understand, this is not what Landauer is about: it's the fact that the "forgotten" gate state must wind up somehow encoded in the quantum state of the system (because the laws that govern it are microscopically reversible), thus increasing the latter's entropy that is the crux of the matter.

One then has to input work to throw this excess entropy out of the system and restore the original system macrostate. If one tried to pull the "trick" of the paper off repeatedly, one would indeed seem to defeat Landauer's principle in the short term. But the hardware of the system would become more and more thermalized as the experiment were repeated and the computer would cease to work.

Improve this answer
$\endgroup$
3
+100
$\begingroup$

Edit: my first answer was wrong.

What this paper appears to be doing is creating a reversible element which is being treated logically as an irreversable OR. Because the element itself is reversible, it can easily avoid Landauer's principle.

As best as I can tell, this has been known for a long time: you can have a combination circuit of reversible logic, and you only pay the energy cost for the measurements taken at the end of the process (which must latch, so are subject to Landauer's principle).

Improve this answer
$\endgroup$
11
  • $\begingroup$ So is it possible that the conclusion of no such limit for useful computation is true? As opposed to useless e.g. it costs more energy to maintain the conditions? $\endgroup$
    – JDługosz
    Commented Sep 3, 2016 at 23:52
  • $\begingroup$ @JDługosz I would have to know more than I do to say for sure, but it looks reasonable to say that there may be no such limit if you can maintain the system in an excited state during the calculation. If you ever let the system relax back to thermal equilibrium. Theoretically if you could get the system into a perfectly metastable state, and keep it there during your computations, it would take no extra energy to maintain that excited state, and thus no lower limit on energy cost. Practically speaking, there may be some algorithms for which this is easy, and some for which it is hard. $\endgroup$
    – Cort Ammon
    Commented Sep 3, 2016 at 23:56
  • $\begingroup$ Thanks for making me read that paper, btw. The idea of physically reversible but logically irreversible operations has a lot of interesting implications in how we make logical decisions as living biological creatures. I don't think we're playing games with trying to beat Landauer's limit with biology any time soon, but it makes me wonder if we're using a similar approach to be more efficient with computation in our own heads. $\endgroup$
    – Cort Ammon
    Commented Sep 3, 2016 at 23:59
  • $\begingroup$ It bothered me that the summary said there was no limit, as opposed to saying this limit is refuted, the actual meaurement is a value above any remaining limits that may exist. $\endgroup$
    – JDługosz
    Commented Sep 4, 2016 at 0:00
  • 2
    $\begingroup$ @JDługosz Yeah, they are probably overstating their conclusion. I find that is typical in science. We're more than happy to make ontological statements like "there is no limit" as long as our currently preferred model shows no limit. We then happily change our ontological statement once a new model takes over. $\endgroup$
    – Cort Ammon
    Commented Sep 4, 2016 at 0:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.