Introduction to Special Relativity Question - Momentum Conservation

Question

I'm currently reading a text for self-study on special relativity, Introduction to Special Relativity by James H. Smith, and I came across a question that I don't see to grasp at the moment.

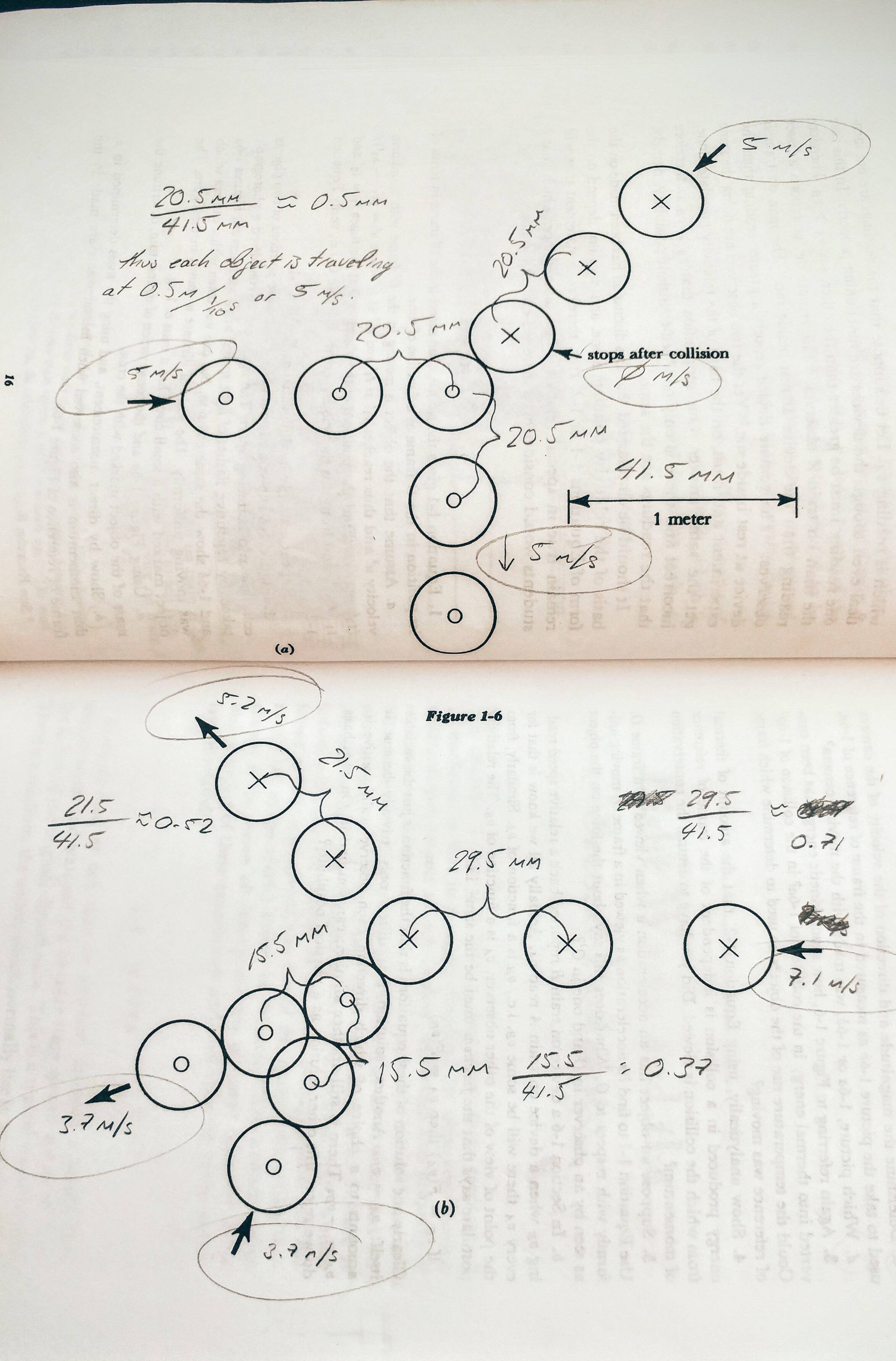
"Figure 1-6 shows a diagram of the successive positions of two objects colliding and bouncing apart. The figure can be considered a photograph taken by repetitive flashes of light spaced at $\frac{1}{10}$ sec intervals. Figures 1-6*a* and 1-6*b* show the same collision, but one was taken with a camera which was moving uniformly. The scale of distance is shown in the figure. The object marked with a small circle has a mass of 1 kg.

a. Using Figure 1-6*a* and the conservation of momentum, determine the mass of the object marked with the cross.

b. Show by direct measurement, and using the mass determined in a, that momentum is also conserved when measurements are made in the frame of reference of Figure 1-6*b*."

My answers:

a. Using the conservation of momentum ($u$ being velocity before collision and $U$ being velocity after collision):

$$ m_ou_o + m_xu_x = m_oU_o + m_xU_x $$

Since 'x' object stops after collision in figure (a) [top], $U_x = 0m/s$: $$ m_ou_o + m_xu_x = m_oU_o + 0 $$ $$ m_x = \frac{m_o(U_o - u_o)}{u_x} $$ According to the diagram, we see that $u_o = U_0 = u_x = 5m/s$: $$ m_x = \frac{1kg(5m/s - 5m/s)}{5m/s} = 0??? $$ Something is not right here. The only way this would seem to me to make sense would be where the frame suddenly begins to move immediately after the collision, and we can account for some of the velocity with the moving frame, but the question clearly states that one of the frames, either (a) or (b), is continuously moving with a uniform speed. Momentum is conserved for ALL inertial frames.

b. Again, plugging values into the conservation of momentum equation, using the velocities found in figure (b) [bottom], things don't add up:

$$ 0.72m_o + 0.38m_x = 0.51m_o + 0.38m_x $$

Is there something I'm doing wrong or not understanding, or are the diagrams not accurate?

Thanks in advance :)

Answer 1

Momentum & velocity are vectors, not scalars. This means that you can't just set up one equation for "momentum conservation" like you can do with energy conservation, but instead have to look at each coordinate direction independently. In part a of your problem, the equation for momentum conservation in the $x$-direction (horizontally along the page) would be $$ m_0 (5 \text{ m/s}) + m_x (5 \text{ m/s})(- \cos 45^\circ) = m_0 (0 \text{ m/s}) + m_x (0 \text{ m/s}) $$ (note that neither puck has momentum in the $x$-direction after the collision.) Similarly, for the $y$-direction, you would have $$ m_0 (0 \text{ m/s}) + m_x (5 \text{ m/s})(- \cos 45^\circ) = m_0 (-5 \text{ m/s}) + m_x (0 \text{ m/s}) $$ These equations can be solved for $m_0$ and $m_x$ (and, thankfully, are consistent with each other.)

Your error is pretty much the same in part b of your problem.