Plane waves in special relativity

Question

I don't understand how there can be plane waves that by definition are spread through all of space if nothing can travel faster than light. Wouldn't every wave have to spread over time with at most the speed of light? I could understand when they are only appearing in the mathematics of calculating waves, but isn't it common to talk about light or photons in terms of plane waves?

Answer 1

The other answers saying that true plane waves don't exist and are mathematical idealizations are perfectly true, but you can certainly have waves that are near enough to plane in reality to give rise to the "problem" you allude to.

This is where we meet a subtlety to the oft-cited, but somewhat mashed assertion that nothing can travel faster than light. The correct statement is no causal link from event $A$ to event $B$ can lie outside $A$'s future lightcone, often stated no signal can propagate faster than $c$. The mashed statement is categorically not true, as I think you've just found out.

As you've noted, if one places a screen nearly transversely to a plane wave, and, if the plane wave is, say a powerful femtosecond pulse, you'll see its narrow (that's why I choose "femtosecond pulse") intersection with the screen scoot across the screen at a very high speed - arbitrarily high if you choose the right angle. In particular, the intersection, a bright line, can be seen to sweep across the screen at greater than $c$. This situation does not gainsay the no-supraluminal-signalling postulate of special relativity because there is no causal link between points on the wavefront; they have a common causal antecedent, namely the light source (e.g. you could be looking at a spherical wave at a great distance from its effectively point source), but the causal links between the source and each point separately are lightlike rays through spacetime. No causal link in this picture is a supraluminal one. Indeed, an observer moving fast enough (but at less than speed $c$) along the screen will see the intersection scoot along the screen in the opposite direction. They can even choose a speed to see the whole screen light up at once. Here we see the relativity of simulteneity at play. The light on screen scenario with the observer-dependent motion of the light pulse is also a variation on the phenomenon of Stellar Aberration.

I say more about special relativity, causality and the postulated forbidding of faster-than-light signaling in my answers here and here.

Let's look at similar examples to try to cement these ideas.

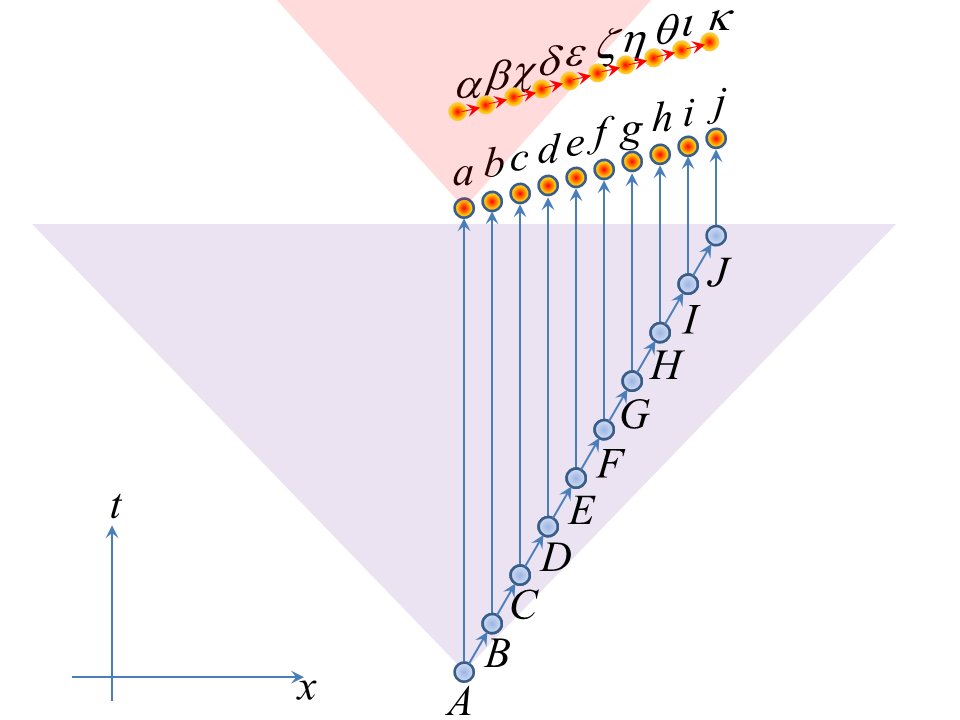
My figure above shows two versions of a "Football Wave" metachronal wave disturbance, both propagating at the same, greater than $c$ speed: the set of events $a,\,b,\,c,\,\cdots$ and $\alpha,\,\beta,\,\gamma,\,\cdots$. The former is perfectly in keeping with the principle of causality, the latter is not. In the former case, a preprogrammer visits positions on the $x$ axis one after the other, leaving instructions with protagonists at these positions to make a wave movement with their arms at a mutually agreed time in the future. These acts of "preprogramming" are the events $A,\,B,\,C,\,\cdots$. The mutually agreed times arrange for the motions of each protagonist's body (the events $a,\,b,\,c,\,\cdots$) in very swift succession to one another, begetting a metachronal wave pattern that travels at greater than speed $c$ from our frame. However, there are no direct causal links $a\rightarrow b,\,b\rightarrow c,\,\cdots$, so when a relatively uniformly moving observer sees the sequence $\cdots,\,c,\,b,\,a$ reversed in their time order, there is no contradiction: all the causal links in the whole graph $\{A,\,B,\,C,\,\cdots\}\cup\{a,\,b,\,c,\,\cdots\}$ lie within the future light cones of their forerunners and still do so even after any Lorentz transformation. However, the sequence of events $\alpha,\,\beta,\,\gamma,\,\cdots$ with putative direct causal links between them (i.e. the "whisper down the lane" signal propagation that governs most natural metachronal motion) violates causality together with Galileo's relativity postulate because some inertial observers will see these events happenning in backwards time order. Very like arguments show that the motion of a laser pointer spot across e.g. the surface of the Moon when the laser origin is on Earth, rotating in a plane at an angular speed of greater than about $45^\circ$ per second so that the spot sweeps across the Moon at greater than $300\,000{\rm km\,s^{-1}}$ is also in keeping with the principle of causality. Because the spot is moving at greater than $c$, it is seen to move in the opposite direction by some inertial observers, which fact is not a problem because there is no direct causal relationship between neighboring reflecting positions on the Moon, as for the events $a,\,b,\,c,\,\cdots$ in the figure. So, we can certainly see sequences of events (propagating "effects") in Nature travelling at greater than $c_I$, it's simply that such an observation rules out direct causal relationships between neighboring events in such a sequence. However, as we have seen with the Mexican Wave by Prior Arrangement, such a sequence does not rule out a causal relationships between the causal forerunners or antecedents of such a sequence. The causal antecedents $A,\,B,\,C,\,\cdots$ of $a,\,b,\,c,\,\cdots$ are causally related, even though there is no direct causal relationship between members of the latter sequence, which can therefore be seen evolving at a speed faster than $c_I$ without violating the causality principle. So, the observation of a sequence moving at the speed faster than the speed of light does not rule out all relationships between the sequence members, only direct causal ones. Any inertial observer, on observing a Mexican Wave moving faster than $c$, could make the null hypothesis that the motion is owing to statistically independent random jumps made by each of the Mexican Wavers. However, if there are $N$ wavers, then the likelihood of seeing them wave in order by chance alone is $2/(N!)$, so one is forced to reject the null hypothesis at pretty much any reasonable statistical significance for even a handful of wavers. The observation tells us that something is going on between them, even if it's not causal (as with any other noncausal statistical correlation).

Answer 2

Plane waves are fully compatible with special relativity, since they are Lorentz-invariant objects: $$\psi_k(x) = e^{i px/\hbar} = e^{i(Et-\vec{p}\cdot\vec{x})/\hbar}$$

You seem to be concerned with the fact that plane waves are spread through all space. But in fact, they're spread through all spacetime! They are perfectly periodic both in space and in time, so there's no starting or ending. The phase of the wave does propagate with the phase velocity, which is always equal or less than $c$.

Aside of the question of compatibility with special relativity, there's the question of the physical meaning of plane waves. Physical waves do have a beginning and an end, and they're produced by some finite source. Therefore, they have limited temporal extension and different spatial symmetry (usually, spherical symmetry), and they're not plane waves but wave packets. Nevertheless, any wave can be written as a sum of plane waves in a Lorentz-invariant way $$\psi(x) = \sum_k a_k \psi_k(x) = \sum_k a_k e^{ipx/\hbar}$$ and most of the waves can be approximated by plane waves far away from their source.

Answer 3

Plane waves are not real, they are just a mathematical device.

In quantum mechanics, particles are represented by wave packets, which do not have infinite amplitude and allow a collection of plane waves to group together by interfering constructively within a certain area and destructively outside that area.

From Wikipedia Wave Packets

In physics, a wave packet (or wave train) is a short "burst" or "envelope" of localized wave action that travels as a unit. A wave packet can be analyzed into, or can be synthesized from, an infinite set of component sinusoidal waves of different wavenumbers, with phases and amplitudes such that they interfere constructively only over a small region of space, and destructively elsewhere.Each component wave function, and hence the wave packet, are solutions of awave equation. Depending on the wave equation, the wave packet's profile may remain constant (no dispersion, see figure) or it may change (dispersion) while propagating.