1
$\begingroup$

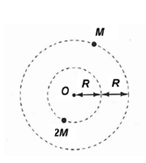
Two star of mass $M$ and $2M$, a distance $3x$ apart, rotate in circles about their common centre of mass $O$. Express the gravitational force acting on the stars in terms of $G,M$ and $x$.

enter image description here

I thought the question is quite simple, as I just apply the Newton's law of gravitation formula to calculate the required gravitational force, which is $$\frac{GM(2M)}{(3x)^2}= \frac{2GM^2}{9x^2}$$

But the answer given is $$\frac{GM^2}{2x^2}$$

Is the answer correct?

Improve this question
$\endgroup$
5
  • $\begingroup$ How can the force be constant? The stars do have diferent angular velocites right? $\endgroup$
    – Lelouch
    Commented Jul 15, 2016 at 2:38
  • 1
    $\begingroup$ @Lelouch Now the angular velocity is not important, the question is only the gravitational force between them. Btw, the stars have the same angular velocities here (in the frame of O), only their velocity vector differs. $\endgroup$
    – peterh
    Commented Jul 15, 2016 at 3:00
  • $\begingroup$ I'm voting to close this question as off-topic because it is merely questioning an incorrect answer in a textbook. The OP clearly understands the physics, and there is no conceptual difficulty here. $\endgroup$
    – sammy gerbil
    Commented Jul 15, 2016 at 13:58
  • $\begingroup$ $\uparrow$Given where? $\endgroup$
    – Qmechanic ♦
    Commented Jul 15, 2016 at 21:52
  • $\begingroup$ That was very obvious mistake , maybe this is the original question?jiskha.com/display.cgi?id=1469130729 $\endgroup$
    – user98038
    Commented Dec 4, 2016 at 18:05

1 Answer 1

Reset to default
4
$\begingroup$

The given answer is bad, and your version is correct. Newtons gravitational formula is very clear, and this is a trivial substitution in it, there isn't too many place of a mistake here.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.