How can it be possible that the presently observable universe is much smaller than particle horizon?

Question

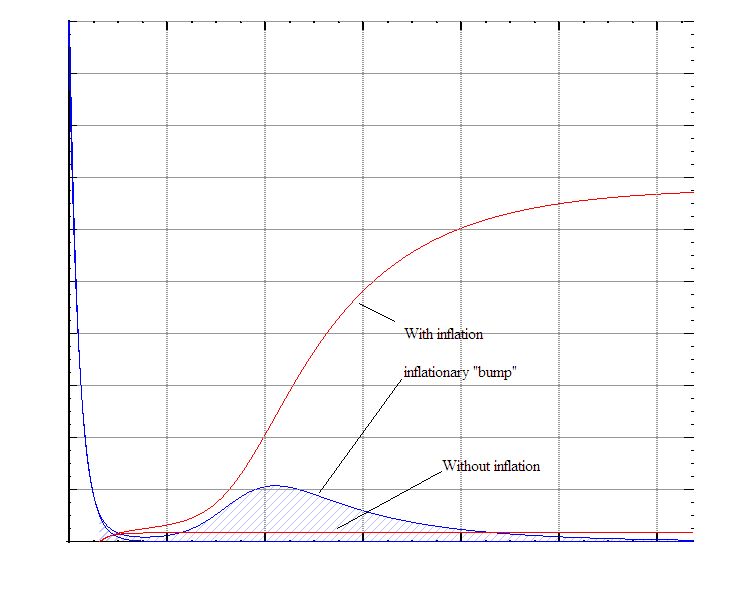
We know that to solve the homogeneity and isotropy problem, we invite the inflation and the inflation at early universe can make the presently observable universe much smaller than the particle horizon hence to avoid the homogeneity and isotropy problem.

But the particle horizon at any time by definition is the largest region where particles can possibly contact with us. Then how can it be possible that the region we now observed is much smaller the particle horizon?

Here is my answer, but if you have a different viewpoint, please argue against me.

(Now I think when we say that what we observed is much smaller than the particle horizon usually only means the observed particles that emitted at a particular time, e.g. at the CMB formation, are inside the particle horizon with respect to any of the observed particles at the emitted time. We shall note that the particle horizon depend on the observer. In the context of inflation, usually, the particle horizon corresponds to the one with respect to the photons at recombination. And we need that the photons we observed today are within the particle horizons of themselves at the time of recombination. Without inflation, this is not the case. Because the inflation will add up the weight of the conformal time at earlier history, hence make the photons at the CMB formation time inside the particle horizon with respect to the photons of that particular time. Equivalently, the inflation push forward the recombination to be more recent)

Answer 1

The particle horizon is at $$ \eta(t)~=~\int_0^t\frac{dt'}{a(t')}, $$ where for the de Sitter cosmology $a(t)~=~exp(t\sqrt{\Lambda/3})$. Here $\Lambda$ is the cosmological constant or parameter. The cosmological horizon is at $r_h~=~\sqrt{3/\Lambda}$. It is not hard to see that if I perform this integration that the particle horizon and the cosmological horizon are identical for large $t$.

The particle horizon differs from the cosmological horizon because of inflation. The vacuum density of the spacetime bubble at around $10^{-30}$ seconds becomes inflationary for about $10^{-35}$sec. Within chaotic infationary terms the vacuum bubble is on a high energy vacuum de Sitter spacetime and this detaches at around $10^{-30}$, and this high energy vacuum bubble can now inflate "like made" until the vacuum rolls into a low energy physical vacuum, that characterizing the vacuum now in this observable universe. This means that the cosmological constant $\Lambda~=~\Lambda(\phi,\dot\phi)$ for $\phi$ the inflaton field. This results in a bump in the above calculation of $\eta(t)$ which I have done numerically in the graph It is interesting what a difference this makes. Below is the graph for the straight cosmological constant, and the case where the cosmological constant changes from the inflaton field the graph is enormously different.

This is why the particle horizon is far larger than the cosmological horizon $r_h~\simeq~1.2\times 10^{10}$ light years. Beyond the cosmological horizon is a region where we can observe galaxies because we are actually witnessing them in the past when they were much closer. Any galaxy beyond the cosmological horizon today will never in our future be observed as it is today on the Hubble frame, Neither can we ever send a signal to such a galaxy. This is why with the Hubble relation $v~=~Hd$, that for $d~=~r_h$ we have $v~=~c$ and we observe these $z~=~1$ galaxies, and galaxies with $z~>~1$. The largest $z$ galaxies observed are around $z~=~8$. In addition the CMB is at $45$ billion light years distance with $z~=~1100$. The particle horizon is much more of a fundamental limit to observability. As one approaches the particle horizon the red shift factor $z~\rightarrow~\infty$ and any information reaching us is arbitrarily red shifted beyond observability.

Answer 2

That's because light outside the particle horizon has started travelling while it was still inside, but has yet to arrive. The particel horizon has retracted beyond the starting point since then, but the light was already underway and is still inside the particle horizon. This also means that the observable universe will diminish to become as big as the particle horizon once all the light that is currently travelling has arrived, but by then the particle horizon will have retracted even more and the "new" observable universe will still be bigger than the "new" particle horizon.

Example for the sake of clarity: some particles were created, 1 lightyear nerer to us than the particle horizon particle horizon, which was, let's say, 1000 light years away. Over the next 499 years the particle horizon has shrunk by 400 light years, now the emitted particles are still 500 light years away but the particle horizon is 400 light years nearer to us than the place the particles came from is. In 500 years those particles will hit us and we will see a point that is effectively beyond the current particle horizon (which will have shrunk even more by then)