I noticed that when I throw salt into a cooking pot and then mix, the salt collects in the center. As salt is denser than water, I would have expected it to go towards the border of the pot, and not in the middle. What is going on exactly there?
$\begingroup$
$\endgroup$
1
asked Jun 15, 2016 at 21:12
-
$\begingroup$ See also What forces are at work causing sand to migrate to the centre of a spinning bucket of water? $\endgroup$– John RennieCommented Jun 16, 2016 at 10:41
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Replace the salt with tea leaves, and you'll find the answer... with wikipedia.
The tea leaf paradox is exactly what you describe: denser than water particles accumulate in the vortex center.
This is an indirect effect of the pressure gradient cited by philip_0008. As you stir, the liquid accumulates at the periphery so that the extra height generates a radial pressure gradient that exactly balances the centrifugal momentum. As I explain in my answer to philip, this is however not sufficient to prevent particles from going outwards (and actually they transiently do so).
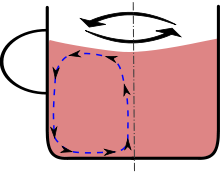
However, a secondary flow is generated because of the wall friction on the liquid, which reduces its outward motion close to the bottom wall, see the wikipedia sketch:
This secondary flow entrains the particles along the bottom wall, dominating over their centrifugal momentum, but cannot entrain them upwards in the center of the vortex against their weight, hence their accumulation in the center.
$\begingroup$
$\endgroup$
6
I've actually tried it when I was younger, intentionally stirring water circularly very fast in a large basin, such as to make a large 'vortex', and noticed that everything that I put in goes toward the center.
The reason seems to be that:
From wikipedia: "The fluid motion in a vortex creates a dynamic pressure (in addition to any hydrostatic pressure) that is lowest in the core region, closest to the axis, and increases as one moves away from it, in accordance with Bernoulli's Principle."
So that any object will have higher pressure at the side facing away from the center, than the side facing the center, thus there is a net pressure toward the center. Similar to how airplanes fly due to the pressure difference at the wings.
-
$\begingroup$ I don't think this can be the answer for particles denser than the liquid. Indeed, the pressure gradient compensates exactly the "centrifugal force" (to make short) in the liquid. If particles are denser, the pressure gradient in the fluid will necessarily be smaller than their own "centrifucal force". $\endgroup$– JoceCommented Jun 15, 2016 at 22:19
-
$\begingroup$ @Joce But remember that when you stir the water, it is the water that is moving fast first, and has developed enough rotation so that by the time the salt acquires enough speed, the water will wave enough pressure to counter the 'centrifugal force' of the salt grains. $\endgroup$ Commented Jun 15, 2016 at 22:25
-
$\begingroup$ @Joce This is partly the reason (in my opinion) why tornadoes can suck houses, which have densities greater than air. $\endgroup$ Commented Jun 15, 2016 at 22:30
-
$\begingroup$ At a given location and time, you can write the balance I was referring to and would find that the centrifugal force is larger. I have just found the correct answer to the question... in wikipedia, see my newer answer. $\endgroup$– JoceCommented Jun 15, 2016 at 22:30
-
$\begingroup$ @Joce The centrifugal force is larger only if you consider the fluid and the particle to have the same velocity, which is not always the case. $\endgroup$ Commented Jun 15, 2016 at 22:36
$\begingroup$
$\endgroup$
One candidate for a force that will oppose the particle's inertia is the so-called inertial lift that is created in the neighbourhood of the wall: grossly speaking, the flow created around the particle interacts with the wall and pushes the particle away from it.
You can see this paper: http://www.pnas.org/content/104/48/18892
...but it will not dominate at the scale and distance from the wall in your home-experiment, see my other newer answer!