Is it possible to synthesize an EMW which is not quantized?

Question

( I initially started to ask, "since according to Quantum-theory of light; the energy of a photon, depends only on the frequency of light-wave (E = h * nu), and no-mention of amplitude. So, does the energy of light doesn't depend upon amplitude?" But that question has already asked by >1 users , but I have 1 more question related to that )

Some of the other-answers suggests, the EMWs having same frequency but different amplitude, is a mixture-condition of photons.

Then, is-it really impossible to synthesize an electromagnetic wave of such an amplitude, so-that the energy is NOT an integral multiple of the basic photon's energy, using a dipole antenna and an oscillator ? like this? 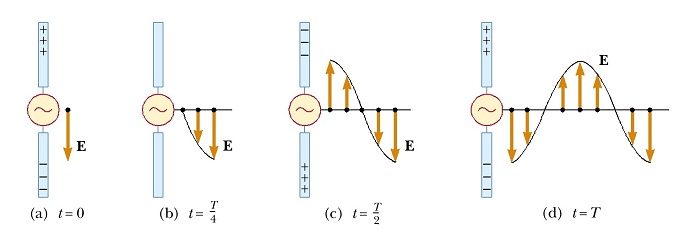Fig-1, Electromagnetic-waves could be synthesized by creating an oscillating electric field in a dipole antenna. (image from http://www.kshitij-iitjee.com/production-of-electromagnetic-waves-by-an-antenna)

I think, in this-way, all-kinds of frequencies and amplitudes could be synthesized (at-least theoretically). 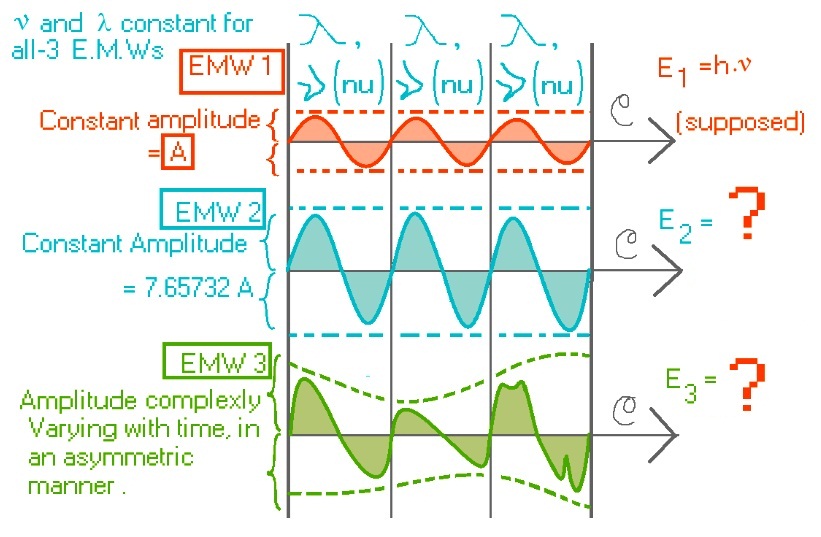Fig 2. EMWs with same frequency but varying amplitude. in Wave-2, I choose the value randomly, just to say, any-value could be placed arbitrarily. Neglect drawing-errors.

Then , what-thing restricting the antenna-system to produce an wave with such an amplitude?

Related question : Where is the amplitude of electromagnetic waves in the equation of energy of e/m waves? Where is the amplitude of electromagnetic waves in the equation of energy of e/m waves?

Answer 1

Possibly you are mixing different concepts together. A short answer is the following: indeed, for a single photon, the energy is proportional to its frequency. That is
\begin{align} E_{singlephoton}=h\nu. \end{align} However, for a wave package or an electromagnetic wave, the total energy is proportional to the photon number, $n_{photon}$, as well. That is \begin{align} E_{wave} = n_{photon}E_{singlephoton}=n_{photon}\cdot h\nu. \end{align} Therefore, the amplitude of an electromagnetic wave is proportional to both the photon number and frequency.

When you changing the envelope of the electromagnetic field, the power--which is the energy flow per unit time--is the vector product of the electrical fields and the magnetic fields integrated over the area you are interested in. That is $$P=\int \mathbf{E}\times \mathbf{B}\cdot d\mathbf{s},$$ where $\mathbf{E}$ and $\mathbf{B}$ are the electrical and magnetic field vectors respectively, and $d\mathbf{s}$ is the area element of the field. From the Maxwell equations, we know that \begin{align} \nabla\times \mathbf{E} &= -\frac{\partial\mathbf{B}}{\partial t}\\ \nabla\times \mathbf{H} &= \mathbf{J}+\frac{\partial\mathbf{D}}{\partial t} \end{align} with $\mathbf{D}$ the familiar displacement of the electrical field. Therefore, to generate the time-dependent and propagating electrical field as shown in your figures, you need to have a time-varying E-M field in some particular forms through the oscillation (changing spatial distribution of fields over time as shown in the Maxwell equations above) of the dipole field in the antenna. Roughly speaking, the oscillating frequency and the oscillation amplitude of the dipole of the antenna determine the energy and wave envelope of the propagating wave together. If the frequency is fixed, the larger the oscillation amplitude is, the more photons this antenna will generate in a unit time. You can change the oscillation frequency and amplitude of the dipole to generate arbitrary shapes of waves through some engineering techniques. A detailed analysis may take longer, but hopefully you get the main idea.

Since this is an exercise problem, I would let you to figure out the quantitative solution of the problem to check your understanding for yourself. For example, part (a) and (b) obviously have the same frequency but different amplitudes. You could use the ideas outlined above to solve the whole problem. Wish this helps.