If I let a stone drop from a certain height and throw a stone from a certain height, with air resistance, which will reach the ground first? When I draw a free body diagram the air resistance in the y axis for the throw is smaller in magnitude compared to the free fall, and yet it is the free fall that reaches the ground first. Why is that?
3 Answers
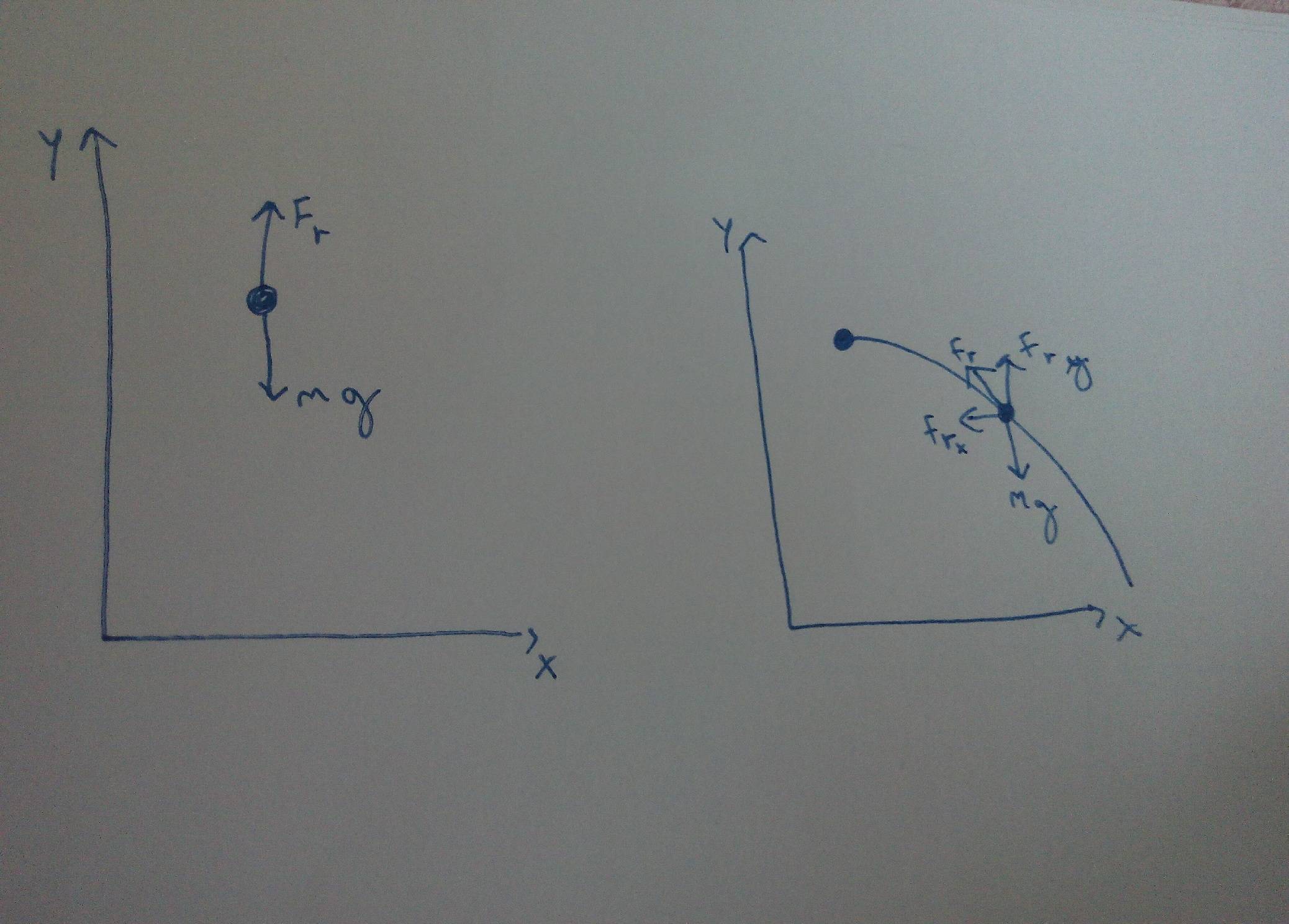
First remember that Air resistance is proportional to $v_{net}^2$.
And $v_{net}^{\text{freefall(1)}}<v_{net}^{\text{throw at angle $\theta$ to normal(2)}}$
So air-resistive force acting in free fall proportional to $(v_{net}^{(1)})^2$
And air-resistive force acting in the other case proportional to $(v_{net}^{(2)})^2\cos(\phi) $ ($\phi$ is the angle made at that moment with normal)
So it might well happen that if you plug in values of $g$and other friction constants and velocities,$(v_{net}^{(2)})^2\cos(\phi)> (v_{net}^{(1)})^2$ in which case freefalling body comes to the ground first.
-
$\begingroup$ @heather Thanks. I have been on stack exchange for some time and I think I know the basic rules. $\endgroup$– QwertyCommented Jun 27, 2016 at 3:33
"Throw a stone from a certain height" - Does not say what angle you throw the stone. To make it equivalent, I will assume, you throw the stone horizontally. Otherwise, the two cases are not equivalent for free fall.
Considering horizontal through, the two stones can take different time to reach the ground depending upon the shape of the stone.
The horizontal move through air can generate some uplift (like that of a plane), making it reach the ground slower.
However, in certain shapes, the horizontal component can be converted into vertically down speed due to the aerodynamics. Think reverse of plane uplift. This way the horizontally thrown stone can even reach the ground faster.
Just doing the exercise with one shape is not enough to give all different outcomes.
The two stones would reach ground at the same time, only if aerodynamics does not impact the vertical movement, which would be pretty difficult to achieve. May be a perfectly spherical stone thrown with no rotation.
To clarify, movement in the X-direction is separate from movement in the Y-direction. Regardless of the fact that one pebble is moving horizontally, both experience the same downwards force of -9.8 m/s^2. Therefore, if they were released from the same height at the same time, one moving straight down and one moving horizontally, they would both reach the ground at the same time.