How to relate heat build up in an open pipe to sound frequency and specific heat capacity of the pipe's material.

Question

I am designing a physics experiment with my clarinet. A temperature probe and a microphone graph changes in temperature and sound pressure, respectively. I am trying to demonstrate how different woods effect the performance of the clarinet through their specific heat capacities as the speed of sound is directly proportional to the temperature, and so changes pitch by some cents, damaging performance. I have one clarinet and the comparison will be made mathematically by taking the specific heat capacities of other woods. But, how should I relate heat build up in an open pipe to sound frequency and specific heat capacity of the pipe's material in order to demonstrate what wood is best for clarinet builds by taking these physical parameters?

Answer 1

When you blow air into the clarinet (or any wind instrument), several things happen:

• vibrations of the reed will cause pressure fluctuations in the air
• these pressure fluctuations travel at the speed of sound through the clarinet
• At openings in the side, and in particular at the opening at the end, some of the pressure waves will be reflected
• The reflected wave will affect the fluttering of the reed, and particular frequencies will be reinforced - this is how a tone is created

The things that affect the pitch are

• the speed of sound in the air
• the "effective length" of the column of air that resonates (I say "effective", because depending on the positions of the fingers, there will be multiple reflections that result in a specific frequency being selected - but there is no single dimension on the clarinet that corresponds to this length unless all holes were closed. And even then, the effective length is not exactly the same as the distance to the opening as there is some effect of the diameter)

The speed of sound depends on

• the density of the air
• the bulk modulus of the air
• the adiabatic index $\gamma=\frac{c_p}{c_v}$

The equation is

$$c=\sqrt{\frac{K}{\rho}}=\sqrt{\frac{\gamma P}{\rho}}$$

The ratio of pressure over density depends on

• the temperature of the air
• the relative humidity: the air you breathe out is saturated at 37°C, but as it cools down in the clarinet some will condense; the remaining humidity, and thus density, will depend in part on the temperature of the body of the clarinet
• the composition of the air: if you hold a note for a long time, the composition of the expired air will increase in $\rm{CO_2}$ which increases the density of the air

The temperature dependence you are talking about will have a couple of different effects; let's try to estimate their relative magnitude. Assume that the room temperature is 20°C, and that the temperature of the clarinet will increase by 2°C as you start playing. How much would that affect each of the terms discussed?

Length:

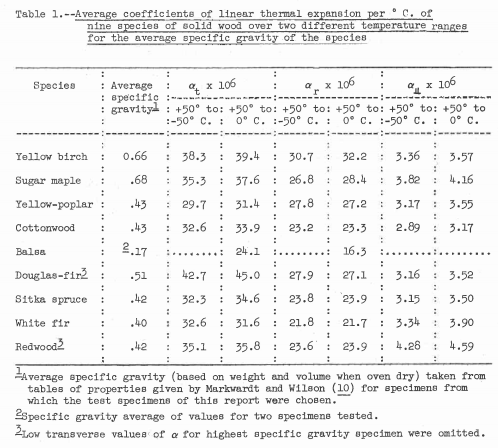
The coefficient of linear expansion of wood depends very strongly on the direction, being about 10x greater across the fiber than along it. I suspect that a clarinet is built with the wood along the length, in which case the coefficient of thermal expansion is about $3\cdot 10^{-6}~\rm{/K}$ source. The following table, from the same source, shows some of the variation between different types of wood:

We conclude that a 2°C change in temperature will change the length by 6 parts in a million - so a concert A (440 Hz) will change by 0.002 Hz. It is safe to say you would not be able to hear or measure such a small change. Note that cheap ABS instruments have a coefficient of thermal expansion that is easily 10x greater; over a wide range of temperatures they might have a small but noticeable shift in pitch.

Temperature

All things being equal, speed of sound varies with temperature because, at constant pressure, the density will increase as temperature drops. It is easy to see that this results in a square root relationship with temperature (not "directly proportional" as you stated in your question):

$$c\propto \sqrt{T}$$

A change of 2°C will change the pitch by $\frac{1}{293}% or about 1.5 Hz. That is clearly noticeable.

However, the temperature will also have a strong impact on the density of the air due to the saturation effect I described earlier. Data on saturated vapor pressure can be found on this page. I took some of the data and fitted a curve, then interpolated that curve over a smaller range. This gave the following values:

T(°C)  P(Pa)
16     1799
18     2052
20     2338
22     2655
24     3005
26     3386
28     3798
30     4243

This shows that the partial pressure of water vapor increases by 317 Pa from 20°C to 22°C. Since the total air pressure remains constant, the total number of molecules per unit volume remains the same; but since the water molecule is significantly lighter than either oxygen or nitrogen, the density of the air will decrease. I will use an average molecular weight for air of 29 (20% oxygen at 32, 80% nitrogen at 28 = 28.8), and a molecular weight for water of 18. 317 Pa partial pressure implies about 0.3% ($\frac{317}{10^5}$) of air was replaced with water vapor. This results in a fractional decrease in density of $0.3%\cdot\frac{28.8-18}{28.8}=0.11%$, an increase in speed of sound of 0.055%, and a decrease in pitch of 0.242 Hz.

Carbon dioxide

We can do a similar analysis for the exhaled carbon dioxide: according to "Quantitative Human Physiology: An Introduction" by Joseph Feher, the partial pressure of $\rm{CO_2}$ in expired air is typically 40 mm Hg - this obviously is going to depend a bit on how "fresh" the air in your lungs is, so the number will increase towards the end of a long breath. Using the same analysis as above, but replacing "mean air" with carbon dioxide, we find the effect of carbon dioxide (molecular mass = 44) quite large: an increase in density up to 2.8%, and therefore a decrease in pitch up to 1.4% or up to 6 Hz. Clearly, the concentration does not change by quite so much - but I believe it's a factor that needs to be controlled for a careful experiment.

Finally - the heating of the clarinet wall will be caused only by the expired air, not by sound pressure of the music. The power levels in sound, and the corresponding displacements, are surprisingly low, as I explored in an earlier answer. Further, since sound in the audible range propagates mostly adiabatically, it simply doesn't have time for any heat to be transferred (during one half cycle it increases in temperature, and during the other half it decreases; these changes happen so quickly that no significant heat transfer takes place. This was described in this earlier question / answer

Here are some graphs showing various relationships between temperature, pressure, humidity, and speed of sound - taken from http://www.phy.mtu.edu/~suits/SpeedofSound.html :