3
$\begingroup$

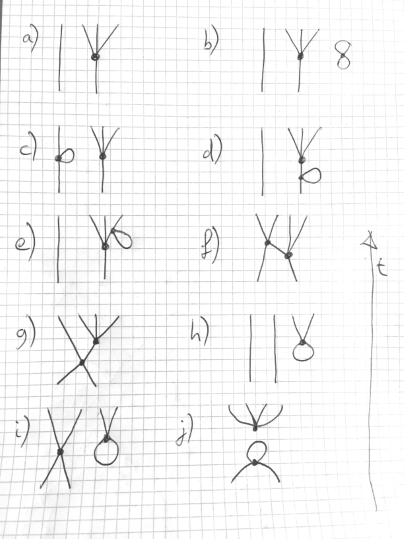
In exercise I.7.3 of A. Zee's QFT in a Nutshell, we have to draw all the Feynman diagrams of the scalar theory

$$ Z(J) = \int D\varphi e^{i\int d^4x\{\frac 12[(\partial\varphi)^2-m^2\varphi^2]-(\lambda/4!)\varphi^4+J\varphi\}} $$

describing two mesons producing four mesons up to and including order $\lambda^2$.

I came up with ten different diagrams a)-j) so far:

enter image description here

I think that only diagrams a)-g) are correct, since in h)-j) there seems to be a violation of conservation of energy: There are vacuum bubbles that "come to life"; particles are created "out of nothing".

However, I cannot find any rules in the book that tell me that these three diagrams are invalid.

  • Am I correct that only a)-g) are correct Feynman diagrams for the problem at hand?
  • What exact rules are there that tell me which Feynman diagrams are valid and which are not?
Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Are you sure Zee didn't want you to draw the connected diagrams for this? Because one usually draws the connected one when takling about diagrams for a process. $\endgroup$
    – ACuriousMind ♦
    Commented Nov 3, 2015 at 15:46
  • $\begingroup$ @ACuriousMind That might well be. Then the correct answer would be f) and g), is that correct? However, my question still stands. Are the diagrams h)-j) correct, and if not, which rules tell us they aren't? $\endgroup$
    – Bass
    Commented Nov 3, 2015 at 15:50
  • 1
    $\begingroup$ Yes, f) and g). I'm not sure what exactly you mean by "correct", though. They are admissible disconnected Feynman diagrams. They just aren't what you draw and compute to get the amplitude for this process. $\endgroup$
    – ACuriousMind ♦
    Commented Nov 3, 2015 at 15:53

1 Answer 1

Reset to default
2
$\begingroup$

As explained by @ACuriousMind, even the diagrams with vacuum bubbles that "come to life" are correct Feynman diagrams. However, when one translates the diagrams to actual amplitude terms, these terms don't contribute anything because of the delta function one adds at each vertex for energy-momentum conservation.

The combined energy-momentum of the virtual particles coming from the loop is zero, so there is no way a particle pair with real momentum is coming from that interaction.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.