How is normal force distributed along the surface of contact?

Question

Help me settle this argument.

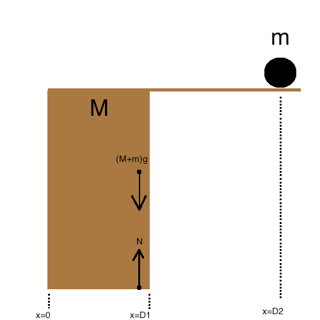
A mass $m$ is placed on a thin diving board. The base of the diving board has mass $M>>m$. Does the board tip over?

I drew the following FBD and concluded there is no net torque.

My friend thinks the normal force $N$ will be applied at a different $x$-coordinate, perhaps $x=D_1/2$. This would result in a net torque. How can I prove him wrong using the laws of classical mechanics?

---

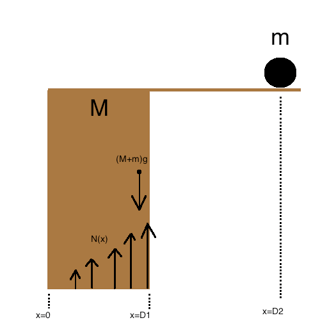
Of course, the normal force is not applied at a single point. It is distributed across the entire surface of contact. Is it possible to compute the force distribution $N(x)$? We could measure this experimentally by placing many small scales under the base.

Answer 1

I think that you're making this problem more complicated than it has to be in order to simply determine if the assembly will tip over or not. You don't really need the spatial distribution of the forces being exerted by the table or ground on the assembly. All you need to note is that if the pivot point is at x=D1 then the ground will exert whatever counter-torque is needed in order to prevent the assembly from rotating counter-clockwise about the pivot point.

So all you need to do is to calculate the torques contributed by mass m and mass M about the pivot point. If the sum of those two torques acts in the counter-clockwise direction, then the ground will exert a counter-torque of the same magnitude but in the opposite direction to prevent the whole assembly from turning counter-clockwise. On the other hand, if the sum of the two torques from m and M acts in the clockwise direction, the ground plays no role in providing a counter-torque and the whole assembly tips over in the clockwise direction around the pivot point.

Calculating the total torque due to m and M should not be difficult. For the purposes of calculating the torque due to M, you can assume just a single force of magnitude Mg (where g is gravitational acceleration) acting downward at its center-of-mass.

Answer 2

If there is no torque, then $$\sum \tau = \mathbf{r_1}\times\mathbf{F_M}+\mathbf{r_2}\times\mathbf{F_m}=0$$ Therefore, $$\mathbf{r_1}\times M\mathbf{g}+\mathbf{r_2}\times m\mathbf{g}=0\tag{1}$$ where $\mathbf{r_i}$ denotes the position of the center of mass of the combined system relative to the force applied. If we give the box dimensions $h$ and $l$, the ball a radius $R$, and say that the ramp extends a distance $d$ from $x=0$, then $$\mathbf{x_{cm}}=\frac{\frac{1}{2}\mathbf{l}M+\left(\frac{1}{2}\mathbf{l}+\mathbf{d}-\frac{1}{2}\mathbf{R}\right)m}{M+m}$$ and $$\mathbf{y_{cm}}=\frac{\frac{1}{2}\mathbf{h}m+\left(\mathbf{h+\frac{1}{2}R}\right)M}{M+m}$$ ($x_{cm}$,$y_{cm}$) are the coordinates of the center of mass. You can then compute $\mathbf{r_1}$ and $\mathbf{r_2}$. If $(1)$ holds, then you are correct; if not, your friend is correct.

Answer 3

Torque? Why do you think you need to think about torque?

Is the center of mass over the base of support? It is if $0 < M D_1/2 + m D_2 < D_1$. I.e., if $\frac{-M D_1}{2 D_2}< m < \frac{D_1}{D_2} (1-M/2)$.