Understanding the Bloch sphere

Question

It is usually said that the points on the surface of the Bloch sphere represent the pure states of a single 2-level quantum system. A pure state being of the form: $$ |\psi\rangle = a |0\rangle+b |1\rangle $$ And typically the north and south poles of this sphere correspond to the $|0\rangle$ and $|1\rangle$ states. Image: ("Bloch Sphere" by Glosser.ca - Own work. Licensed under CC BY-SA 3.0 via Commons - https://commons.wikimedia.org/wiki/File:Bloch_Sphere.svg#/media/File:Bloch_Sphere.svg)

1. But isn't this very confusing? If the north and south poles are chosen, then both states are on the same line and not orthogonal anymore, so how can one choose an arbitrary point $p$ on the surface of the sphere and possibly decompose it in terms of $0,1$ states in order to find $a$ and $b$? Does this mean that one shouldn't regard the Bloch sphere as a valid basis for our system and that it's just a visualization aid?

2. I have seen decompositions in terms of the internal angles of the sphere, in the form of: $a=\cos{\theta/2}$ and $b=e^{i\phi}\sin{\theta/2}$ with $\theta$ the polar angle and $\phi$ the azimuthal angle. But I am clueless as to how these are obtained when $0,1$ states are on the same line.

Answer 1

The Bloch sphere is beautifully minimalist.

Conventionally, a qubit has four real parameters; $$|\psi\rangle=a e^{i\chi} |0\rangle + b e^{i\varphi} |1\rangle.$$ However, some quick insight reveals that the a-vs-b tradeoff only has one degree of freedom due to the normalization a² + b² = 1, and some more careful insight reveals that, in the way we construct expectation values in QM, you cannot observe χ or φ themselves but only the difference χ – φ, which is 2π-periodic. (This is covered further in the comments below but briefly: QM only predicts averages $\langle \psi|\hat A|\psi\rangle$ and shifting the overall phase of a wave function by some $|\psi\rangle\mapsto e^{i\theta}|\psi\rangle$ therefore cancels itself out in every prediction.)

So if you think at the most abstract about what you need, you just draw a line from 0 to 1 representing the a-vs-b tradeoff: how much is this in one of these two states? Then you draw circles around it: how much is the phase difference? What stops it from being a cylinder is that the phase difference ceases to matter when a = 1 or b = 1, hence the circles must shrink down to points. And voila, you have something which is topologically equivalent to a sphere. The sphere contains all of the information you need for experiments, and nothing else.

It’s also physical, a real sphere in 3D space.

This is the more shocking fact. Given only the simple picture above, you could be forgiven for thinking that this was all harmless mathematics: no! In fact the quintessential qubit is a spin-½ system, with the Pauli matrices indicating the way that the system is spinning around the x, y, or z axes. This is a system where we identify $$|0\rangle\leftrightarrow|\uparrow\rangle, \\ |1\rangle\leftrightarrow|\downarrow\rangle,$$ and the phase difference comes in by choosing the +x-axis via $$|{+x}\rangle = \sqrt{\frac 12} |0\rangle + \sqrt{\frac 12} |1\rangle.$$

The orthogonal directions of space are not Hilbert-orthogonal in the QM treatment, because that’s just not how the physics of this system works. Hilbert-orthogonal states are incommensurate: if you’re in this state, you’re definitely not in that one. But this system has a spin with a definite total magnitude of $\sqrt{\langle L^2 \rangle} = \sqrt{3/4} \hbar$, but only $\hbar/2$ of it points in the direction that it is “most pointed along,” meaning that it must be distributed on some sort of “ring” around that direction. Accordingly, when you measure that it’s in the +z-direction it turns out that it’s also sort-of half in the +x, half in the –x direction. (Here “sort-of” means: it is, if you follow up with an x-measurement, which will “collapse” the system to point → or ← with angular momentum $\hbar/2$ and then it will be in the corresponding “rings” around the x-axis.)

Spherical coordinates from complex numbers

So let’s ask “which direction is the general spin-½ $|\psi\rangle$ above, most spinning in?” This requires constructing an observable.

To give an example observable, if the +z-direction is most-spun-in by a state $|\uparrow\rangle$ then the observable for $z$-spin is the Pauli matrix $$\sigma_z = |\uparrow\rangle\langle\uparrow| - |\downarrow\rangle\langle\downarrow|=\begin{bmatrix}1&0\\0&-1\end{bmatrix},$$which is +1 in the state it's in, -1 in the Hilbert-perpendicular state $\langle \downarrow | \uparrow \rangle = 0.$

Similarly if you look at $$\sigma_x = |\uparrow\rangle \langle \downarrow | + |\downarrow \rangle\langle \uparrow |=\begin{bmatrix}0&1\\1&0\end{bmatrix},$$ you will see that the $|{+x}\rangle$ state defined above is an eigenvector with eigenvalue +1 and similarly there should be a $|{-x}\rangle \propto |\uparrow\rangle - |\downarrow\rangle$ satisfying $\langle {+x}|{-x}\rangle = 0,$ and you can recover $\sigma_x = |{+x}\rangle\langle{+x}| - |{-x}\rangle\langle{-x}|.$

So, let’s now do it generally. The state orthogonal to $|\psi\rangle = \alpha |0\rangle + \beta |1\rangle$ is not too hard to calculate as $|\bar \psi\rangle = \beta^*|0\rangle - \alpha^* |1\rangle,$ so the observable which is +1 in that state or -1 in the opposite state is:$$ \begin{align} |\psi\rangle\langle\psi| - |\bar\psi\rangle\langle\bar\psi| &= \begin{bmatrix}\alpha\\\beta\end{bmatrix}\begin{bmatrix}\alpha^*&\beta^*\end{bmatrix} - \begin{bmatrix}\beta^*\\-\alpha^*\end{bmatrix} \begin{bmatrix}\beta & -\alpha\end{bmatrix}\\ &=\begin{bmatrix}|\alpha|^2 - |\beta|^2 & 2 \alpha\beta^*\\ 2\alpha^*\beta & |\beta|^2 - |\alpha|^2\end{bmatrix} \end{align}$$Writing this as $v_i \sigma_i$ where the $\sigma_i$ are the Pauli matrices we get:$$v_z = |\alpha|^2 - |\beta|^2,\\ v_x + i v_y = 2 \alpha^* \beta.$$ Now here's the magic, let's allow the Bloch prescription of writing $$\alpha=\cos\left(\frac\theta2\right),~~\beta=\sin\left(\frac\theta2\right)e^{i\varphi},$$ we find out that these are:$$\begin{align} v_z &= \cos^2(\theta/2) - \sin^2(\theta/2) &=&~ \cos \theta,\\ v_x &= 2 \cos(\theta/2)\sin(\theta/2) ~\cos(\phi) &=&~ \sin \theta~\cos\phi, \\ v_y &= 2 \cos(\theta/2)\sin(\theta/2) ~\sin(\phi) &=&~ \sin \theta~\sin\phi. \end{align}$$So the Bloch prescription uses a $(\theta, \phi)$ which are simply the spherical coordinates of the point on the sphere which such a $|\psi\rangle$ is “most spinning in the direction of.”

So instead of being a purely theoretical visualization, we can say that the spin-½ system, the prototypical qubit, actually spins in the direction given by the Bloch sphere coordinates! (At least, insofar as a spin-up system spins up.) It is ruthlessly physical: you want to wave it away into a mathematical corner and it says, “no, for real systems I’m pointed in this direction in real 3D space and you have to pay attention to me.”

How these answer your questions.

1. Yes, N and S are spatially parallel but in the Hilbert space they are orthogonal. This Hilbert-orthogonality means that a system cannot be both spin-up and spin-down. Conversely the lack of Hilbert-orthogonality between, say, the z and x directions means that when you measure the z-spin you can still have nonzero measurements of the spin in the x-direction, which is a key feature of such systems. It is indeed a little confusing to have two different notions of “orthogonal,” one for physical space and one for the Hilbert space, but it comes from having two different spaces that you’re looking at.

2. One way to see why the angles are physically very useful is given above. But as mentioned in the first section, you can also view it as a purely mathematical exercise of trying to describe the configuration space with a sphere: then you naturally have the polar angle as the phase difference, which is $2\pi$-periodic, so that is a naturally ‘azimuthal’ coordinate; therefore the way that the coordinate lies along 0/1 should be a ‘polar’ coordinate with 0 mapping to $|0\rangle$ and π mapping to $|1\rangle$. The obvious way to do this is with $\cos(\theta/2)$ mapping from 1 to 0 along this range, as the amplitude for the $|0\rangle$ state; the fact that $\cos^2 + \sin^2 = 1$ means that the $|1\rangle$ state must pick up a $\sin(\theta/2)$ amplitude to match it.

Answer 2

A. Two-state systems

Let a two-state system, the states being independent of the space-time coordinates. In this case the system has a new degree of freedom. A classical example is a particle with spin angular momentum $\:\frac12 \hbar\:$.

Let to the two states there correspond the basic states \begin{equation} \vert u\rangle= \begin{bmatrix} 1\\ 0 \end{bmatrix} \equiv \text{up state} \,, \quad \vert d\rangle= \begin{bmatrix} 0\\ 1 \end{bmatrix} \equiv \text{down state} \tag{01}\label{01} \end{equation} named up and down state respectively.

A system state is expressed by the state vector \begin{equation} \vert\psi\rangle = \xi\vert u\rangle \boldsymbol{+} \eta\vert d\rangle \quad \text{where} \:\:\:\xi,\eta \in \mathbb{C}\quad \text{and}\:\:\: \vert\xi\vert^{2} \boldsymbol{+}\vert\eta\vert^{2} =1 \tag{02}\label{02} \end{equation} The complex numbers $\:\xi,\eta\:$ are the probability amplitudes and the non-negative reals $\:\vert\xi\vert^{2},\vert\eta\vert^{2}\:$ the probabilities to be the system in state $\:\vert u\rangle,\vert d\rangle\:$ respectively.

The Hilbert space of the system states is in many respects identical to (the unit sphere of) the complex space $\:\mathbb{C}^{2}$.

An observable of the system would be represented by a $\:2\times2\:$ hermitian matrix A of the form \begin{equation} A= \begin{bmatrix} a_3 & a_1\!\boldsymbol{-}\!ia_2 \vphantom{\dfrac{a}{b}}\\ a_1\!\boldsymbol{+}\!ia_2 & a_4\vphantom{\dfrac{a}{b}} \end{bmatrix} \quad \text{with} \:\:\:\left(a_1,a_2,a_3,a_4\right) \in \mathbb{R}^{4} \tag{03}\label{03} \end{equation} so the linear space of the $\:2\times2\:$ hermitian matrices is in many respects identical to $\:\mathbb{R}^{4}$. From the usual basis of $\:\mathbb{R}^{4}\:$ we construct a basis for this space of matrices \begin{equation} E_1= \begin{bmatrix} 0 & \!\!\hphantom{\boldsymbol{-}}1 \vphantom{\tfrac{a}{b}}\\ 1 & \!\!\hphantom{\boldsymbol{-}}0\vphantom{\tfrac{a}{b}} \end{bmatrix} \quad , \:\:\: E_2= \begin{bmatrix} 0 & \!\!\boldsymbol{-} i \vphantom{\tfrac{a}{b}}\\ i & \!\!\hphantom{\boldsymbol{-}} 0\vphantom{\tfrac{a}{b}} \end{bmatrix} \quad , \:\:\: E_3= \begin{bmatrix} 1 & \!\!\hphantom{\boldsymbol{-}} 0 \vphantom{\frac{a}{b}}\\ 0 & \!\!\hphantom{\boldsymbol{-}} 0\vphantom{\frac{a}{b}} \end{bmatrix} \quad , \:\:\: E_4= \begin{bmatrix} 0 & \!\!\hphantom{\boldsymbol{-}}0 \vphantom{\tfrac{a}{b}}\\ 0 & \!\!\hphantom{\boldsymbol{-}}1 \vphantom{\tfrac{a}{b}} \end{bmatrix} \tag{04}\label{04} \end{equation}

Now, if the basic states $\:\vert u\rangle,\vert d\rangle\:$ of equation \eqref{01} correspond to the eigenstates of eigenvalues $\:\boldsymbol{+}1,\boldsymbol{-}1\:$ respectively of an observable then this observable would be represented by the matrix
\begin{equation} \begin{bmatrix} 1 & \!\!\hphantom{\boldsymbol{-}} 0 \vphantom{\frac{a}{b}}\\ 0 & \!\!\boldsymbol{-} 1\vphantom{\frac{a}{b}} \end{bmatrix} \tag{05}\label{05} \end{equation} not included in \eqref{04}. But instead of the basis \eqref{04} we could make use of the following linear combinations of them \begin{align} E'_1 \!=\!E_1\!=\!& \begin{bmatrix} 0 & \!\!\hphantom{\boldsymbol{-}}1 \vphantom{\tfrac{a}{b}}\\ 1 & \!\!\hphantom{\boldsymbol{-}}0\vphantom{\tfrac{a}{b}} \end{bmatrix} \qquad\qquad\quad \,E'_2 \!=\!E_2\!=\! \begin{bmatrix} 0 & \!\!\boldsymbol{-} i \vphantom{\tfrac{a}{b}}\\ i & \!\!\hphantom{\boldsymbol{-}} 0\vphantom{\tfrac{a}{b}} \end{bmatrix} \nonumber\\ E'_3\!=\!\left(E_3\!-\!E_4\right)\!=\!& \begin{bmatrix} 1 & \!\!\hphantom{\boldsymbol{-}} 0 \vphantom{\frac{a}{b}}\\ 0 & \!\!\boldsymbol{-} 1\vphantom{\frac{a}{b}} \end{bmatrix} \qquad E'_4 \!=\!\left(E_3+E_4\right)\!=\! \begin{bmatrix} 1 & \!\!\hphantom{\boldsymbol{-}}0 \vphantom{\tfrac{a}{b}}\\ 0 & \!\!\hphantom{\boldsymbol{-}}1 \vphantom{\tfrac{a}{b}} \end{bmatrix} \tag{06}\label{06} \end{align} and changing symbols and arrangement

\begin{equation} I= \begin{bmatrix} 1 & \!\!\hphantom{\boldsymbol{-}}0 \vphantom{\tfrac{a}{b}}\\ 0 & \!\!\hphantom{\boldsymbol{-}}1 \vphantom{\tfrac{a}{b}} \end{bmatrix} \quad , \:\:\: \sigma_1= \begin{bmatrix} 0 & \!\!\hphantom{\boldsymbol{-}}1 \vphantom{\tfrac{a}{b}}\\ 1 & \!\!\hphantom{\boldsymbol{-}}0\vphantom{\tfrac{a}{b}} \end{bmatrix} \quad , \:\:\: \sigma_2= \begin{bmatrix} 0 & \!\!\boldsymbol{-} i \vphantom{\tfrac{a}{b}}\\ i & \!\!\hphantom{\boldsymbol{-}} 0\vphantom{\tfrac{a}{b}} \end{bmatrix} \quad , \:\:\: \sigma_3= \begin{bmatrix} 1 & \!\!\hphantom{\boldsymbol{-}} 0 \vphantom{\frac{a}{b}}\\ 0 & \!\!\boldsymbol{-} 1\vphantom{\frac{a}{b}} \end{bmatrix} \tag{07}\label{07} \end{equation} where $\:\boldsymbol{\sigma}=\left(\sigma_1,\sigma_2,\sigma_3\right)\:$ the Pauli matrices.

Now, the basic states $\:\vert u\rangle,\vert d\rangle\:$ of equation \eqref{01} are eigenstates of $\:\sigma_3\:$ so it's necessary to be expressed with the subscript $\:'3'\:$ \begin{equation} \vert u_3\rangle= \begin{bmatrix} \:\:1\:\:\vphantom{\dfrac{a}{b}}\\ \:\:0\:\:\vphantom{\dfrac{a}{b}} \end{bmatrix} \,, \quad \vert d_3\rangle= \begin{bmatrix} \:\:0\:\:\vphantom{\dfrac{a}{b}}\\ \:\:1\:\:\vphantom{\dfrac{a}{b}} \end{bmatrix} \tag{08}\label{08} \end{equation} This must be done for the probability amplitudes $\:\xi,\eta\:$ also \begin{equation} \vert\psi\rangle = \xi_3\vert u_3\rangle \boldsymbol{+} \eta_3\vert d_3\rangle \quad \text{where} \:\:\:\xi_3,\eta_3\in \mathbb{C}\quad \text{and}\:\:\: \vert\xi_3\vert^{2} \boldsymbol{+}\vert\eta_3\vert^{2} =1 \tag{09}\label{09} \end{equation} The reason for this is that we can use as basic states of the Hilbert space equally well the eigenstates $\:\vert u_1\rangle,\vert d_1\rangle\:$ of eigenvalues $\:\boldsymbol{+}1,\boldsymbol{-}1\:$ respectively of $\:\sigma_1\:$ \begin{equation} \vert u_1\rangle=\frac{\sqrt{2}}{2} \begin{bmatrix} \:\:1\:\:\vphantom{\dfrac{a}{b}}\\ \:\:1\:\:\vphantom{\dfrac{a}{b}} \end{bmatrix}=\frac{\sqrt{2}}{2}\left(\vert u_3\rangle \boldsymbol{+}\vert d_3\rangle\right) \,, \quad \vert d_1\rangle=\frac{\sqrt{2}}{2} \begin{bmatrix} \:\:1\:\vphantom{\dfrac{a}{b}}\\ -1\:\,\vphantom{\dfrac{a}{b}} \end{bmatrix}=\frac{\sqrt{2}}{2}\left(\vert u_3\rangle \boldsymbol{-}\vert d_3\rangle\right) \tag{10}\label{10} \end{equation} so that \begin{equation} \vert\psi\rangle = \xi_1\vert u_1\rangle \boldsymbol{+} \eta_1\vert d_1\rangle \quad \text{where} \:\:\:\xi_1,\eta_1\in \mathbb{C}\quad \text{and}\:\:\: \vert\xi_1\vert^{2} \boldsymbol{+}\vert\eta_1\vert^{2} =1 \tag{11}\label{11} \end{equation} or the relevant to $\:\sigma_2\:$ \begin{equation} \vert u_2\rangle=\frac{\sqrt{2}}{2} \begin{bmatrix} \:\:1\:\:\vphantom{\dfrac{a}{b}}\\ \:\:i\:\:\vphantom{\dfrac{a}{b}} \end{bmatrix}=\frac{\sqrt{2}}{2}\left(\vert u_3\rangle \boldsymbol{+}i\vert d_3\rangle\right) \,, \quad \vert d_2\rangle=\frac{\sqrt{2}}{2} \begin{bmatrix} \:\:1\:\vphantom{\dfrac{a}{b}}\\ -i\:\,\vphantom{\dfrac{a}{b}} \end{bmatrix}=\frac{\sqrt{2}}{2}\left(\vert u_3\rangle \boldsymbol{-}i\vert d_3\rangle\right) \tag{12}\label{12} \end{equation} so that \begin{equation} \vert\psi\rangle = \xi_2\vert u_2\rangle \boldsymbol{+} \eta_2\vert d_2\rangle \quad \text{where} \:\:\:\xi_2,\eta_2\in \mathbb{C}\quad \text{and}\:\:\: \vert\xi_2\vert^{2} \boldsymbol{+}\vert\eta_2\vert^{2} =1 \tag{13}\label{13} \end{equation} The eigenstates $\vert u_1\rangle,\vert d_1\rangle,\vert u_2\rangle,\vert d_2\rangle$ are shown schematically in Figure-04.

Now, \begin{align} \xi_1 & =\tfrac{\sqrt{2}}{2}\left(\xi_3\boldsymbol{+}\eta_3\right) \tag{14a}\label{14a}\\ \eta_1 & =\tfrac{\sqrt{2}}{2}\left(\xi_3\boldsymbol{-}\eta_3\right) \tag{14b}\label{14b} \end{align} so \begin{align} \vert\xi_1\vert ^{2} & =\frac12\boldsymbol{+}\mathrm{Re}\left(\xi_3\eta^{\boldsymbol{*}}_3\right) \tag{15a}\label{15a}\\ \vert \eta_1\vert^{2} & =\frac12\boldsymbol{-}\mathrm{Re}\left(\xi_3\eta^{\boldsymbol{*}}_3\right) \tag{15b}\label{15b} \end{align} Also \begin{align} \xi_2 & =\tfrac{\sqrt{2}}{2}\left(\xi_3\boldsymbol{-}i\eta_3\right) \tag{16a}\label{16a}\\ \eta_2 & =\tfrac{\sqrt{2}}{2}\left(\xi_3\boldsymbol{+}i\eta_3\right) \tag{16b}\label{16b} \end{align} so \begin{align} \vert\xi_2\vert ^{2} & =\frac12\boldsymbol{-}\mathrm{Im}\left(\xi_3\eta^{\boldsymbol{*}}_3\right) \tag{17a}\label{17a}\\ \vert \eta_2\vert^{2} & =\frac12\boldsymbol{+}\mathrm{Im}\left(\xi_3\eta^{\boldsymbol{*}}_3\right) \tag{17b}\label{17b} \end{align} In equations \eqref{15a},\eqref{15b},\eqref{17a},\eqref{17b} by $\:z^{\boldsymbol{*}}\:$ we denote the complex conjugate of the complex number $\:z\:$ and by $\:\mathrm{Re}\left(z\right),\mathrm{Im}\left(z\right)\:$ the real and imaginary parts of $\:z$.

Since $\:\vert\xi_3\vert^{2} \boldsymbol{+}\vert\eta_3\vert^{2} =1\:$ we set (see Figure-01) \begin{align} \xi_3 & =\cos\omega_3\cdot e^{i\alpha_3} \:\:,\qquad 0\le\omega_3\le\frac{\pi}{2} \tag{18a}\label{18a}\\ \eta_3 & =\sin\omega_3\cdot e^{i\beta_3} \tag{18b}\label{18b}\\ \theta_3 & =2\omega_3=\text{polar angle with respect to $x_3-$axis}\:\:,\qquad 0\le\theta_3\le \pi \tag{18c}\label{18c} \end{align} so \begin{align} \xi_3\eta^{\boldsymbol{*}}_3 & =\cos\omega_3\cdot e^{i\alpha_3}\sin\omega_3\cdot e^{\boldsymbol{-}i\beta_3}=\cos\left(\dfrac{\theta_3}{2}\right)\cdot\sin\left(\dfrac{\theta_3}{2}\right)\cdot e^{\boldsymbol{-}i\left(\beta_3 \boldsymbol{-}\alpha_3\right)} =\dfrac{1}{2}\sin\theta_3\cdot e^{ \boldsymbol{-}i\phi_3} \tag{19a}\label{19a}\\ \phi_3 & = \beta_3\boldsymbol{-}\alpha_3 =\text{azimuthal angle with respect to $x_3-$axis}\:\:,\qquad 0\le\phi_3\le 2\pi \tag{19b}\label{19b} \end{align} Under these definitions \begin{align} \mathrm{Re}\left(\xi_3\eta^{\boldsymbol{*}}_3\right) & =\mathrm{Re}\left(\dfrac{1}{2}\sin\theta_3\cdot e^{ \boldsymbol{-}i\phi_3}\right)=\dfrac{1}{2}\sin\theta_3\cos\phi_3=\rho_3\cos\phi_3 \tag{20a}\label{20a}\\ \mathrm{Im}\left(\xi_3\eta^{\boldsymbol{*}}_3\right) & =\mathrm{Im}\left(\dfrac{1}{2}\sin\theta_3\cdot e^{ \boldsymbol{-}i\phi_3}\right)=\boldsymbol{-}\dfrac{1}{2}\sin\theta_3\sin\phi_3=\boldsymbol{-}\rho_3\sin\phi_3 \tag{20b}\label{20b}\\ \rho_3 & =\vert\xi_3\vert \cdot\vert\eta_3\vert =\cos\omega_3\sin\omega_3 =\dfrac{1}{2}\sin\theta_3 \tag{20c}\label{20c} \end{align} and equations \eqref{15a},\eqref{15b},\eqref{17a},\eqref{17b} yield the following probabilities \begin{align} \vert\xi_1\vert ^{2} & =\frac12\boldsymbol{+}\mathrm{Re}\left(\xi_3\eta^{\boldsymbol{*}}_3\right)=\frac12\boldsymbol{+}\rho_3\cos\phi_3=\frac12\left(1\boldsymbol{+}\sin\theta_3\cos\phi_3\right) \tag{21a}\label{21a}\\ \vert \eta_1\vert^{2} & =\frac12\boldsymbol{-}\mathrm{Re}\left(\xi_3\eta^{\boldsymbol{*}}_3\right)=\frac12\boldsymbol{-}\rho_3\cos\phi_3=\frac12\left(1\boldsymbol{-}\sin\theta_3\cos\phi_3\right) \tag{21b}\label{21b} \end{align} \begin{align} \vert\xi_2\vert ^{2} & =\frac12\boldsymbol{-}\mathrm{Im}\left(\xi_3\eta^{\boldsymbol{*}}_3\right)=\frac12\boldsymbol{+}\rho_3\sin\phi_3=\frac12\left(1\boldsymbol{+}\sin\theta_3\sin\phi_3\right) \tag{22a}\label{22a}\\ \vert \eta_2\vert^{2} & =\frac12\boldsymbol{+}\mathrm{Im}\left(\xi_3\eta^{\boldsymbol{*}}_3\right)=\frac12\boldsymbol{-}\rho_3\sin\phi_3=\frac12\left(1\boldsymbol{-}\sin\theta_3\sin\phi_3\right) \tag{22b}\label{22b} \end{align}

Note that the state $\vert\psi\rangle$ of equation \eqref{09} could be expressed as \begin{equation} \vert\psi\rangle \boldsymbol{=}e^{i\alpha_3}\left[\cos\left(\dfrac{\theta_3}{2}\right)\vert u_3\rangle \boldsymbol{+} e^{i\phi_3}\sin\left(\dfrac{\theta_3}{2}\right)\vert d_3\rangle \right] \tag{23}\label{23} \end{equation} or ignoring the phase factor $e^{i\alpha_3}$ \begin{equation} \vert\psi\rangle \boldsymbol{=}\cos\left(\dfrac{\theta_3}{2}\right)\vert u_3\rangle \boldsymbol{+} e^{i\phi_3}\sin\left(\dfrac{\theta_3}{2}\right)\vert d_3\rangle \tag{24}\label{24} \end{equation}

---

B. On Sphere - In Ball

In Figure-01 we see the details of definitions \eqref{18a},\eqref{18b} and \eqref{18c}. This is a plane view from a point on the plane of the circle $\:\rm{K_3}\Xi$ in Figure-03. Note that this Figure-01 is valid if all subscripts $\:'3'\:$ will be replaced by $\:'1'\:$ or $\:'2'$. The definition and meaning of various points with be given in the following.

In Figure-02 we see the geometry of equations \eqref{21a},\eqref{21b} and \eqref{22a},\eqref{22b}. This is a plane view from a point on the positives of the $\:x_3-$axis.

See a 3d view of Figure-03 here

In Figure-03 we have a sphere of diameter 1 in a 3-dimensional space $\:\mathbb{R}^{3}\:$ not identical to the physical space. On the sphere a point $\:\Xi\:$ represents a state of the system \begin{equation} \psi =\xi_1\vert u_1\rangle \boldsymbol{+} \eta_1\vert d_1\rangle = \xi_2\vert u_2\rangle \boldsymbol{+} \eta_2\vert d_2\rangle = \xi_3\vert u_3\rangle \boldsymbol{+} \eta_3\vert d_3\rangle \tag{25}\label{25} \end{equation} Now for $\:\jmath=1,2,3\:$ \begin{align} \rm A_{\boldsymbol{\jmath}} & = point\:\:on\:\:+1/2\:\:of\:\:x_{\boldsymbol{\jmath}}\!-\!axis\:\:representing\:\:the\:\: \vert u_{\boldsymbol{\jmath}}\rangle\:\: eigenstate \tag{26.01}\label{26.01}\\ \rm B_{\boldsymbol{\jmath}} & = point\:\:on\:\:-1/2\:\:of\:\:x_{\boldsymbol{\jmath}}\!-\!axis\:\:representing\:\:the\:\: \vert d_{\boldsymbol{\jmath}}\rangle\:\: eigenstate \tag{26.02}\label{26.02}\\ \rm K_{\boldsymbol{\jmath}} & = projection\:\:of\:\:the\:\:state\:\:point\:\:\Xi\:\:on\:\: x_{\boldsymbol{\jmath}}\!-\!axis \tag{26.03}\label{26.03}\\ \Xi\rm A_{\boldsymbol{\jmath}} & = \vert\eta_{\boldsymbol{\jmath}}\vert=magnitude\:\: of\:\: probability\:\: amplitude\:\: of \:\: \vert d_{\boldsymbol{\jmath}}\rangle\:\: eigenstate \tag{26.04}\label{26.04}\\ \Xi\rm B_{\boldsymbol{\jmath}} & = \vert\xi_{\boldsymbol{\jmath}}\vert=magnitude\:\: of\:\: probability\:\: amplitude\:\: of \:\: \vert u_{\boldsymbol{\jmath}}\rangle\:\: eigenstate \tag{26.05}\label{26.05}\\ \rm K_{\boldsymbol{\jmath}}\rm A_{\boldsymbol{\jmath}} & = \vert\eta_{\boldsymbol{\jmath}}\vert^{2}= probability\:\: of \:\: \vert d_{\boldsymbol{\jmath}}\rangle\:\: eigenstate \tag{26.06}\label{26.06}\\ \rm K_{\boldsymbol{\jmath}}\rm B_{\boldsymbol{\jmath}} & = \vert\xi_{\boldsymbol{\jmath}}\vert^{2}= probability\:\: of \:\: \vert u_{\boldsymbol{\jmath}}\rangle\:\: eigenstate \tag{26.07}\label{26.07}\\ \theta_{\boldsymbol{\jmath}} & = \angle(\Xi\mathrm O_{\boldsymbol{\jmath}}\mathrm A_{\boldsymbol{\jmath}})= polar\: angle \: with\:respect\:to\:the\:x_{\boldsymbol{\jmath}}\!-\!axis \tag{26.08}\label{26.08}\\ \phi_{\boldsymbol{\jmath}} & = \angle(\Xi\mathrm O_{\boldsymbol{\jmath}}\mathrm A_{\boldsymbol{\jmath}})= azimuthal\: angle \: with\:respect\:to\:the\:x_{\boldsymbol{\jmath}}\!-\!axis \tag{26.09}\label{26.09}\\ \omega_{\boldsymbol{\jmath}} & = \angle(\Xi\mathrm B_{\boldsymbol{\jmath}}\mathrm K_{\boldsymbol{\jmath}})= half\:the\:polar\: angle \: \theta_{\boldsymbol{\jmath}} \tag{26.10}\label{26.10}\\ \rm K_{\boldsymbol{\jmath}}\Xi & = \vert\xi_{\boldsymbol{\jmath}}\vert\cdot\vert\eta_{\boldsymbol{\jmath}}\vert= \rho_{\boldsymbol{\jmath}}= radius\: of \: the\:circle,\:intersection\: of\:the\: sphere \nonumber\\ & \hphantom{=}\:\:with \:the\: plane\:through\:point\:\Xi\:normal\:to\:the\: x_{\boldsymbol{\jmath}}\!-\!axis \tag{26.11}\label{26.11} \end{align}

Answer 3

You can associate points on the surface of a unit sphere with pure spin states in the following simple way.

A point of the sphere $(n_x,n_y,n_z)$ is associated with an eigenvector of the operator $n_x\sigma_x+n_y\sigma_y+n_z\sigma_z$ with a positive eigenvalue and vice versa. This includes all spin 1/2 single particle spin states.

And this is not random or visualization or mathematics. If you have a Stern-Gerlach device with a magnetic field inhomogeneity pointing in the direction $(n_x,n_y,n_z)$ then it will consistently deflect that beam in a particular direction when it has that state that is eigen to $n_x\sigma_x+n_y\sigma_y+n_z\sigma_z.$

But isn't this very confusing? If the north and south poles are chosen, then both states are on the same line and not orthogonal anymore,

It isn't confusing in the slightest. The geometry is related to the orientation of the physical device in the lab to which your state gives reliable results. The oppositely oriented device gives reliable results too. This is common for orthogonal states that teonorthgonal states can be eigen to the same operator.

So different points of the Bloch sphere identify different orientations that give the "up" result for different states. Do not confuse the orientation of the measurement device in 3d space with the geometry of the states in spin space.

so how can one choose an arbitrary point $p$ on the surface of the sphere and possibly decompose it in terms of $0,1$ states in order to find $a$ and $b$?

Its the other way around. How did you decide to call some state 0 and another 1? You picked a random orientation and called it z and oriented your device to have the magnetic field inhomogeneity point that way. That gave you an up and a down.

But now we can specify any spin state. You same you have an arbitrary point $(n_x,n_y,n_z)$ then find the eigen vector of $n_x\sigma_x+n_y\sigma_y+n_z\sigma_z.$ with positive eigen value. Call it $\left|s\right\rangle,$ then $$\left|s\right\rangle=\langle 0\left|s\right\rangle\left|0\right\rangle+\langle 1\left|s\right\rangle\left|1\right\rangle$$ so there is your $a$ and $b$ except you don't know the overall phase and magnitude but a single particle spin state doesn't have one of those.

Does this mean that one shouldn't regard the Bloch sphere as a valid basis for our system and that it's just a visualization aid?

No, it means you shouldn't confuse then geometry in the lab with the geometry of the Hilbert space. Physics is an experimental science so they are most definitely related but they are not the same.

If you want to project a vector onto an eigenspaces you don't project the labels onto each other. You can have a spin state and another spin state and when you put one through a Stern-Gerlach device oriented for the other then the spatial degrees of freedom split and separate into one that is up in that direction and one that is spatially down from that direction and the spin state literally changes to point up in the beam that spatially went up and to point down in the beam that went down. So the one particle's spin has become entangled with its own position.

The size of the Hilbert Projection tells you the size of the spatial parts that got deflected and split. But you also don't literally need to remember rules like that. If you write down the Schrödinger equation for the Stern-Gerlach device the beam splits and separates into the correct size parts and the spins align into the two polarizations and it happens without you telling it to do that.

So then the spin state is clear. It is telling you the direction it will reliably go if you give it a chance. And if you put it in a differently oriented Stern-Gerlach it will be forced to go in one of the two directions allowed by that orientation and it will split and go in both. To get the sizes of each part you can evolve the Schrödinger equation or compute the eigenvectors of the operator $n_x\sigma_x+n_y\sigma_y+n_z\sigma_z$ and dot it with the eigenvector of positive eigenvalue orthogonal to the other vector.

And yes there are easier ways to do this and more you can get out of it. But hopefully you see the other geometry.

Could you show how one obtains then the $cos \theta/2$ and $e^{i\phi}$ terms?

I was using the Pauli spin operators, if you want to pick a basis you can write them as matrices (an operator is a function on a vector space, a matrix stands in for an operator after you select a basis; the operator exists and is the same regardless of what basis you may or may not select later). $$n_x\sigma_x+n_y\sigma_y+n_z\sigma_z=\left(\begin{matrix} n_z & n_x-in_y \\ n_x+in_y&-n_z \end{matrix}\right).$$

And the eigenvector with positive eigenvalue is $\left(\begin{matrix} -n_x+in_y \\ n_z-1 \end{matrix}\right),$ unless $n_z=1$ then it is $\left(\begin{matrix} 1\\ 0 \end{matrix}\right).$ Let's deal with the case of $n_z=1$ first, in that case $a=1$ and $b=0$ and $\theta=0$ so $a=\cos(\theta/2)$, $b=e^{i\phi}\sin(\theta/2)$ all works out.

If you want to write the eigenvector as a unit vector you get $\frac{1}{\sqrt{2-2n_z}}\left(\begin{matrix} -n_x+in_y \\ n_z-1 \end{matrix}\right).$ If you want to adjust the phase so that the first coordinate is real and positive then you get $\frac{1}{\sqrt{2-2n_z}\sqrt{n_x^2+n_y^2}}\left(\begin{matrix}n_x^2+n_y^2\\ (n_x+in_y)(1-n_z) \end{matrix}\right).$

The rest is trigometry, e.g. $\frac{n_x+in_y}{\sqrt{n_x^2+n_y^2 }}=e^{i\phi}.$ So we just need to show that $\cos(\theta/2)=\sqrt{\frac{n_x^2+n_y^2}{2-2n_z}}$ and that $\sin(\theta/2)=\sqrt{\frac{1-n_z}{2}}.$ The latter is a trig identity $\sin(\theta/2)=\sqrt{\frac{1-\cos(\theta)}{2}}.$

The former is $$\sqrt{\frac{n_x^2+n_y^2}{2-2n_z}}=\sqrt{\frac{n_x^2+n_y^2+n_z^2-n_z^2}{2-2n_z}}$$ $$=\sqrt{\frac{1-n_z^2}{2-2n_z}}=\sqrt{\frac{(1-n_z)(1+n_z)}{2-2n_z}}$$ $$=\sqrt{\frac{1+n_z}{2}}=\sqrt{\frac{1+\cos(\theta)}{2}}=\cos(\theta/2).$$

Answer 4

A mere extended comment streamlining the fine answer of @Timaeus to a more memorable form.

The state vector
$$ |\psi\rangle= \begin{pmatrix} \cos \theta/2 \\ e^{i\phi} \sin \theta/2 \end{pmatrix}$$ defines a pure state density matrix through its projection operator, $$\bbox[yellow]{ |\psi\rangle \langle \psi | = \begin{pmatrix} \cos^2 \theta/2 & \sin \theta/2 ~ \cos\theta/2 ~e^{-i\phi} \\ \sin \theta/2 ~ \cos\theta/2 ~e^{i\phi} & \sin^2 \theta/2 \end{pmatrix}=\rho }~. $$ Note the manifest invariance under over-all rephasing of $|\psi\rangle$.

The general principles' expression of this idempotent hermitean density matrix is also, evidently, $$ \rho=\frac{1}{2}(1\!\! 1 + \hat n \cdot \vec \sigma) , $$ with $\hat n = (\sin \theta \cos \phi, \; \sin \theta \sin \phi, \; \cos \theta)^T. $

That is, the $\hat z$ axis rotates to the $\hat n$ axis by full (adjoint) rotation angles, specifying a half-angle (spinor, fundamental) operator expression.

Answer 5

Think about photon spin

Thinking about this more concrete case helped me get some useful pictures in my head. There is even a well known a more optics oriented analogue worth having in mind: the Poincaré Sphere.

Photon spin is a two-state quantum system, which as Frobenius mentions, is what the Bloch sphere models.

Photon spin is also easy to understand/visualize/manipulate experimentally.

Physical polarizer filters

First let's think about the most concrete thing possible: the polarizer filters.

There are two types of polarizer filters you could think about:

• linear polarizer, at any angle between -90 and 90.

  E.g. here's one at 90 degrees:

  

  and here's one at 45 degrees:

  

  and here's one at 0 degrees:

  

  Source

  Wikipedia describes a few ways to create such filters, and the above pictures are Polariod filters, which is used in sun glasses and photography and therefore readily available.

  From a quantum mechanics point of view, the 90 and 0 degree orientations make the same measurement: the only difference is that one lets the photon pass but the other blocks it. But we can use both equally to determine the level of linear vertical polarization of the photon: you just have to take the complement the value.

  And since every measurement corresponds to a Hermitian matrix, we can represent both 0 and 90 with a single matrix:

  $$M_0 = \begin{bmatrix} 1 & 0 \\ 0 & -1 \\ \end{bmatrix} $$

  And the matrix for 45 degrees is:

  $$M_+ = \begin{bmatrix} 0 & 1 \\ 1 & 0 \\ \end{bmatrix} $$

• circular polarizer, which as Wikipedia explains is usually made with a quarter wave plate + a linear polarizer:

  

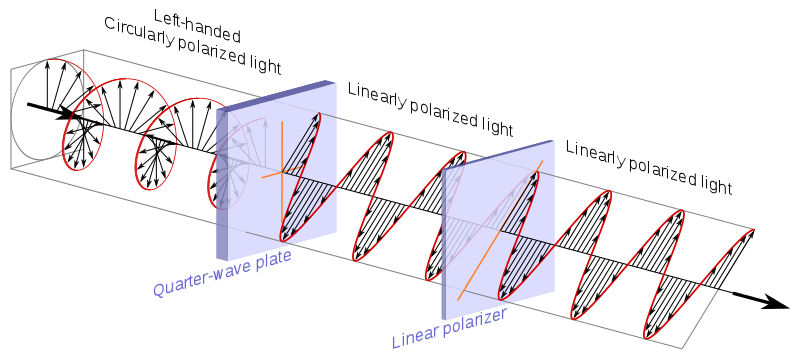
  Source.

  Its corresponding matrix is:

  $$M_i = \begin{bmatrix} 0 & -i \\ i & 0 \\ \end{bmatrix} $$

The above matrices are the so called Pauli matrices.

Some interesting state vectors

Now let's give names to 6 poles representing 6 possible interesting photon states on the Bloch sphere, and try to understand how they interact with the filters.

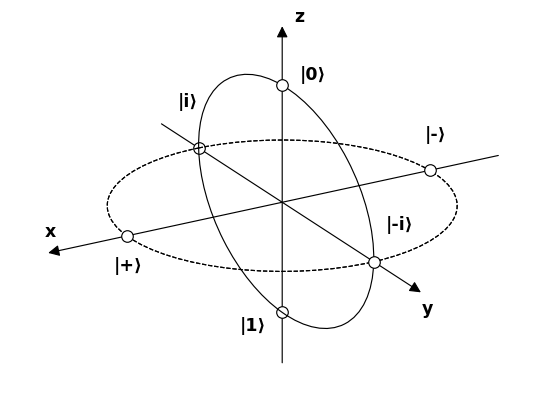
Source.

$$ \begin{alignat*}{4} &\vert 0\rangle &&= &&\begin{bmatrix}1\\0\end{bmatrix} &&= \text{linear 90°} \\ &\vert 1\rangle &&= &&\begin{bmatrix}0\\1\end{bmatrix} &&= \text{linear 0°} \\ &\vert +\rangle &&= \frac{1}{\sqrt{2}} &&\begin{bmatrix}1\\1\end{bmatrix} &&= \text{linear 45°} \\ &\vert -\rangle &&= \frac{1}{\sqrt{2}} &&\begin{bmatrix}1\\-1\end{bmatrix} &&= \text{linear -45°} \\ &\vert i\rangle &&= \frac{1}{\sqrt{2}} &&\begin{bmatrix}1\\i\end{bmatrix} &&= \text{circular clockwise} \\ &\vert -i\rangle &&= \frac{1}{\sqrt{2}} &&\begin{bmatrix}1\\-i\end{bmatrix} &&= \text{circular counter-clockwise} \\ \end{alignat*} $$

The first thing we notice is that the following pairs are all bases:

• $\vert 0\rangle$ and $\vert 1\rangle$
• $\vert +\rangle$ and $\vert -\rangle$
• $\vert i\rangle$ and $\vert -i\rangle$

For example, we could represent:

$$ \begin{alignat*}{3} &\vert 0\rangle &&= \frac{1}{\sqrt{2}}(\vert +\rangle &&+ \vert -\rangle) \\ &\vert 1\rangle &&= \frac{1}{\sqrt{2}}(\vert +\rangle &&- \vert -\rangle) \\ &\vert 0\rangle &&= \frac{1}{\sqrt{2}}(\vert i\rangle &&-i \vert -i\rangle) \\ &\vert 1\rangle &&= \frac{1}{\sqrt{2}}(-i\vert i\rangle &&+ i\vert -i\rangle) \end{alignat*} $$

And then, we also observe that:

• $\vert 0\rangle$ and $\vert 1\rangle$ are eigenvectors of $M_0$
• $\vert +\rangle$ and $\vert -\rangle$ are eigenvectors of $M_+$
• $\vert i\rangle$ and $\vert -i\rangle$ are eigenvectors of $M_i$

If we remember that the result of a measurement in quantum mechanics is the eigenvector of an eigenvalue, with probability proportional to the projection, we get the following sample probabilities for these experiments:

• $\vert 0\rangle$ state on:

  • linear polarizer 90°: 100% pass

  • linear polarizer 0°: 0% pass

  • linear polarizer 45°: 45% pass, because:

    $$\vert 0\rangle = \frac{1}{\sqrt{2}}(\vert +\rangle + \vert -\rangle)$$

  • linear polarizer -45°: 45% pass

  • circular polarizers: 45% pass. This is because a linear state 0 can be decomposed into two circular polarizations:

    $$ \vert 1\rangle = \frac{1}{\sqrt{2}}(-i\vert i\rangle +i \vert -i\rangle) $$

• $\vert 1\rangle$:
  • linear 90°: 0% pass
  • linear 0°: 100% pass
  • linear 45°: 45% pass
  • linear -45°: 45% pass
  • circular: 45% pass
• $\vert +\rangle$:
  • linear 90°: 45% pass
  • linear 0°: 45% pass
  • linear 45°: 100% pass
  • linear -45°: 0% pass
  • circular polarizers: 45% pass
• $\vert i\rangle$:
  • linear 90°: 45% pass
  • linear 0°: 45% pass
  • linear 45°: 45% pass
  • linear -45°: 45% pass
  • circular clockwise: 100% pass
  • circular counter-clockwise: 0% pass

Relative phase

One important semiclassical intuition to remember is that:

circular polarization == two orthogonal linear polarizations 90 degrees out of phase:

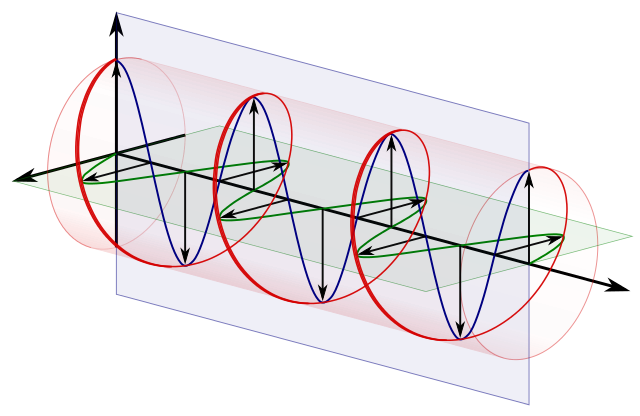
Source.

So for example in:

$$ \vert i\rangle = \frac{1}{\sqrt{2}} \begin{bmatrix}1\\0\end{bmatrix} + \frac{i}{\sqrt{2}} \begin{bmatrix}0\\1\end{bmatrix} = \frac{1}{\sqrt{2}} \vert 0\rangle + \frac{i}{\sqrt{2}} \vert 1\rangle $$

we have a 90 degree relative phase because of the $i$ relative phase difference between $\vert 0\rangle$ and $\frac{i}{\sqrt{2}} \vert 1\rangle$.

But in the diagonal one, they are in-phase relative to $\vert 0\rangle$ and $\vert 1\rangle$:

$$ \vert +\rangle = \frac{1}{\sqrt{2}} \begin{bmatrix}1\\0\end{bmatrix} + \frac{i}{\sqrt{2}} \begin{bmatrix}0\\1\end{bmatrix} = \frac{1}{\sqrt{2}} \vert 0\rangle + \frac{1}{\sqrt{2}} \vert 1\rangle $$

so the relative phase is 0 for that one.

Walk around the sphere

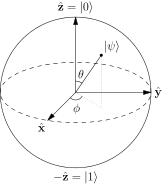
One common way to represent a state in the Bloch sphere is to give just the two $\theta$ and $\phi$ angles as shown below:

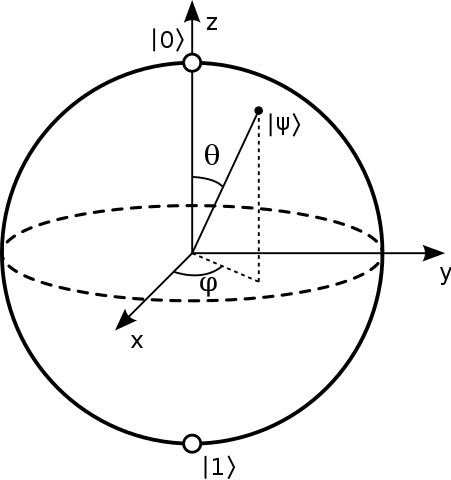
Source.

Since a sphere is non-Euclidean, a good way to visualize it is to walk through some easy to understand paths around it. On the following image we do two paths:

• start at 0, pass through +, 1, -, and return back to 0
• start at 0, pass through i, 1, -i, and return back to 0

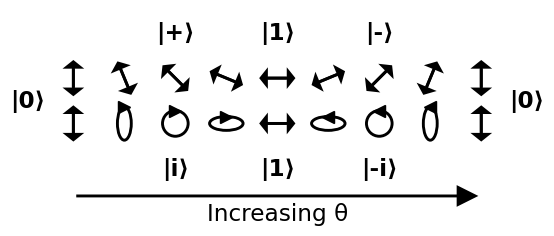
Source.

Walking from + through i, -, -i and back to + is left as an exercise: the circle would become an oblique eclipse, and thins downs more and more into a 45 degree line.

This leads to a clear interpretation of the angles:

• $\theta$: the larger it is, the more likely $\vert 1\rangle$ becomes compared to $\vert 0\rangle$
• $\phi$: the relative phase between $\vert 0\rangle$ and $\vert 1\rangle$. This relative phase cannot be detected by a vertical or horizontal polarizer

How can we go down from 4 real numbers to just 2 in the state?

On the Bloch sphere, we can represent state with only 2 real parameters: the angles $\theta$ and $\phi$

But in the more explicit full full state vectors, there appear to be 2 complex numbers, and therefore 4 real numbers:

$$ \begin{alignat*}{4} &\begin{bmatrix}a + ib\\c + id\end{bmatrix} \\ \end{alignat*} $$

Why one of the numbers must be removed is easy: the total probability has to be 1, and so:

$$a^2 + b^2 + c^2 + d^2 = 1$$

so at that point we are already restricted to a 3-sphere.

The second one is more interesting: we can remove another parameter because the global phase of the state cannot be detected by any experiments and so we are free to choose it arbitrarily.

A global phase $\phi$ is a complex number. The modulus of that number must be 1 to maintain the total probability. Because of this, a natural way to write a global phase is as:

&&e^{i\phi}$$

which automatically satisfies the above property, but sill allows for any possible value.

Experiments cannot detect global phase shifts because the outcomes of measuring:

$$k_0 \vert 0\rangle + k_1 \vert 0\rangle$$

on any of the filters is the same as that of measuring:

$$\text{phase} \times k_0 \vert 0\rangle + \text{phase} \times k_1 \vert 0\rangle$$

because $|\text{phase}| = 1$.

A natural choice is therefore to pick a global phase that rotates the state such that the multiplier of $\vert 0\rangle$ becomes a real number, i.e. setting $b = 0$.

So for example, by multiplying by an imaginary number, we could map more general states into more restricted ones such as

$$ \begin{alignat*}{2} &\begin{bmatrix}i\\0\end{bmatrix} &&\times -i &&= \begin{bmatrix}1\\0\end{bmatrix} &&= \vert 0\rangle \\ &\begin{bmatrix}-i\\0\end{bmatrix} &&\times i &&= \begin{bmatrix}1\\0\end{bmatrix} &&= \vert 0\rangle \\ &\begin{bmatrix}-1\\0\end{bmatrix} &&\times -1 &&= \begin{bmatrix}1\\0\end{bmatrix} &&= \vert 0\rangle \\ &\frac{1}{\sqrt{2}}\begin{bmatrix}i\\i\end{bmatrix} &&\times -i &&= \frac{1}{\sqrt{2}}\begin{bmatrix}1\\1\end{bmatrix} &&= \vert +\rangle \\ \end{alignat*} $$

Why are there exactly three Pauli matrices?

I think there are deep and clear mathematical reasons that explain this, linked to them being a basis of the 2x2 Hermitian matrix space as mentioned at: https://physics.stackexchange.com/a/415228/31891 and https://en.wikipedia.org/wiki/Bloch_sphere#Pure_states and it is the crux of the question of why the Bloch sphere is used, but I haven't fully grasped it.

But in more practical terms: the three measurement devices we described are the only three possibilities (up to global rotations) such that after you pass through one, you lose all information about the other two (50% probability on the other two experiments).

Therefore they are orthogonal in a certain sense, and maximal as there is no other experiment that we could add to that set of experiments such that this property holds.

Play with Quirk

https://algassert.com/quirk

This is another worthwhile suggestion. Click those images until it all makes sense.

Other physical systems

Basically every type of quantum computer provides a physical example of what physical things look like on a Bloch sphere. It would be good to understand the different types in more detail.

Most of them appear to be state 0 on the state of lowest energy, 1 on the first energy level, and anything on the equator is a superposition. TODO physical interpretation/control of phase.