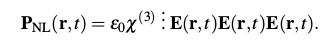0
$\begingroup$

Upon my research of polarization of light I stumbled upon the following formula: enter image description here

What do the three dots mean? I have never seen them before.
Edit: Found this notation in the book "Nonlinear fiber optics", $P_{NL}$ is the nonlinear polarization component, while $\chi$ is unknown to me (haven't found it, but I assume that it is a tensor for the polarization)

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Where did you stumble upon that formula, and what do the variables in the equation denote? $\endgroup$
    – ACuriousMind ♦
    Commented May 28, 2015 at 12:31
  • $\begingroup$ Updated it as far as possible, thanks for mentioning that. $\endgroup$
    – arc_lupus
    Commented May 28, 2015 at 12:43

1 Answer 1

Reset to default
2
$\begingroup$

The triple dots denote a tensor-contraction over three indices. This is a generalization of the notation for the scalar product (which is contraction over one index). The three adjacent terms $E$ are implied to form a tensor product.

$\chi^{(3)}$ is third order term of the perturbation expansion of the full (non-linear) susceptibility (more specifically electrical polarizability; in non-linear optics the susceptibility is expanded in perturbation series, while usually one only considers the linear term).

So $\chi^{(3)}$ is a tensor of rank 4 and three of its indices are contracted with the indices of the $E$-fields giving the polarization $P_{NL}$. I guess the first non-zero, non-linear term is cubic because there is usually no effect analogous to diamagnetism for electrical polarization.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.