4
$\begingroup$

So I am studying for a final and can't seem to solve this. There is a log floating in water and I need to find its weight. The question I have is what parts of the volume of the log count when summing the forces in the Y axis.

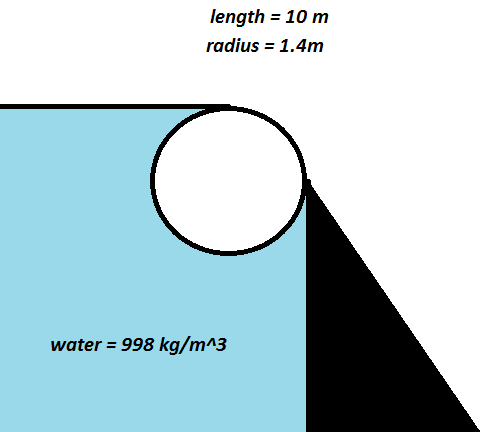
enter image description here

What i have now for AREA alone is $r^2 - .25\pi r^2$ pushes down and $.5\pi r^2 + r^2$ pushes up. however this does not take into account the air above the log in the upper right quadrant. is the force pushing up taking into account the half circle below the water and also the $2r^2$ above the horizontal of the log?

The actual question for this image says: A log is stuck against a dam as shown in the diagram. given the radius of the log of 1.4 m and the length of the log into the page, 10 m find the weight of the log in kN.

Improve this question
$\endgroup$
1
  • $\begingroup$ Is this possible? Would the unbalanced forces acting on the log end up rotating it so that water came up between the dam and the log (or could that be overcome by static friction caused by the water pushing the log against the dam)? $\endgroup$
    – Peter Shor
    Commented Dec 10, 2011 at 20:16

2 Answers 2

Reset to default
6
$\begingroup$

There are two possibilities.

  1. Water is not leaking up between the log and the dam and flowing over the dam. In this case, the pressure on the bottom of the log depends only on the depth, and the amount of force needed to balance the water is the same as the weight of a log with the density of water, with an extra corner added; i.e., the red area in the crude picture below. This would be the weight of water in a volume $L(\frac{3}{4} \!\pi R^2 + R^2)$, where $L$ is the length of the log and $R$ is the radius of the log. (I expect this was not the way that your teacher intended you to solve the problem. Also note that this log is denser than water, which means that it is very difficult to see how it could have reached this position.)

  2. Water is leaking, and flowing up between the log and the dam. In this case, you have a very complicated problem in hydrodynamics which you cannot solve without more information.

picture of log with corner added,

Improve this answer
$\endgroup$
2
  • $\begingroup$ this is correct according to profs answer. tyvm! $\endgroup$
    – joncodo
    Commented Dec 11, 2011 at 2:34
  • $\begingroup$ But I suspect he may have intended you to figure out the answer by integrating the force (which should also have gotten you the same answer, but not as easily). $\endgroup$
    – Peter Shor
    Commented Dec 11, 2011 at 3:58
0
$\begingroup$

Please correct me if I've misunderstood, but wouldn't this still be an application of the Archimedes' principle? If the log is floating then the it would have displaced its weight in water; the force pushing down is balanced by the force pushing up. So if we assume it is completely submerged (this may be a weak assumption), then we know the volume of water displaced and therefore its mass (we are given the density of water). Once we have the mass of water, we can work out its weight.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ But the water on half the log is higher than the other half. $\endgroup$
    – Peter Shor
    Commented Dec 10, 2011 at 22:21
  • $\begingroup$ @PeterShor True. That's why I wasn't sure about the assumption I was making. I think something a long the lines of the first point in your solution is likely the way to tackle it. $\endgroup$
    – Omar
    Commented Dec 10, 2011 at 23:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.