3
$\begingroup$

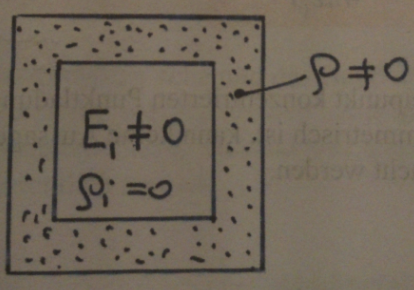
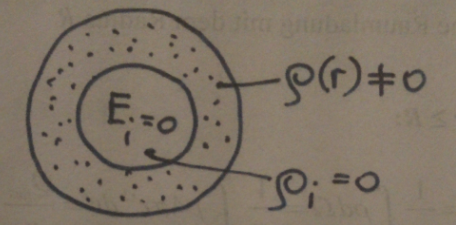
I am currently visiting a course about electrodynamics. In my last lecture it was said that if a hollow sphere is inside of a bigger sphere, but only in the bigger sphere there are spherically symmetrically distributed charges, then the electric field inside of the hollow one would be zero: enter image description here

Ok, this makes sense, if I look at the following equation:

$$ \oint_\Gamma \textbf{E}_id \mathbf \Gamma=\oint_\Gamma E_i d\Gamma=E_i\oint_\Gamma d\Gamma=E_i4\pi r^2=\frac{1}{\varepsilon_0} \int_\Omega \rho d\Omega=\frac{1}{\varepsilon_0}\int_{r=0}^{r=R}\rho 4\pi r^2 dr=\frac{Q}{\varepsilon_0} $$

Then I write $$ E_i4\pi r^2=\frac{Q}{\varepsilon_0} = 0 $$ so the electrical field must be zero, if there are no charges inside, whereas $\Gamma$ is the surface, $\rho$ the charge density and $\Omega$ the volume of a sphere. What I don't understand is, if you look at the next picture, the electrical field is not zero. Why?

enter image description here

Improve this question
$\endgroup$
5
  • $\begingroup$ Hint: when you write $$ ...=\oint_\Gamma E_i d\Gamma=E_i\oint_\Gamma d\Gamma=... $$ You assume that the electric field is constant over the surface of integration (and perpendicular to it). Is this true in the second example? $\endgroup$
    – yohBS
    Commented Mar 19, 2015 at 22:40
  • $\begingroup$ Thank you for the hint! This is definitely not true in the second case, because the electric field expands radially. But I still can't grasp it really. So I have no charges in that cube, but still an electric field is created? $\endgroup$
    – Daiz
    Commented Mar 19, 2015 at 23:08
  • $\begingroup$ If the electric field varies and assumes positive and negative values along the surface you integrate, it can all cancel out to zero. Just imagine the field created by a point charge, and now draw a sphere that doesn't include the point charge. The flux through the sphere is zero in total, but there is still an electric field at all points in space. $\endgroup$
    – MonkeysUncle
    Commented Mar 20, 2015 at 0:14
  • $\begingroup$ The hollow sphere has much more symmetry than the hollow cube. For the hollow sphere, all points on the boundary are equivalent. Since the full surface integral is zero (total flux through boundary sphere is zero), the symmetry of all points on a sphere leads to the $E$ field being identically zero in the spherical case. In the case of a cubical box, some points are different than others. For example, why would $E$ at a vertex (corner of the cube, there are eight) be the same as $E$ at the midpoint of a face of the cube (there are six)? Or midpoint of an edge of the cube (there are twelve)? $\endgroup$
    – Jeppe Stig Nielsen
    Commented Aug 8, 2016 at 7:32
  • $\begingroup$ The fact that this has confused others before you, can be seen in www.physicsforums.com: Electric field inside a uniformly charged cubical box. Some of the posts in that thread are wrong. $\endgroup$
    – Jeppe Stig Nielsen
    Commented Aug 8, 2016 at 7:35

1 Answer 1

Reset to default
1
$\begingroup$

So,the integral is equal to zero because the $Q$ that is enclosed in the Gaussian surface is zero.That does not necessarily mean that the electric field is equal to zero.The only other way for the integral to be equal to zero is if the sum of the dot products inside the integral is equal to zero.It means that you have equal negative dot products as positive ones,thus giving you the integral of $\hat l\cdot d\vec S=0$ where $\hat l$ is the direction of the electric field.
To intuitively think about it you need very good visualization skills.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.