Some doubts regarding electric field inside a hollow shell made of charges

Question

This question is a follow up to this question. Here my doubt is about a shell made up entirely of charges. I am asking this question because all physics and electrostatics/electrodynamics books talk only about conducting shells and spheres. Only a few contain details about a sphere made of charges. There are no details about a hollow shell made of charges. Hence lies the purpose of this thought experiment:

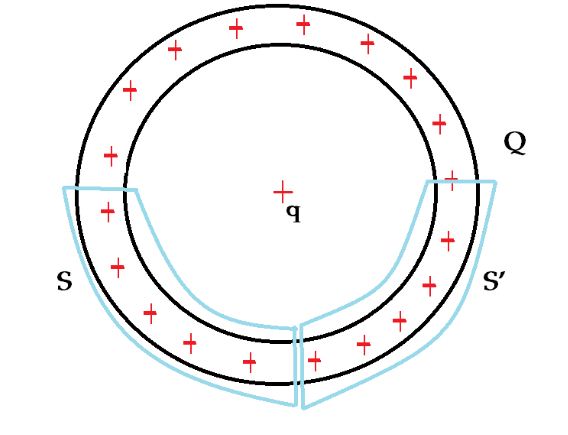
Consider a symmetrical hollow shell made up entirely of uniformly distributed charges such that the total charge is $Q$. We also place a charge $q$ at the centre of the cavity. We also take two Gaussian surfaces $S$ and $S'$. Let the larger radius be $R$ and smaller radius be $r$.

In $S$ we have by using Gauss's Law:

$E_1\pi R^2+E_2\pi r^2+E_32\pi (R^2-r^2)+E_42\pi(R^2-r^2)=\frac{Q}{\epsilon_0}$

Till here I am able to figure out.

Here are my doubts:

1. How do I find the magnitude of electric field $E_1$ and $E_2$?
2. Is $E_2$ is zero? If yes why? If $E_2$ is non-zero, then where do the field lines go?
3. What happens to electric field lines $E_3$ and $E_4$? If we consider a Gaussian surface $S'$ in another quarter of the volume of the shell just like above, that section too would have $E'_3$ and $E'_4$ similar to $E_3$ and $E_4$. Since $E_3$ and $E'_3$ are of same magnitude and directed towards each other and both are created by like charges, what happens to them? Won't there be repulsion or would the situation be stable?
4. The field lines from $q$ are directed radially outwards. But they encounter the positive surface of charge. So what happens to the lines?
5. What would be the net electric field due to the system at a point outside the sphere? Would it be $E_{net}=\frac{Q}{\pi\epsilon_0D^2}$ or $E_{net}=\frac{Q+q}{\pi\epsilon_0D^2}$ ?

I would be very grateful if someone would help me resolve these doubts.

Answer 1

The key to using Gauss' law is to find a good symmetry of the problem. Since you are asking about a hollow shell of charges the obvious symmetry to use is spherical symmetry. Your quarter volume does not have spherical symmetry so it is not a good idea to use it.

How do I find the magnitude of electric field E1 and E2?

First, remove $q$ at the center and focus on the field from the shell. The point charge at the center can be simply added back at the end using Coulomb's law.

Draw Gaussian surfaces in the shape of spheres centered in the center of the shell. For shells of radius $r'<r$ the Gaussian sphere contains no net charge and therefore by Gauss' law the net flux is zero. Then by spherical symmetry the E-field must be zero everywhere.

For Gaussian spheres of radius $R<r'$ the Gaussian surface contains a net charge of $Q$ and therefore by Gauss' law the net flux is $Q/\epsilon_0$. Then by spherical symmetry the E-field must be $Q/(4\pi\epsilon_0 r'^2)$ directed radially outward.

For Gaussian spheres of radius $r<r'<R$ you follow the same procedure, but the charge will be $0<Q'<Q$.

Is E2 is zero? If yes why? If E2 is non-zero, then where do the field lines go?

Yes, see above for why. The field lines go radially outward.

What happens to electric field lines E3 and E4? If we consider a Gaussian surface S′ in another quarter of the volume of the shell just like above, that section too would have E′3 and E′4 similar to E3 and E4. Since E3 and E′3 are of same magnitude and directed towards each other and both are created by like charges, what happens to them? Won't there be repulsion or would the situation be stable?

The quarter volume Gaussian surface is a bad surface to use, but clearly E3 and E4 are both 0 by spherical symmetry.

The field lines from q are directed radially outwards. But they encounter the positive surface of charge. So what happens to the lines?

Nothing, they continue out. Field lines from a positive charge either go out to infinity or they end on a negative charge. Since the shell and the charge in the middle are positive the field lines cannot end on the shell.

What would be the net electric field due to the system at a point outside the sphere? Would it be Enet=Qπϵ0D2 or Enet=Q+qπϵ0D2 ?

Simply add Coulomb's law to the shell field that we found above.