Rolling w/o slipping - does a friction force acts in this scenario?

Question

Assume the following scenario of rolling without slipping:

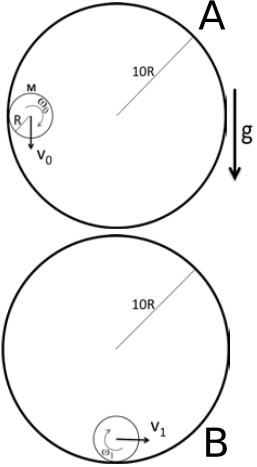
A small disk starts rolling w/o slipping at $\vec{r}_{CM}=-9R\hat{x}$ (scenario A) and reaches the bottom of the large hollow disk (scenario B).

I was told that there is no friction in scenario B, but how is this possible?
In scenario A - I was told that there is friction.

As far as I know, when a wheel rolls w/o slipping, it always has friction in the opposite direction of its movement.

Further explanation:

At the bottom of the disk, the following equation apply: $$(-R\hat{y})\times (-f_s\hat{x}) = I \alpha$$

So $f_s=\mu_s\cdot N$ and $N=m\omega^2\cdot 9R$, so $N > 0$ (because that $\omega > 0$).

How would you resolve the conflict?

Answer 1

As far as I know, when a wheel rolls w/o slipping, it always has friction in the opposite direction of its movement.

Here you go wrong . In pure rolling (that is no slipping ) the bottom point is at rest wrt ground . So there is no kinetic friction acting on it as there is no relative motion .But static friction acts .
In your question gravity accelerates the motion and makes the velocity of the contact point change . Hence kinetic friction starts to act whose direction need not be always opposite to the movement but opposite to the relative motion of the contact point .

Check this out :
* Rolling