2
$\begingroup$

Let's consider an extended version of the Standard Model (SM) with a new Yukawa operator of the form $$ \sum_\ell g_\ell\bar{\ell}\ell \phi ,$$ where $\ell$ is any lepton of the SM and $\phi$ is a new real spin-0 particle, which is assumed to be a singlet of $SU(2)_L$. This new term breaks the $SU(2)_L$ symmetry, but I'll not try to justify its existence.

Now, my question:

  • I want to compute the loop correction to the vertex $\mu e\phi$, which does not exist in the original theory. One possible contribution for this term is show in the figure below (where I also suppose that neutrinos are massive). Does something guarantee that this loop computation will give a finite result in the framework presented here?

$\hspace{6.5cm}$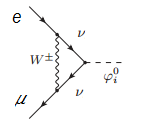enter image description here,

  • If this is not the case, what conditions must be imposed to the lagrangian in order to have finite contributions? Is it enough to have a Hamiltonian with dimension $d\leq4$ operators? Or is it essential to have a perfectly defined gauge theory?
Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

If you are considering massless neutrinos there is no such a diagram since all interactions would preserve flavor. If you take instead massive neutrinos, you are probing lepton flavor violation within the SM since the new interaction with $\varphi$ respects flavor. It is thus very very small, being controlled by the neutrino masses. In turn, it is therefore clear that this diagram is finite since you need to do a mass insertion which makes the integral convergent enough.

Improve this answer
$\endgroup$
10
  • $\begingroup$ Thank you for you answer! But I still don't see why this diagram is finite. If $k$ is the momentum running in the loop, each neutrino propagator comes with $k^{-1}$ and the gauge boson propagator with $k^{-2}$. So, I have something like $\int \frac{dk^4}{k^4}$, which is logarithmically divergent. Where do I get wrong? $\endgroup$
    – Melquíades
    Commented Sep 6, 2014 at 13:15
  • $\begingroup$ this maximally divergent contribution to the integrand is flavor preserving since it knows nothing about the neutrino masses that you have just drop in the power counting. The next term that depends on neutrino masses, the only that can give you off-diagonal flavor terms, must have (at least) an extra $m_\nu/k$ term which makes the integral UV convergent. $\endgroup$
    – TwoBs
    Commented Sep 6, 2014 at 13:51
  • 2
    $\begingroup$ yes, it would be finite. Because no matter how large the mass is, the divergent part of the integral is mass independent and therefore flavor preserving. $\endgroup$
    – TwoBs
    Commented Sep 6, 2014 at 14:20
  • 1
    $\begingroup$ If the coupling $g_\nu$ isn't multiple of the identity in flavor space then the divergent part of that diagram will not be generically be zero. The reason is that $g_\nu$ is another source of flavor violation in addition to the neutrino mass matrix (think about it before diagonalizing the neutrino masses). Technically, you can see that the usual GIM mechanism fails: $V_{ei}V_{\mu i}^* g^{(\nu)}_i\neq 0$ for $g^{(\nu)}_i$ that depends on i. $\endgroup$
    – TwoBs
    Commented Sep 7, 2014 at 7:16
  • 1
    $\begingroup$ Yes, by definition of renormalization you can always reabosorb the divergences in a bare parameter tof the lagrangian. In the case at hand you need it to contain $\bar{e}\mu\varphi+ h.c.$. $\endgroup$
    – TwoBs
    Commented Sep 8, 2014 at 5:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.