Problem with forces acting upon a sloped plane

Question

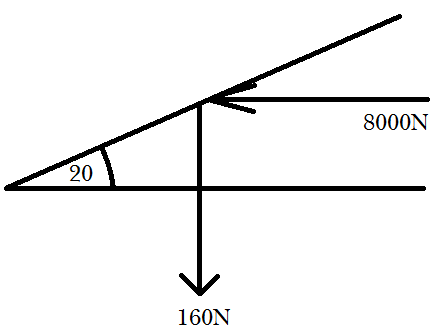
We have a structure that is sloped at 20 Degrees to the horizontal and weights 160N. We then have a force (wind) which is acting along the horizontal plane which has been calculated to be of about 8000N.

The problem we are having is that we are unable to find what amount of ballast that needs to be added at the bottom of the structure to stop the structure from being blown away. The structure will not be bolted to the ground. At the moment we do not have an exact amount for the coefficient of friction, but the structure will be placed on a flat concrete surface.

We have tried to find a solution by breaking the forces along the vertical and horizontal components, but we where unsure if we where heading in the right direction. Below is an image which describes the setting:

Could someone please advise us as to which mathematical model we should use?

Thanks in advance

Answer 1

You have three problems to solve: slipping, tipping around a horizontal axis) and rotationally turning (around a vertical axis).

I would assume that if you solve slipping and tipping, that you will have generated enough static friction to make turning (spinning like a top) not a problem. (YOU need to check this, but I would expect it to be so.) So now you have only tipping and slipping to solve.

For tipping, you pick a convenient point to sum moments. If there is no motion, then the moments must sum to zero. You can hypothesize the case where the structure is just about to tip and see what CW moment is needed to counteract the CCW moment generated by the horizontal force.

In the diagram you drew, if you pick the point of rotation as the vertex of the $20^o$ angle, and take the length from that angle to the point of application of the force to be $L_1$ meters, then the horizontal force acts through a moment arm of ${L_1}{sin(20^o)m}$, and the total Counter-Clockwise (CCW) moment generated is ${8000L_1}{sin(20^o)N^.m}$. The CW moment needed to balance that out is ${((160L_2)+(WL_3}{cos(20^o)N^.m}$, where $W$ is the weight you add to the structure. $L_2$ is the length along the 20 degree slope that would just meet up with a vertical line drawn through the center of the 160N force; and $L_3$ is the distance along the slope that would just meet up with a vertical line drawn through the center of gravity of $W$.

For slipping, you really do need to know the static coefficient of friction, $u_s$ (or make a conservative guess). Then you need to solve for $(160N+W)u_s > 8000N$.

Answer 2

I would treat the point of contact with the ground as having rotational freedom but no freedom of horizontal or vertical motion. From there, the moments of all the forces must balance. I would assign the positive x-axis to be the direction of the slanted object and the origin to be the point of contact with the ground. You then have 3 forces, all acting at different locations along the object. Take $l$ to be the length of the object.

• $F_g$ force of gravity which acts at distance $\frac{1}{2}l$ and angle $\theta=-\frac{8}{9} \pi$ (downward)
• $F_w$ force of the wind that acts at distance $d_w$ and angle $\theta=\frac{8}{9} \pi$ (horizontal)
• $F_b$ force of the ballast that acts at distance $d_b$ and angle $\theta=-\frac{8}{9} \pi$

Alternatively, denote these as $F_i$, $d_i$, and $\theta_i$ for i from 1 to 3. Write the moment balance at the origin.

$$\sum_i F_i d_i sin \theta_i=0$$

If everything else is known then you can solve this for $F_b$.