Converging mirrors and the transition between inverted and non-inverted images

Question

I was talking with a friend recently about concave mirrors, which frequently invert the reflected image - I think we were playing with a spoon. I raised the question, if you had a mirror whose shape could be easily controlled so you could move it from convex through being flat to being concave, at what point does the image flip from being the right way up to being inverted - is there some sort of discontinuity? If not how would this transition occur?

Answer 1

The concave mirror does not necessary leads to an inverted image. It depends how far you are from the mirror. When you are placed between the focal plane and mirror's surface (see Fig. 1) you see a non-inverted image. When you are exactly at the focal plane you see nothing (Fig. 2). And when you are located behind the focal plane, that is usual case, you see an inversion (Fig. 3, 4). Changing mirrors shape can be considered in this context as an equivalent moving of the object relative to the focal plane.

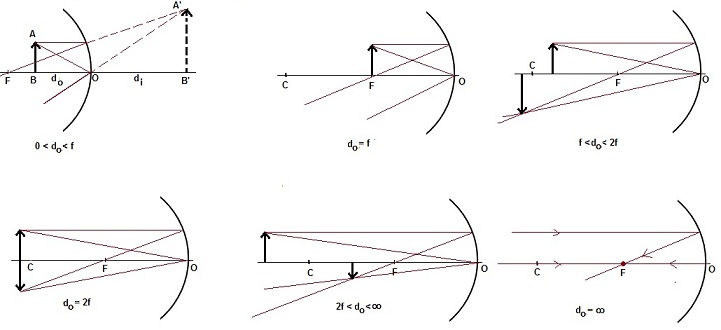
When the object is crossing the focal plane, the image transoms from a very small to a very large abruptly. But you are not able to notice this abruptness since it is very hard to recognize the image approaching the focal plane from the both sides.

Notice that changing the curvature of the mirror is characterized by some critical radius when one observe this effect and this is not the case when the mirror is flat. When the mirror becomes flat the image changes smoothly changing just a zoom.