Let $H\equiv u^2/2+p/\rho+gz=\Omega^2r^2/2+p/\rho+gz$. From Navier-Stokes equation for steady inviscid flow we may derive the following general form of Bernoulli equation (see Fluid Dynamics by Batchelor, Chapter 3):
$$\nabla H=\mathbf{u}\times\omega$$
in which $\mathbf{u}$ is the velocity with magnitude $u$, $p$ is pressure, $\rho$ is density, $g$ is gravitational acceleration, $z$ is height from some reference position, $\Omega$ is the rotation speed about the vertical axis, $r$ is the radial distance from the rotation axis, and $\omega\equiv\nabla\times\mathbf{u}$ is the vorticity. What the equation above says is that $H$ varies in a direction perpendicular to both velocity and vorticity. Equivalently, $H$ is constant along streamlines and vortexlines. In the particular case of irrotational flow, i.e. $\omega=0$, we have $\nabla H=0$, and therefore $H$ has the same value throughout the fluid.
But a liquid in solid body rotation is not irrotational. In fact the vorticity has the constant value $\omega=2\Omega\mathbf{e}_z$ everywhere in the fluid, in which $\mathbf{e}_z$ is the unit vector in the vertically upward direction. Therefore $H$ varies from one streamline to another (the streamlines in solid-body rotation are circles concentric with the rotation axis). If $\mathbf{e}_\theta$ be the azimuthal unit vector then $\mathbf{u}=\Omega r\mathbf{e}_\theta$. Therefore:
$$\nabla H=2\Omega^2r~\mathbf{e}_\theta\times\mathbf{e}_z=2\Omega^2r~\mathbf{e}_r\\
\frac{dH}{dr}=2\Omega^2r\\
\therefore\quad H=\Omega^2r^2+\textrm{constant}$$
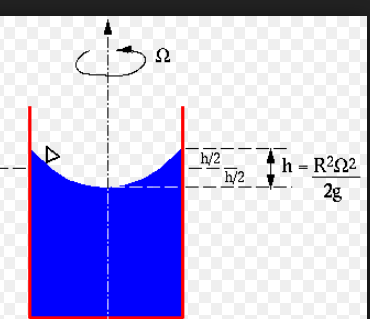
Thus we have the Bernoulli equation for a streamline on the free surface lying at radial distance $r$ from the rotation axis:
$$H=\Omega^2r^2+\textrm{constant}=\frac{\Omega^2r^2}{2}+\frac{p}{\rho}+gz\\
\therefore\quad\frac{\Omega^2r^2}{2}-gz=\textrm{constant}$$
in which constant pressure (at the free surface) has been absorbed into the other constant. This is the equation for the free surface of a liquid in solid body rotation.