What is a $p_x + i p_y$ superconductor? Relation to topological superconductors

Question

I often read about s-wave and p-wave superconductors. In particular a $p_x + i p_y$ superconductor - often mentioned in combination with topological superconductors.

I understand that the overall Cooper pair wavefunction may have orbital angular momentum = 0 (s-wave) or orbital angular momentum = 1 (p-wave) where the first one is spherically symmetric.

Now what does the splitting in a real ($p_x$) and imaginary ($p_y$) part mean? Why is it written in this form and why is that important (e.g. for zero Majorana modes) ?

Answer 1

Symmetry of the superconducting gap

First of all, a bit of theory. Superconductivity appears due to the Cooper pairing of two electrons, making non-trivial correlations between them in space. The correlation is widely known as the gap parameter $\Delta_{\alpha\beta}\left(\mathbf{k}\right)\propto\left\langle c_{\alpha}\left(\mathbf{k}\right)c_{\beta}\left(-\mathbf{k}\right)\right\rangle $ (the proportionality is merely a convention that will not matter for us) with $\alpha$ and $\beta$ the spin indices, $\mathbf{k}$ some wave vector, and $c$ the fermionic destruction operator. $\Delta$ corresponds to the order parameter associated to the general recipe of second order phase transition proposed by Landau. Physically, $\Delta$ is the energy gap at the Fermi energy created by the Fermi surface instability responsible for superconductivity.

Since it is a correlation function between two fermions, $\Delta$ has to verify the Pauli exclusion principle, which imposes that $\Delta_{\alpha\beta}\left(\mathbf{k}\right)=-\Delta_{\beta\alpha}\left(-\mathbf{k}\right)$. You can derive this property from the anti-commutation relation of the fermion operator and the definition of $\Delta_{\alpha\beta}\left(\mathbf{k}\right)$ if you wish. When there is no spin-orbit coupling, both the spin and the momentum are good quantum numbers (you need an infinite system for the second, but this is of no importance here), and one can separate $\Delta_{\alpha\beta}\left(\mathbf{k}\right)=\chi_{\alpha\beta}\Delta\left(\mathbf{k}\right)$ with $\chi_{\alpha \beta}$ a spinor matrix and $\Delta\left(\mathbf{k}\right)$ a function. Then, there are two possibilities

• $\chi_{\alpha\beta}=-\chi_{\beta\alpha}\Leftrightarrow\Delta\left(\mathbf{k}\right)=\Delta\left(-\mathbf{k}\right)$ this situation is referred as the spin-singlet pairing

• $\chi_{\alpha\beta}=\chi_{\beta\alpha}\Leftrightarrow\Delta\left(\mathbf{k}\right)=-\Delta\left(-\mathbf{k}\right)$ this situation is referred as the spin-triplet pairing.

Singlet includes $s$-wave, $d$-wave, ... terms, triplet includes the famous $p$-wave superconductivity (among others, like $f$-wave, ...).

Since the normal situation (say, the historical BCS one) was for singlet pairing, and because only the second Pauli $\sigma_{2}$ matrix is antisymmetric, one conventionally writes the order parameter as $$ \Delta_{\alpha\beta}\left(\mathbf{k}\right)=\left[\left[\Delta_{0}\left(\mathbf{k}\right)+\mathbf{d}\left(\mathbf{k}\right)\boldsymbol{\cdot\sigma}\right]\left(\mathbf{i}\sigma_{2}\right)\right]_{\alpha\beta} $$ where $\Delta_{0}\left(\mathbf{k}\right)=\Delta_{0}\left(-\mathbf{k}\right)$ encodes the singlet component of $\Delta_{\alpha\beta}\left(\mathbf{k}\right)$ and $\mathbf{d}\left(\mathbf{k}\right)=-\mathbf{d}\left(-\mathbf{k}\right)$ is a vector encoding the triplet state.

Now the main important point: what is the exact $\mathbf{k}$-dependency of $\Delta_{0}$ or $\mathbf{d}$ ? This is a highly non-trivial question, to some extent still unanswered. There is a common consensus supposing that the symmetry of the lattice plays a central role for this question. I highly encourage you to open the book by Mineev and Samokhin (1998), Introduction to unconventional superconductivity, Gordon and Breach Science Publishers, to have a better idea about that point.

The $p_{x}+\mathbf{i}p_{y}$ superconductivity

For what bothers you, the $p_{x}+\mathbf{i}p_{y}$ superconductivity is the superconducting theory based on the following "choice" $\Delta_{0}=0$, $\mathbf{d}=\left(k_{x}+\mathbf{i}k_{y},\mathbf{i}\left(k_{x}+\mathbf{i}k_{y}\right),0\right)$ such that one has $$ \Delta_{\alpha\beta}\left(\mathbf{k}\right)\propto\left(\begin{array}{cc} 1 & 0\\ 0 & 0 \end{array}\right)\left(k_{x}+\mathbf{i}k_{y}\right)\equiv\left(k_{x}+\mathbf{i}k_{y}\right)\left|\uparrow\uparrow\right\rangle $$ which is essentially a phase term (when $k_{x}=k\cos\theta$ and $k_{y}=k\sin\theta$) on top of a spin-polarized electron pair. This phase accumulates around a vortex, and has non-trivial properties then.

Note that the notation $\left|\uparrow\uparrow\right\rangle $ refers to the spins of the electrons forming the Cooper pair. A singlet state would have something like $\left|\uparrow\downarrow\right\rangle -\left|\downarrow\uparrow\right\rangle $, and for $s$-wave $\Delta_0$ is $\mathbf{k}$ independent, whereas $\mathbf{d}=0$.

• Note that the $p$-wave also refers to the angular momentum $\ell=1$ as you mentioned in your question. Then, in complete analogy with conventional composition of angular momentum (here it's for two electrons only), the magnetic moment can be $m=0,\;\pm1$. The natural spherical harmonic for these states are then $Y_{\ell,m}$ with $Y_{1,\pm1}\propto k_{x}\pm\mathbf{i}k_{y}$ and $Y_{1,0}\propto k_{z}$, so it should be rather natural to find the above mentioned "choice" for $\mathbf{d}\left(\mathbf{k}\right)$. I nevertheless say a "choice" since this is not a real choice: the symmetry of the gap should be imposed by the material you consider, even if it is not yet satisfactorily understood.
• Note also that only the state $m=+1$ appears in the $p_{x}+\mathbf{i}p_{y}$ superconductivity. You might wonder about the other magnetic momentum contribution... Well, they are discarded, being less favourable (having a lower transition temperature for instance) under specific conditions that you have to know / specify for a given material. Here you may argue about the Zeeman effect for instance, which polarises the Cooper pair. [NB: I'm not sure about the validity of this last remark.]

Relation between $p_{x}+\mathbf{i}p_{y}$ superconductivity and emergent unpaired Majorana modes

Now, quickly, I'll try to answer your second question: why is this state important for emergent unpaired Majorana fermions in the vortices excitations ? To understand that, one has to remember that the emergent unpaired Majorana modes in superconductors are non-degenerate particle-hole protected states at zero-energy (in the middle of the gap if you prefer). Particle-hole symmetry comes along with superconductivity, so we already validate one point of our check list. To make non-degenerate mode, one has to fight against the Kramers degeneracy. That's the reason why we need spin-triplet state. If you would have a singlet state Cooper pair stuck in the vortex, it would have been degenerate, and you would have been unable to separate the Majorana modes, see also Basic questions in Majorana fermions for more details about the difference between Majorana modes and unpaired Majorana modes in condensed matter.

A more elaborate treatment about the topological aspect of $p$-wave superconductivity can be found in the book by Volovik, G. E. (2003), Universe in a Helium Droplet, Oxford University Press, available freely from the author's website http://ltl.tkk.fi/wiki/Grigori\_Volovik.

• Note that Volovik mainly discuss superfluids, for which $p$-wave has been observed in $^{3}$He. The $p_{x}+\mathbf{i}p_{y}$ superfluidity is also called the $A_{1}$-phase [Volovik, section 7.4.8]. There is no known $p$-wave superconductor to date.
• Note also that the two above mentionned books (Samokhin and Mineev, Volovik) are not strictly speaking introductory materials for the topic of superconductivity. More basics are in Gennes, Tinkham or Schrieffer books (they are all named blabla... superconductivity blabla...).

Answer 2

I would like to particularly address this nice question relating the Hamiltonian formulation of this superconducting state (via Bogoliubov-de Gennes (BdG) equation) to the low energy quantum field theory, especially the Topological Quantum Field Theory (TQFT).

1. What is a $p_x+i p_y$ superconductor:

It is a chiral $p$-wave superconductor.

It is an odd-parity and spin-triplet pairing superconductor.

The excited state of $p_x+i p_y$ superconductor around the vortex carries a quantized angular momentum $L$ related to the $p_x+i p_y$ order parameter.

We can write either chiral $p_x+i p_y$ or anti-chiral $p_x-i p_y$ pairing order parameter.

The wave function of the condensate is $$ \Psi_\pm = e^{i \varphi} \bigg[ d_x \Big( -\left|\uparrow\uparrow\right\rangle + \left|\downarrow\downarrow\right\rangle \Big) + i d_y \Big( \left|\uparrow\uparrow\right\rangle + \left|\downarrow\downarrow\right\rangle \Big) + d_z \Big( \left|\uparrow\downarrow\right\rangle + \left|\downarrow\uparrow\right\rangle \Big) \bigg] (k_x \pm i k_y) $$ which we usually simplify it as: $$ \Psi_\pm = e^{i \varphi} \bigg[ i(\vec{d} \cdot \vec{\sigma}) \sigma_y \bigg] (k_x \pm i k_y), $$ where $\vec{d}=(d_x,d_y,d_z)$ and $\vec{\sigma}=(\sigma_x, \sigma_y, \sigma_z)= ( \begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix}, \begin{pmatrix} 0 & -i \\ i & 0 \end{pmatrix}, \begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix} )$, the $$i\vec{\sigma} \sigma_y =(\begin{pmatrix} -1 & 0 \\ 0 & 1 \end{pmatrix}, \begin{pmatrix} i & 0 \\ 0 & i \end{pmatrix},\begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix})$$

where the $2 \times 2$ matrix have the spin-pairing as $ \begin{pmatrix} | \uparrow \uparrow\rangle & | \uparrow \downarrow\rangle \\ | \downarrow \uparrow\rangle & |\downarrow \downarrow \rangle \end{pmatrix} $.

Since chiral $p$-wave superconductor is fully gapped (the pairing gap causes the Fermi sea gapped everywhere in all directions around $\vec{k}_F$), we can ask what is the field theory description. Especially a Topological Field Theory description:

It is a spin-Ising TQFT. It is a fermionic spin TQFT that requires to be defined on the spin manifold. In terms of Chern-Simons (CS) theory, it is a $(SO(3)_1 × U(1)_{−1})$ CS theory. It only has two quasi-particle sectors: $\{1, \psi\}$. The 1 is a bosonic trivial vacuum and the $\psi$ is the fermionic sector related to the Bogoliubov fermion $\psi$ (when one deals with the BdG equation).

---

2. How is it related to topological superconductors?

In a modern definition (of Wen and Kitaev), a chiral $p_x+i p_y$ superconductor is not a Topological Superconductor. A chiral $p_x+i p_y$ superconductor is instead an invertible fermionic intrinsic Topological Order. Topological superconductor as a Symmetry-Protected Trivial State (or Symmetry-Protected Topological State, a SPT state) must be a Short Range Entangled state that has no chiral edge mode. But a $p_x+i p_y$ superconductor has a chiral Majorana-Weyl gapless edge mode (see 3). A chiral $p_x+i p_y$ superconductor is not a SPT state.

So in short, a 2+1D chiral $p_x+i p_y$ superconductor:

• not a SPT state (not a Short Range Entangled Symmetry-Protected Topological/Trivial State)

• not a Topological Superconductor

• an invertible fermionic intinsic Topological Order

However, if we stack a chiral $p_x+i p_y$ with a anti-chiral $p_x-i p_y$ superconductor, what we obtain is a Topological Superconductor respect to $Z_2$-Ising global symmetry as well as a $Z_2^f$-fermionic parity symmetry. So it is a 2+1D $Z_2 \times Z_2^f$-Topological Superconductor. And indeed the 1+1D edge modes on the boundary of the system have central charge $(c_L,c_R)=(1/2,-1/2)$, thus the chiral central charge $c_L-c_R=0$ (mod 4), which is indeed a non-chiral edge mode and a gappable edge by breaking the $Z_2$-Ising global symmetry with some appropriate interactions.

It turns out that stacking from 1 to 8 layers of such $Z_2 \times Z_2^f$-Topological Superconductor ($p_x+i p_y/p_x-i p_y$), you can get 8 distinct classes (and at most 8, mod 8 classes) of TQFTs. They are labeled by $\nu \in \mathbb{Z}_8$ classes of 2+1D fermionic spin-TQFTs:

There are a list of topological invariant data given above. Such as topological ground state degeneracy (GSD), reduced modular $S^{xy}$ and $T^{xy}$ matrices for anyonic statistics.

The 8-th class is the same as the 0-th class.

More details are here.

3. How is it related to Majorana modes?

A 2+1D chiral $p_x+i p_y$ superconductor has a 1+1D boundary chiral Majorana-Weyl gapless edge mode, which has a central charge $c=1/2$. The vortex of $p_x+i p_y$ superconductor traps the Majorana zero modes. The dynamical vortex with this non-Majorana zero mode can be identified as the $\sigma$-anyon in the Ising TQFT with quasi-particle sector $\{1, \psi, \sigma\}$.

Note added 1: If we consider the odd classes ($\nu=1,3,5,6$) $\in \mathbb{Z}_8$ class Topological Superconductor described in the above part (2), there exists a special non-Abelian anyon, usually denoted $\sigma$ anyon. If we take this $\sigma$ anyon trace around the trefoil knot as the worldline in the spacetime trajectory, we can get a statistical Berry phase $(-1)$. This is related to the mathematics of Arf invariant. One can derive that.

Usually the non-Abelian anyon has a non-Abelian statistical Berry matrix when doing braiding process, but the trefoil trajectory worldline (below) for $\sigma$ anyon gives only an Abelian phase $(-1)$.

Note added 2: The illustration of stacking $\nu$-layers of (Ising/$\bar{\text{Ising}}$ TQFT or p+ip/p-ip) superconductors:

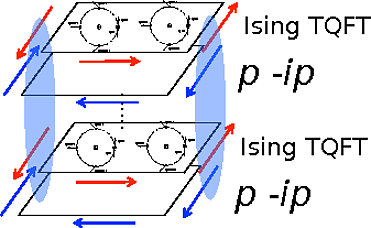
The winding figures illustrate the 1/2-quntum vortices ($\frac{hc}{2e}$ flux) that traps Majorana-zero mode as a non-Abelian $\sigma$ anyon.

=======================================

The more details can be read in this Reference: arxiv 1612.09298 Annals of Physics 384C (2017) 254-287