0
$\begingroup$

I am sorry if my question is too simple, but I am a bit of a beginner on tension. So this is the problem:

A system of two objects suspended over a pulley by a flexible cable is sometimes referred to as an Atwood’s machine. Here, let the mass of the counterweight be 1000 kg. Assume the mass of the empty elevator is 850 kg, and its mass when carrying four passengers is 1150 kg. For the latter case calculate (a) the acceleration of the elevator and (b) the tension in the cable.

So they call: $$m_E = 1150kg$$ $$m_C = 1000kg$$

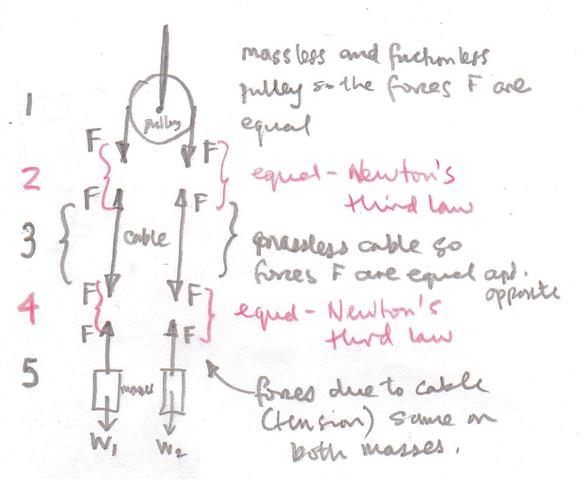
Now what I don't understand from the explanation I was given is that the tension force and acceleration for both objects is the same in this example.

We leave the motor out of the system for this calculation, and assume the cable’s mass is negligible and the pulley is frictionless and massless, which assures that the “tension” in the cord has the same magnitude on both sides of the pulley.

How can tension force be the same if tension force is the weight plus the net force, and if the weight is different for both objects, then how is the tension and acceleration the same magnitude on both objects?

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Possible duplicate of Why I think tension should be twice the force in a tug of war $\endgroup$
    – garyp
    Commented Nov 27, 2016 at 16:44
  • $\begingroup$ @garyp I get that example because both person A and B are pulling with the same force. However, the two elevators have different weights, pulling with different forces $\endgroup$
    – Pablo
    Commented Nov 27, 2016 at 16:47
  • $\begingroup$ Not a duplicate since this one involves acceleration in an essential way. @Pablo: The(magnitude of) acceleration of the two objects is obviously the same, since they are connected by the cable. However, the accelerating force (your net force) will be different between the two, since their masses are different. So, in one case that force is $F_{a_E}=m_E\, a$, in the other it's $F_{a_C}=m_C\, a$. Note also that the signs of the forces will be different between the two. $\endgroup$
    – Pirx
    Commented Nov 27, 2016 at 16:55
  • $\begingroup$ @Pirx but why is the acceleration of the objects the same if they are both pulled by different weights? $\endgroup$
    – Pablo
    Commented Nov 27, 2016 at 17:02
  • 1
    $\begingroup$ The cable keeps the two objects at fixed positions relative to each other. Think about what that means. And, no, the two objects accelerate according to the respective net forces acting on them, which includes the force from the rope, not just the weight. $\endgroup$
    – Pirx
    Commented Nov 27, 2016 at 17:12

2 Answers 2

Reset to default
1
$\begingroup$

If the cable is inextensible then the acceleration of the two masses must be the same.

Work down the diagram from 1 to 5 to show that the tension in a massless cable (forces exerted by cable on the two masses) is the same.

enter image description here

Improve this answer
$\endgroup$
0
$\begingroup$

The confusion comes from thinking that "the tension force is the weight plus the net force" for both masses.

For the lighter mass the tension force is its weight plus the net force on it $T=W_C+m_Ca$, but for the heavier mass, the tension is force is its weight minus the net force on it $T=W_E-m_Ea$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.