1
$\begingroup$

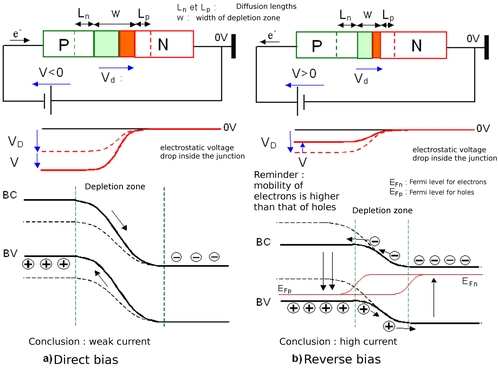
I asked a more general Question before, in whiches answer this question arose: If you look at this picture (I have similar pictures in my books on the topic, where they evade the question):

enter image description here,

(where I assume $E_{Fn}$ and $E_{Fp}$ to be meant as the (quasi-) chemical potential of the holes and the electrons in that point). In b) there is drawn a plot of $E_{Fn}$, and it is curved (obviously because of the influence of a applied potential). $E_{Fn}$ is curved in such a way, that its values far in the n and far in the p-Region differ exactly by the the applied potential difference between the two regions (That means, applying a voltage V shifts the chemical potential at one end of the pn-junction by an Energy eV).

I want to know why the chemical potential $E_{Fn}$ is shifted exactly(!) like that? Is there any quasi-equilibrium-condition that forces it to do so? In what region is this condition valid? What theory describes this behaviour? Is has to be some theory that makes statements about chemical potentials, but it isn't thermodynamic, which just makes statements about thermodynamic equilibrium (this junction is not in equilibrium).

Improve this question
$\endgroup$
9
  • $\begingroup$ Each part of the material will be in steady state, with that steady state being a reflection of the detailed balance of electrons vs holes in the material (based on local conditions such as doping). The overall state also has to reflect the fact that you've jacked up one end of the material by a potential V, which directly impact the chemical potential on that side of the junction. $\endgroup$
    – Jon Custer
    Commented Oct 11, 2016 at 18:01
  • $\begingroup$ yes, and I want to know how exactly V directly impacts the chemical potential (and let's the chemical potential stay shifted) $\endgroup$
    – Quantumwhisp
    Commented Oct 13, 2016 at 13:42
  • $\begingroup$ How does raising an object in a gravitational field impact the chemical potential? (This is a standard thermodynamics class question). $\endgroup$
    – Jon Custer
    Commented Oct 13, 2016 at 18:52
  • $\begingroup$ Yes, if the distribution of the particles stays the same, increasing the potential energy will increase the chemical potential. But who ensures that the distribution stays the same? Let's say I divide a box, and increase the potential energy at one side of the box--> The chemical Potential is shifted. Many particles will flow to the other side of the box, until the chemical potential is constant again. $\endgroup$
    – Quantumwhisp
    Commented Oct 13, 2016 at 21:58
  • $\begingroup$ Yes, so what does the voltage source do? $\endgroup$
    – Jon Custer
    Commented Oct 13, 2016 at 22:29

1 Answer 1

Reset to default
3
$\begingroup$

The total chemical potential of an electron system (which includes electrostatic potential), also called electrochemical potential or Fermi level, represents the energy you need to add an electron to the system in thermal equilibrium including work performed in electrostatic fields. Therefore, when you apply an electrical potential difference to two isolated bodies (like a p- and an n-y type semiconductor), each in thermal equilibrium, the electrochemical potential of the semiconductors will differ just by this voltage.This situation occurs when you apply a voltage to a pn-junction and you can assume that the p- and n- regions are still approximately in equilibrium and therefore still possess an electrochemical potential. This is the reason why the quasi-electrochemical potentials are shifted exactly by the applied voltage. Then one speaks of local equilibrium and local electrochemical potential (or quasi-electrochemical potential). This local quasi-Fermi level concept is often extended to local electron and holes quasi-Fermi levels in semiconductor devices with applied voltages and currents that are as a whole not in thermal equilibrium. These quasi-Fermi levels for electrons and holes are, in general, different and their slope corresponds to the local diffusion+drift current. Applying zero voltage means that the two semiconductors are in thermal equilibrium and have the same electrochemical potential (Fermi level) which, due to the difference in (non-total) internal chemical potentials, in general, produces an electrical potential difference called contact potential. This equilibrium potential difference, however, seen in pn-junctions, cannot be measured with a voltmeter because there is no difference in electrochemical potential between the n- and the p-region.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Thank you, this is helpfull. But usualy, when the chemical potential not constant, there would be changes in the system, until it is constant (that would be, the system reaches equilibrium). When the voltage is applied, the quasi-electrochemical potentials are shifted, as you said. But then usualy, there will be drift currents that try to restore equilibrium. This is not the case here (the shifted electrochemical potentials remain shifted). Why? $\endgroup$
    – Quantumwhisp
    Commented Oct 12, 2016 at 14:06
  • $\begingroup$ The shifted electrochemical potentials of the n- and p-regions remain shifted because you enforce it with the applied voltage. $\endgroup$
    – freecharly
    Commented Oct 12, 2016 at 14:59
  • $\begingroup$ The applied voltage is just a condition for the electric potential in the divice to differ by $V$ at the borders of the divice. You could still achieve a constant chemical Potential by having many more particles at the side (n or p) where the potential is lower. I'm sorry that I'm not yet satisfied, I might be annoying for not accepting the answer. Comments are not a fast way to paraphrase what I'm talking about. $\endgroup$
    – Quantumwhisp
    Commented Oct 13, 2016 at 13:40
  • $\begingroup$ As I pointed out above,when you apply to two isolated electron systems, each in thermodynamic equilibrium, an electrostatic potential difference the electrochemical potentials will differ by this applied voltage but no steady current will flow between them. In the pn-junction the contacts plus n- or p-regions are assumed to be approximately in thermodynamic equilibrium each when you apply a voltage between them, in spite of the current flowing. Therefore the n- and p-regions have (quasi-) electrochemical potentials that differ by the applied voltage, like in the case of the isolated systems. $\endgroup$
    – freecharly
    Commented Oct 13, 2016 at 13:50
  • $\begingroup$ When you say "the contacts are in thermodynamic equilibrium", then you mean that holes and electrons are in equilibrium, and there is no charge? (for example in an undoped semiconductor, equilibrium would mean there are as mutch electrons as holes?) If this is what you mean by equilibrium, and this is also the situation at the contact, then I get what you mean. $\endgroup$
    – Quantumwhisp
    Commented Oct 13, 2016 at 14:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.