1
$\begingroup$

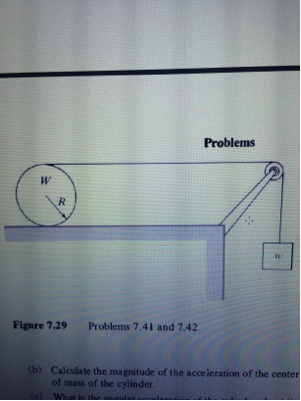
I got a box hanging from a rope that passes through an ideal pulley that attaches to the superior part of a Cilynder and makes it rotate. An exercise asks me two things: a) determine the magnitude of the acceleration of the block b) what is the magnitude of the acceleration of the center of mass of the Cilynder?

My question is: shouldnt the acceleration of center of mass of the Cilynder be equal to the block? Since angular acceleration= acceleration/radius enter image description here

Improve this question
$\endgroup$
4
  • $\begingroup$ No. Use Newton's second law for rotations and $a = \alpha r$. $\endgroup$
    – QuantumBrick
    Commented May 24, 2016 at 20:03
  • $\begingroup$ Are you sure quantum brick? When I try to find the distance covered by the center of mass of the cylinder, I find that it is rθ, with θ measured in radians, and since θ=1/2*t^2*α, and α=ar, it follows that the distances covered by the block and the center of mass of the cylinder are the same, and from that it follows that the acceleration is the same. Am I missing something here? $\endgroup$
    – Andreas C
    Commented May 24, 2016 at 20:50
  • $\begingroup$ When center of cylinder moves $x$, the top moves $2x$. $\endgroup$
    – Floris
    Commented May 24, 2016 at 22:59
  • $\begingroup$ Floris, it makes sense, according to the solutions of the exercise, a) is twice of b). But How can I get that assumption? $\endgroup$
    – Vitor Aguiar
    Commented May 25, 2016 at 0:05

1 Answer 1

Reset to default
2
$\begingroup$

If you see on the cylinder as a wheel then note that its center moves twice slower than top point attached to the block. Same is for acceleration. In each moment the cylinder rotates around the point of its touch to the table, so radius from touch-point to the center is twice less than radius to the cylinder top point.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.