Detailed form of light waves in vacuum and how to test it experimentally?

Question

Consider a light wave in vacuum.

• Do the $\vec{B}$ and $\vec{E}$ fields have to be orthogonal to each other? Since you can add constants to a solution to Maxwell's equation it doesn't seem neccesary from theory.

• How would one measure if the $\vec{B}$ and $\vec{E}$-vectors of a light wave are perpendicular?

• Must it be a plane wave? How to determine that by measurement?

• Must $\vec{E}$ and $\vec{B}$ be in phase? How to test this experimentally?

Edit:

A part of my question is covered in Does the direction of propagation of the natural light is perpendicular to the direction of electric and magnetic field making up natural light? but that question is closed and the answers doesn't help. However my question is more about experiment than theory, so is a different question.

Answer 1

The d'Alembert wave equation for $\mathbf B$ and $\mathbf E$ results from the Maxwell equation in the vacuum. Suppose a solution for a limited region of space and time.

That solution can be then expressed as an infinite Fourier series, where each term has the form of a plane wave: $\mathbf A_k\exp{(\mathbf{k.x}-\omega t + \phi)}$. It can be shown from the Maxwell equations that for each plane wave, $\mathbf B$ and $\mathbf E$ are orthogonal and in phase. But it doesn't mean that for a given $\mathbf x$ and $t$, $\mathbf E$ and $\mathbf B$ of the complete solution, sum of all plane waves, are also orthogonal and in phase. For any two of them, if $\mathbf {E_1.B_1} = \mathbf {E_2.B_2} = 0$, $(\mathbf {E_1} + \mathbf {E_2})\mathbf .(\mathbf {B_1} + \mathbf {B_2})$ is not zero in general.

The complete solution is not a plane wave, because a sum of plane waves of several directions is not itself a plane wave, not even can be called a wave.

And for each $\mathbf x$ and $t$, the resultant fields from the sum of the plane waves, $\mathbf {E}$ and $\mathbf {B}$ are vectors changing with time, where the concept of being in phase doesn't have a meaning. For example, in the previous example, it is possible that $\mathbf E_1 = -\mathbf E_2$ in a given place and time, vanishing momentarily the resulting electric field, while $\mathbf B_1 + \mathbf B_2 \neq 0$

Answer 2

Although dipoles are very popular in EM theory and are well understood by antenna engineers, they are just one of an infinite set of radiating structures. any structure that contains accelerating coulomb charges will radiate. Where and when the conditions that define plane waves occurs is complex and requires a solid knowledge of modern EM theory derived from Maxwell. You must first define what defines your plane wave. One definition you may use is that it not be a spherical wave. This is difficult condition to achieve in a vacuum or a finite volume of an isotropic medium. A dipole or any finite source will produce spherical waves. At huge distances from such a source the wave approaches a plane or flat wave over a small area. Another definition is that the E and B field are in phase and are orthogonal, this is the only true radiating field that will propagate indefinitely. The field reduces in amplitude inversely with distance from the source.

The impedance of a plane wave is at rest with the medium in which it travels when the wave impedance is equal to that of the medium in which it travels. The impedance of free space is 377 Ohm’s, coax cable 50 Ohm’s and wave-guide 1 Ohm. If you tamper with E and B in any way that departs from the matched and orthogonal condition then long distance transmission is impaired.

Having said all this for near field applications or experiments you can do whatever you like to the fields. If you wish to measure the magnitude and or phase of only the E component of the field then you want the impedance of you probe to be grossly mismatched to the B component this can be achieved by using a very short non resonant dipole (several thousand Ohm’s). To measure the B component of the field use a small non resonant loop, such a loop should also grossly mismatch the impedance of the field and be in the order of 1 Ohm for 377 Ohm plane waves. Appropriately designed probes can enable you to measure the impedance and orthogonality of waves. A dipole will simultaneously support near and far field components in a state of superposition near to the dipole. The only component that will radiate into space is the orthogonal component. The reactive non in phase components remain close to the dipole exchanging energy with one another

Answer 3

Do the $\vec{B}$ and $\vec{E}$ fields have to be orthogonal to each other?

Not really, because aren't really two different fields there. The depiction of two orthogonal waves is not realistic. See section 11.10 of Jackson's Classical Electrodynamics: "one should properly speak of the electromagnetic field Fμν rather than E or B separately". Also see Wikipedia: "the electric and magnetic fields are better thought of as two parts of a greater whole".

Since you can add constants to a solution to Maxwell's equation it doesn't seem necessary from theory.

Agreed.

How would one measure if the $\vec{B}$ and $\vec{E}$ vectors of a light wave are perpendicular?

There aren't really two different and perpendicular vector-field variations. There's just one electromagnetic field variation.

Must $\vec{E}$ and $\vec{B}$ be in phase?

Yes. See the Derivation from electromagnetic theory section of the Wikipedia electromagnetic radiation article:

"Also, E and B far-fields in free space, which as wave solutions depend primarily on these two Maxwell equations, are in-phase with each other. This is guaranteed since the generic wave solution is first order in both space and time, and the curl operator on one side of these equations results in first-order spatial derivatives of the wave solution, while the time-derivative on the other side of the equations, which gives the other field, is first order in time, resulting in the same phase shift for both fields in each mathematical operation."

It's like E is the spatial derivative of the electromagnetic wave, whilst B is the time derivative. To get the gist of this, imagine you're out at sea in a canoe, and a tsunami comes along. As it approaches, your canoe starts to slope upwards, slowly at first, then faster. Then the slope starts to flatten out, and your canoe is momentarily horizontal on the top of the tsunami, then the process is reversed, something like this:

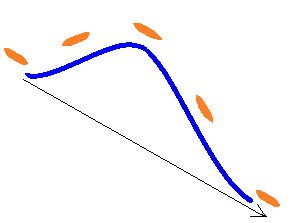
The slope of your canoe denotes E, and the rate of change of slope denotes B. One is the spatial derivative, the other is the time derivative. This is why we have Faraday's law $\vec \nabla \times \vec E = - \frac{\partial \vec B}{\partial t}$. The crucial thing to appreciate is that the curl of E is the rate of change of B. One doesn't cause the other, they're just two aspects of the same thing. For an electromagnetic wave, E and B change together because there's only one wave there. Like I said, the depiction of two orthogonal waves is not realistic.

_{Image courtesy of mathematica}