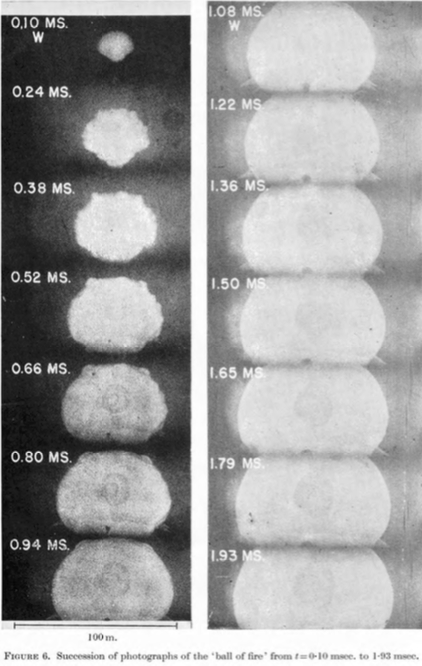
I was recently shown a very interesting sequence of images depicting the expansion of the fireball of Trinity explosion, a nuclear weapon's test conducted in New Mexico in 1945.
I read here that the radius as a function of time $t$ of a (nuclear) explosion depends pre-eminently on the explosion's energy $E$ and the surrounding medium's density $\rho$. Interestingly, there exists a unique way of constructing a length scale from the three quantities $E$, $\rho$, and $t$, as can be verified by dimensional analysis. It is $$ R = \left(\frac{E \, t^2}{\rho}\right)^\frac{1}{5}. $$ Consequently, by reading off the explosion's radius at $t = 0.94 \, \mathrm{ms}$ from the above image as roughly $R \approx 70 \, \mathrm{m}$ and assuming as surrounding density Earth's atmospheric density $\rho_\text{E}$ at sea level, we can get an idea of the energy of the explosion: $$ E = \frac{\rho \, R^5}{t^2} \approx 7.28 \cdot 10^{13} \, \mathrm{J} \simeq 17.4 \, \mathrm{kt} \text{ of TNT}. $$ This is basically what Geoffrey Taylor did in his 1949 paper (he guessed $16.8 \, \mathrm{kt} \text{ of TNT}$). It stirred up quite a fuss in its days by calculating and publicly announcing this and a number of other pieces of classified information about the US Trinity test which he extracted just from the above images (they had been declassified in 1947, two years after the test).
Here comes the question: Based on this information how can I estimate the temperature inside the explosion's fireball itself, i.e. after the shockwave has passed, say $t = 1 \, \mathrm{ms}$ after the explosion?
I thought about using $E = \frac{3}{2} N k_B T$. However, I don't know how to get a handle on the number of particles inside the fireball even if I know its volume. The density in there must be far lower than the surrounding Earth atmosphere.