What is the physical intuition behind the fact that 'energy is not continuous'?

Question

First of all I am a novice regarding my knowledge of quantum mechanics. But curiously I do want to know what is the problem if energy is continuous like spontaneously flowing tap water.

In fact I actually don't know what is continuous referring here. What does this statement mean? Energy is the ability to do work. So, what is the problem with continuous energy?

Plank solved the problem of Classical physics by chopping up the energy into discrete particles which possess energy proportional to the frequency of the radiation.

So, what is this? What led Planck to chop the energy? What is the physical intuition behind this? Please help giving me a math-free explanation.

Answer 1

What is meant by continuous or discrete is the values that the energy of a specific system can take when you make a measurement. For instance, for a free particle, the energy is continuous in the sense that the energy of the particle can take any value (any real number). But in a discrete energy system, like an electron orbiting in an atom, the energy that the electron can have is not arbitrary, in the sense that it can take a value on only specific numbers. So, in the first case, you can increase the energy of the free particle by any small arbitrary amount, in the case of the electron, if you want to increase its energy, you have to give it at least an amount of energy that makes it "jump" to the next allowable energy level. The electron cannot have any intermediate energy between those levels. This is big simplification, so I m sure I will be down voted by my "colleagues". Thanks in advance.

For the origins of quantum mechanics, please read a popular science book, it is a little too long (or at least Wikipedia).

Answer 2

Let me first give you an example on what 'continuous' and 'discrete' are, and then show you how it relates to energy.

Let's say water is flowing in a stream. We say that the water flow is 'continuous' since we don't see induvidual 'blocks' or 'lumps' of water flowing one after the other in the stream. All we see is one continuous indivisible 'body' of water which negotiates rocks and ridges with ease by simply 'flowing' over them.

It may seem apparent at first that energy also behaves in the same manner. The way we think of energy untuitively is that it is some sort of invisible continuous fluid which floats in space from body to body. The evidence to support this intuition physically is not scanty.

Look at the example of visible spectra. This is what you get when you pass a beam of white light through a prism. Prior to the 20th century, the theory that explained the properties of light satisfactorily was the Wave theory of light. According to it, each color of light corresponds to a particular wavelength, and thus also corresponds to a particular energy with which it moves. Since this spectra is continuous, it means that the white light has all energy levels or colors, there are no gaps or sudden color changes. Can you make out exactly where, for example, yellow turns orange ?

But then take a look at this spectra too:

Do you see bold, discrete and distinct lines of red and blue ? This is a hydrogen spectra. The colors are the different wavelengths of light emitted by hydrogen gas filled in a tube when current is passed through it. These distinct lines baffled 19th century physicists. They couldn't understand how only particular 'discrete' wavelengths of light could be emitted by hydrogen atoms and not 'continuously' like the visible spectra.

At the start of the 20th century, Max Planck was conducting an experiment concerning black body radiation. By chance, he observed that in the energy values he tabulated, the values were always integral multiples of $h\nu$, where $\nu$ is the frequency of radiation emitted by the black body. It conlusively meant that energy could only be emitted in specified amounts and had an elementary, basic unit much like the 'lumps' of water I described in the second paragraph. If energy is transmitted in space from body to body, it means that that energy is made up of such elementary 'lumps' called 'quanta' (later they termed it as 'photon', a particle-like entity), much like all mass is made up of 'atoms'.

The 'discrete' wavelengths in the hydrogen spectra was due to the atoms collectively emitting 'discrete' photons of specified energy and not of any energy (as in the continuous spectrum) as electrons jumped to lower energy levels from higher ones.

Another question may arise: 'Why' does energy have to only be transmitted in discrete, specified quanta ? The answer ultimately is that it 'just is'. Later in the 20th century, Einstein showed that energy and mass are two sides of the same coin. So one may also argue that: Just as mass has a quanta called 'atom', energy also may have such a quanta in the form of 'photons'. Eitherwise, none of these can be given as 'the one and only reason' since it is purely a question of philosophy, not physics.

P.S: As julian fernandez said, it will take a really long time to fully type out the origins of quantum mechanics. What I have given is just a brief intro to the field.

Hope it helps !

Answer 3

First two answers to your questions must have gave you some good understanding of Energy, Spectra & some history of early Quantum Mechanics.

Please read them again before you read my analogy.

I'm directly moving onto analogy without wasting "Space" & "Time".

In Classical systems, the movement of energy is like an movement upwards on a Ramp.

In Quantum systems, the movement of energy is like movement upwards on Stairs.

On a ramp, the moment is straight and continuous. The higher work done - the higher you go.

On a stair case, the moment is not continuous. Each step you take for a higher planes requires a "unique" value of work done to reach it.

Consider Photo-Electric effect : (If you don't know what it is, Wiki it)

Imagine you're using Red light to strip of Electrons from a Iridium Metal. Iridium is one of those which requires almost close to 6 eV to strip of electrons from it's surface.

Among the visible region Red is the one with very low frequencies - naturally low energy. If we irradiate the Red light on the Iridium metal - at first, as expected - the electrons don't strip off. If we keep increasing the "Intensity" of red light i.e. giving more & more Red light on the metal - From Classical idea of Energy, we'd expect -- energy must keep increasing in some systemic way and eventually electrons have to strip off.

Here's exactly where Classical Mechanics ends & Quantum Mechanics rise.

From first answer by Mr.Gaurav, you can clearly understand that Red light energy can only take unto a certain level of stairs. After that it stops. However, intensity you provide after that ,.. it doesn't matter. It reaches it's maximum & start decreasing there after.

Look at this Graph - Each Wavelength of varying Intensity with Temperature.

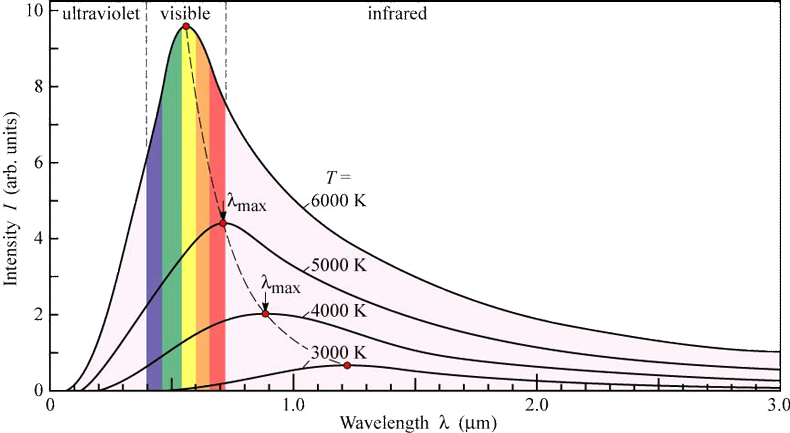
You can clearly how each color (wavelength) reaches it's maximum upto a point & starts declining after.

Classical view predicts this :

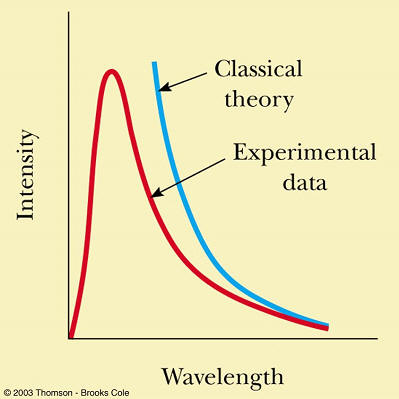
As you keep increasing the intensity, the energy (Temperature) keeps building up continuously. That's the not the case in reality. Each frequency has it's own maximum capacity.

So, as per Classical mechanics, as you keep increasing the intensity - the energy builds up & you move onto higher & higher level on the ramp. There are no such things as levels.

Please keep in mind, all this analogy is just to understand the idea of Quantization - the actual idea of Quantization is bit more complicated than this.

In fact, even Max Planck was skeptical of this very idea to the end. He thought of this Quantization - as some mathematical trick to solve the Ultra-Voilet Catastrophe of Rayleigh-Jeann Law in explaining Blackbody radiation.

It's like using Abacus to Add numbers when we were kids. At that age, it's the best we can operate & perform additions. As we grow up, we move onto much sophisticated methods & tools.

Same with the idea of Quantization or entire Quantum Mechanics for that matter. Right now, it's like Abacus for us to understand Atomic world. Probably in time, we move onto much higher & sophisticated ideas.

I hope it helps.

PS: For better understanding, refer these topics : Black-Body Radiation, Ultra-Voilet Catastrophe, Equipartition Theorem of Statistical Mechanics & Max Planck early contributions to Quantum Mechanics.

Answer 4

By the turn of the 20th C it was accepted that matter was not continuous: matter was made of atoms.

Energy was seen as continuous.

However there was an outstanding puzzle called the blackbody radiation problem. The calculated spectrum did not match that of observation. Still, it was felt that was merely a matter of describing it correctly and would not require a change in the fundamentals of physics. Planck was able to solve this by introducing a discretisation of energy.

At first this was considered as perhaps a mathematical trick; but then Einstein showed how this could be used to explain the photo-electric effect. This began to establish the discrete nature of energy on a former basis; and was in fact the beginnings of QM.

Einstein won the Nobel prize for this work. Surprising, when one might think that he would have won this for SR or GR. But they were still seen then as outré theories.

Answer 5

The concept of energy describes the physical phenomenon that one body can transfer to another body heat and movement. Today it's obvious that for this energy transfer photons are responsible. If your feets on the ground, that means that the electrons from the ground interact with the electrons from your feet. For this please remember that materia is a composition of atom nucleus surrounded (and guarded) by electrons. And this electrons don't touch each other because they have an electric field (this is their nature).

The interaction between electrons happens by the exchange of photons. But the electrons in a body are not free to emit or assimilate any photons. Discovering the spectral lines in the light and seeing the context between elements and their specific lines it was clear that the light emission was a discrete process.

Planck used statistical methods and has had a great intuition. So he found the right formula to describe the black body radiation. The result was the knowledge about the existence of light in portions. Einstein call them light quanta, later named photons.