Reaction Force on String Wrapped Around Circular Peg

Question

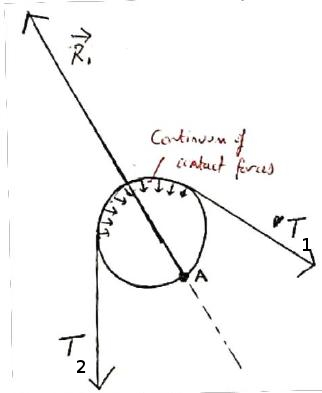
A massless inextensible string is wrapped around a frictionless circular peg. The string is taut, with tension $T_2$ and $T_1$ at the points where it leaves of the pg as shown. The segment wrapped around the peg exerts a continuum of contact forces on the peg, perpendicular to the tangent to the surface of the peg at each point. As a result the peg exerts a net reaction force $R$ on the segment which is the sum of this continuum of contact forces (with directions reversed).

The section of string wrapped around the peg is in mechanical equilibrium. WITHOUT assuming $T_1 = T_2$ (I am attempting to deduce that based on the direction of $R$), how can I deduce that $R$ must be perpendicular to the surface of the peg where the centre of mass of the segment wrapped around it is?

Alternatively, can one prove that $T_1 = T_2$ without assuming that $R$ is perpendicular? Arguments involving torque on the peg do not work in this case as the peg is frictionless.

Answer 1

There are two observations that can be made about this problem.

1) If T1 is not equal to T2, the string will slip on the peg, which is frictionless.

2) If the reaction force from the peg, force R1, is not perpendicular to the peg's surface, there will be a component of R1 that is parallel to the surface of the peg, and the peg will rotate. For a frictionless peg, it is difficult to see how a non-perpendicular reaction can be produced.

Alternative explanation: Assuming that one could load the peg with equal forces as shown, and produce a non-perpendicular reaction force, the peg would continue to rotate as long as the forces were present. No net work would be put into this system because the string producing T1 and T2 would be stationary. However, net work could be produced by the rotating peg. If this was the case, the law of conservation of energy would be violated. Since we know that this can't happen, the reaction force must be perpendicular to the peg's surface.

Answer 2

Assume T1>T2 and T1 and T2 acting in the same direction. The difference T1-T2 will just lead to a slipping of the string over the frictionless peg. This will not cause a reaction force. The 'common' component, i.e. the part of the force that is felt on either side of the peg T2 causes the reaction force R. So R is equal to the minimum of T1 and T2: R=2*min(T1,T2).

Assume now that there is an angle A between T1 and T2. The reaction force will then be the vectorial sum of the two 'common components' or R=2*min(T1,T2)*cos(A/2).

Answer 3

Consider the net torque on the segment of string wrapped around the peg about the peg's centre. The reaction force the peg exerts on the string at each point is radial, and so does not contribute to this net torque. This net torque must therefore equal $\mathbf{T_1} \times \mathbf{r_1} - \mathbf{T_2} \times \mathbf{r_2}$, where $\mathbf{r_1}$ and $\mathbf{r_2}$ are vectors extending from the points of application of $\mathbf{T_1}$ and $\mathbf{T_2}$ respectively to the peg's centre. As the segment of string is massless, to prevent it developing an infinite angular acceleration about the centre of the peg, the net torque about it must be 0. Therefore $T_1 = T_2$. From this it is easy to deduce that $\mathbf{R}$ must be perpendicular to the tangent to the surface of the peg where the centre of mass of the string segment is.