The poles of Feynman propagator in position space

Question

This question maybe related to Feynman Propagator in Position Space through Schwinger Parameter. The Feynman propagator is defined as: $$ G_F(x,y) = \lim_{\epsilon \to 0} \frac{1}{(2 \pi)^4} \int d^4p \, \frac{e^{-ip(x-y)}}{p^2 - m^2 + i\epsilon} $$ $$= \begin{cases} -\frac{1}{4 \pi} \delta(s) + \frac{m}{8 \pi \sqrt{s}} H_1^{(1)}(m \sqrt{s}) & s \geq 0 \\ -\frac{i m}{ 4 \pi^2 \sqrt{-s}} K_1(m \sqrt{-s}) & s < 0\end{cases} $$

using $(+,-,-,-)$ Minkowski signature convention.

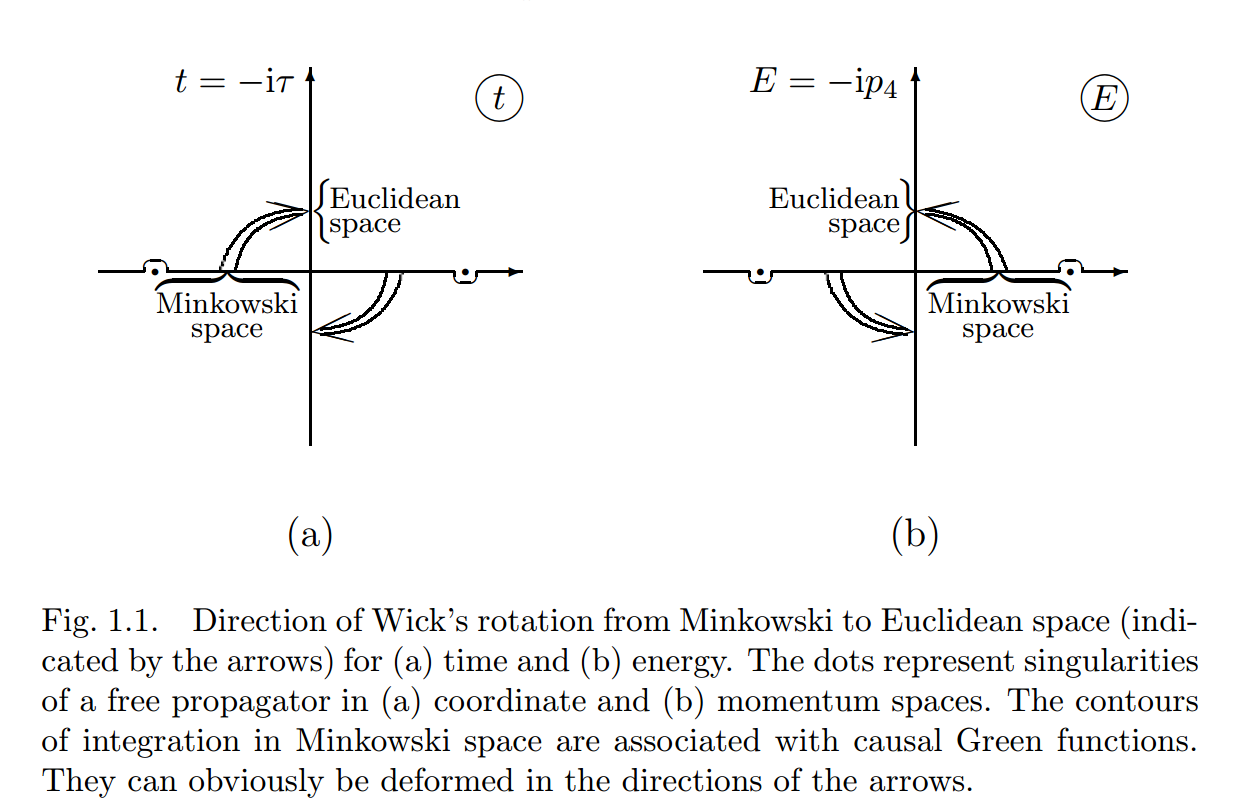
If one wants to apply the trick of Wick rotation, then one should know the position of the poles. It's easy to see that the poles $p_0$ of $\Delta(p)=\frac{1}{p^2 - m^2 + i\epsilon}$ are $p_0 = \pm (\omega - i\epsilon)$. Then, my question is what's the poles $x_0$ or $t$ of $$ \Delta(x) = G_{F,\epsilon}(x) = \int d^4p \, \frac{e^{-ip x}}{p^2 - m^2 + i\epsilon}. $$

I have tried as following:

Because $$ \Delta(p) = \frac{1}{p^2-m^2+i\epsilon} = -i \int_0^\infty d\alpha ~e^{i(p^2 - m^2 +i\epsilon)\alpha} $$ Thus $$ \Delta(x) = \int \frac{d^4 p}{(2\pi)^4} e^{-ipx} \Delta(p) \\ = -i \int_0^\infty d\alpha \int \frac{d^4 p}{(2\pi)^4} ~e^{-ipx+i(p^2 - m^2 +i\epsilon)\alpha} \\ = -i \int_0^\infty d\alpha \frac{1}{(2\pi)^4} [-i\pi^2\alpha^{-2} e^{\frac{-ix^2}{4\alpha}-i(m^2-i\epsilon)\alpha}] $$ Let $\beta = \frac{1}{\alpha}$, then we get $$ \frac{-1}{16\pi^2} \int_0^\infty d\beta~ e^{-\frac{i\beta x^2}{4}-\frac{i(m^2-i\epsilon)}{\beta}} $$ But how to do the last integration and what's the poles $x_0$?

ps: This material by Yuri Makeenko (page 8) gives a figure to show poles and the directions of Wick rotation.

Answer 1

There is an integration formula (see "Table of integrals, series and products" 7ed, p337 section3.324 1st integral) $$\int_0^\infty d\beta \exp\left[-\frac{A}{4\beta}-B\beta\right]=\sqrt{\frac{A}{B}}K_1\left(\sqrt{AB}\right)\qquad [\mathrm{Re}A\ge0, \mathrm{Re}B>0].$$ If $\mathrm{Re}A\ge0, \mathrm{Re}B>0$ is violated, the integral will be divergence.

In your case, $A=4(im^2+\epsilon)$ and $B=ix^2/4$, so $\mathrm{Re}A=4\epsilon>0$ and $\mathrm{Re}B=0$ which does not satisfy the convergent condition. Therefore, to guarantee the convergence of the integral, we should treat $B=ix^2/4$ as the limit $B=\lim_{\epsilon'\rightarrow0+}i(x^2-i\epsilon')/4$. Thus we have $$\Delta(x)=\lim_{\epsilon,\epsilon'\rightarrow0+}\frac{-1}{16\pi^2}\int_0^\infty d\beta \exp\left[-\frac{i\beta (x^2-i\epsilon')}{4}-\frac{i(m^2-i\epsilon)}{\beta}\right]\\ =\lim_{\epsilon,\epsilon'\rightarrow0+}\frac{-1}{4\pi^2}\sqrt{\frac{m^2-i\epsilon}{x^2-i\epsilon'}}K_1\left(\sqrt{-(m^2-i\epsilon)(x^2-i\epsilon')}\right)\\ =\lim_{\epsilon'\rightarrow0+}\frac{-m}{4\pi^2\sqrt{x^2-i\epsilon'}}K_1\left(m\sqrt{-(x^2-i\epsilon')}\right)$$ As a result, the singularity of the propagator is at $x^2-i\epsilon=t^2-\mathbf{x}^2-i\epsilon=0$, i.e. $t=\pm(|\mathbf{x}|+i\epsilon)$.

Actually, the convergent condition of the integral restricts the analytic regime of $\Delta(x)$: $$0<\mathrm{Re}(ix^2)=\mathrm{Re}(it^2)=-\mathrm{Im}(t^2)$$ i.e. $$(2n-1)\pi\le\arg(t^2)=2\arg(t)\le 2n\pi\\ (n-\frac{1}{2})\pi\le\arg(t)\le n\pi$$ Therefore, $\Delta(x)$ only can be analytically continued to the second and the forth quadrants in the complex plane of $t$. In conclusion, the wick rotation in $t$ plane should be clockwise.

Answer 2

The poles lay on the light cone, i.e. $G(x,y) \rightarrow \infty$ for $x\rightarrow y$. To see this, try calculating the integral via residue calculus. First you make a lorentz transformation of the integration variable, so that x has only one entry left (this is either the temporal entry for timelike x or one of the spatial for spacelike). Now for $s = \sqrt{x^2}=0$ there is no possibility of a exponential damping factor regardless of where you close the contour and the integral will diverge.

To see that those are the only singularities, look at the right hand side of your equation: you have the delta-function that diverges for s=0 and the Hankel/Bessel-Funtions that combined with their prefactor both diverge like $1/s$ and have no other singularities.