This question is in reference to the book "Introduction to Modern Physics" by Richtmyer and Kennard, particularly in their discussion of the graphical representation of quantized angular momentum.
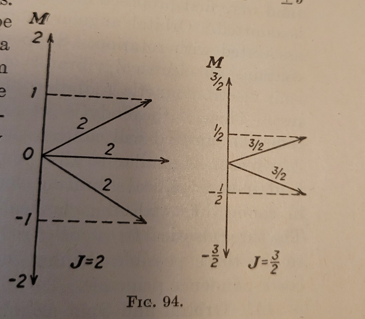
They describe that for a given state $|J,m_j\rangle$, one "pictorial" alternative is to draw vectors with a length of $J$, and to pick the angle such that it projection towards the chosen axis is $m_J$, and they provide the following example (in their notation they write $m_J$ as $M$):
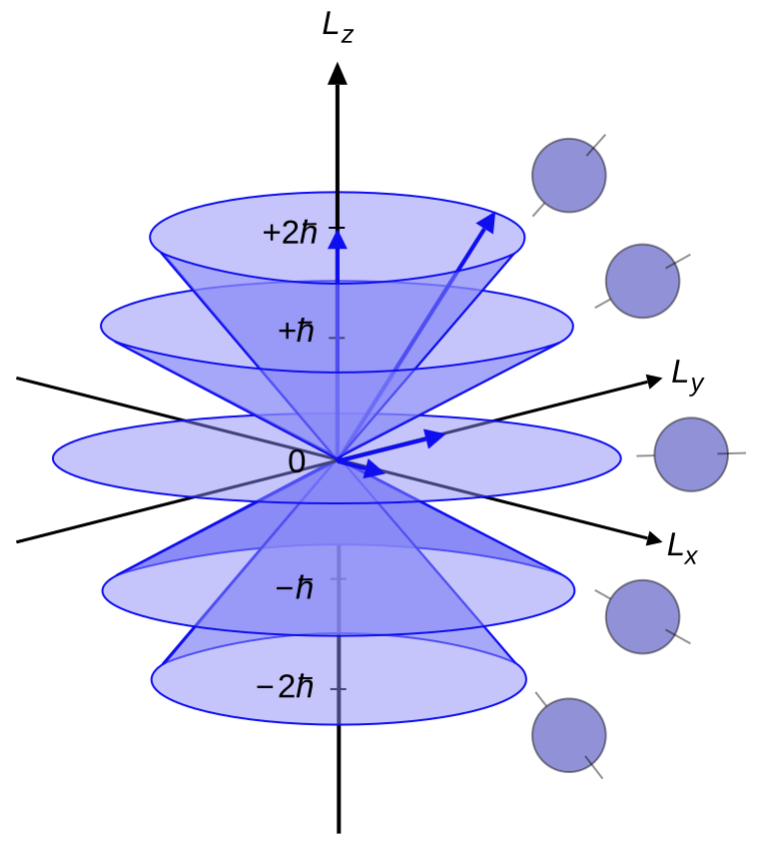
So far so good. Then they suggest that a more accurate representation would be to draw the vector with a length of $\sqrt{J(J+1)}$. They mention that in this case, the vector is necessarily inclined even when $m_J = \pm J$, so it represents better that in general $\vec{J}$ has no definite direction. I believe this agrees with this kind of representation:
But right after that they make this rather cryptic statement:
The square of its component perpendicular to the axis then represents correctly the value of the square of the corresponding component of the angular momentum
First, shouldn't it be the component parallel to the chosen axis? And secondly, I'm not sure why are they mentioning the square of the component $m_J^2$. Are they suggesting a different graphical representation or is this just a simple mistake on their end? The only thing I could think of is that in the particular case $m_J=\pm J$, then the component of the vector perpendicular to the axis is $\sqrt{J}$, so its square does equal $m_J$, but it is definitely not $m_J^2$ (so the statement is still wrong) and this only occurs at the extreme values.