The first is, the INPUT to the transformation should be the "real
time" of the event in the base reference frame S. By "real time", I
mean that if I "perceive" event A as having taken place at time 3s,
but it happened 1 light-second away,
What you are calling the "real time" is the coordinate time and this is what is input into the Lorentz transformation equation. An inertial reference frame is hypothetically set up by creating an infinite network of rulers and synchronised clocks that are all at rest with the origin and all synchronised. Lets say we set up reference frame S with Earth at the origin. We could set up a clock on a space station that is 1 light second away and another on a light station that is 2 light seconds away, all in a straight line. We establish the distances and synchronise the clocks by sending light signals to and fro. Once we done that we can forget about light travel times and only use the times indicated by a cock that is at rest in the reference frame and adjacent to the event being considered. We can have as many clocks as we like, to make sure there is always one next to a given event. he times indicated by these clocks are the coordinate times. Now if we send a light signal from Earth to space station 1, at time zero, then we "measure" that it arrives at the station at coordinate time 2. By "measure" we sometimes mean "imagine"/"perceive"/"calculate"/"predict". In reality the earliest we can have confirmation the signal arrived is at 2 seconds when a confirmation signal returns. A better definition of measure, is what we what we have when we look at the records of the space station and the records of any other confirmation signals from the network of stationary observers and put it all together at some future time. So coordinate or measured time is what you call "real" time.
I mean that if I "perceive" event A as having taken place at time 3s,
but it happened 1 light-second away, and I perceive event B as having
taken place at time 6s, but it happened 2 light-seconds away
The observer at rest on the Earth measures the coordinates of event A as being (x,t) = (3,1) and event B as being (6,2). The time for an object to travel between those two events is 6-3 = 3 seconds according to the measured time the Earth observer. The Earth observer also measures the coordinate distance to be 2-1 = 1 light second. Another observer moving at v relative to the Earth has her own set of synchronised cloaks and she will measure a different coordinate time between those two events and a different coordinate distance between the same two events. All observers with different velocities relative to the Earth we disagree on the coordinate times and coordinate distances between the two events. The only thing they can all agree on is the proper time shown by a clock that is actually transported in a straight line and constant velocity between those two vents so it is the only clock that is actually present at both events. This is called the proper time between the two events or invariant space time interval and is probably what most people would call the "real time". Coordinate times between spatially separated events are made with two different clocks that are spatially separated and differences in the ticking rates of clocks, length contraction of rulers and the simultaneity of relativity cause coordinate times to be observer dependent. All observers also agree on the relative velocity v between frame S and S' and if you enter v, and coordinate distance x and coordinate time t of observer S in the Lorentz transformation, it spits out the coordinate distance x' and the coordinate time t' that will be measured by observer S' and the equation automatically allows for any issues about light travel times.
Usually in SR if it not specifically made clear, we are talking about times and distances where light travel travel times are already accounted for. If light travel times are what we trying to measure and analyse, then it should be made clear that is what we are doing and this is the exception in general. What we "observe" is not necessarily what we measure. Unfortunately what we actually see or observe is light that arrived from an event that may have happed much earlier, but unfortunately when we say observer S observed event A at (x,t) we usually mean "calculated"/"perceived"/"measured" which can be confusing. What we see is the "received time" or the coordinate time plus light travel time and the "observed time" is usually meant to mean the coordinate time or calculated time, unless specifically stated otherwise.
Terrell rotation is an optical illusion due to light travel times and is confusing because nothing actually physically rotates as correctly noted by @m4r35n357 in the comments.
Edit: Added the following diagrams to try and demonstrate how the Lorentz transforms can be related to moving clocks that are adjacent to events rather than the usual spacetime diagrams. The interactive app used for these diagrams is here.
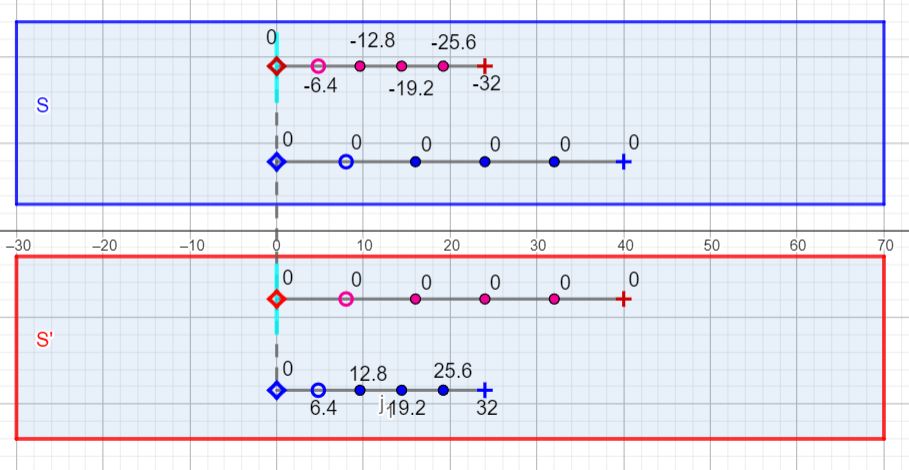
In the diagram the blue points represent clocks at rest in frame S and the red points are clocks at rest in S' which is moving at 0.8c relative to S. The observer at the origin of S' sends out a signal as she passes the observer at the origin of S. The clocks are 8 light seconds apart in both frames.
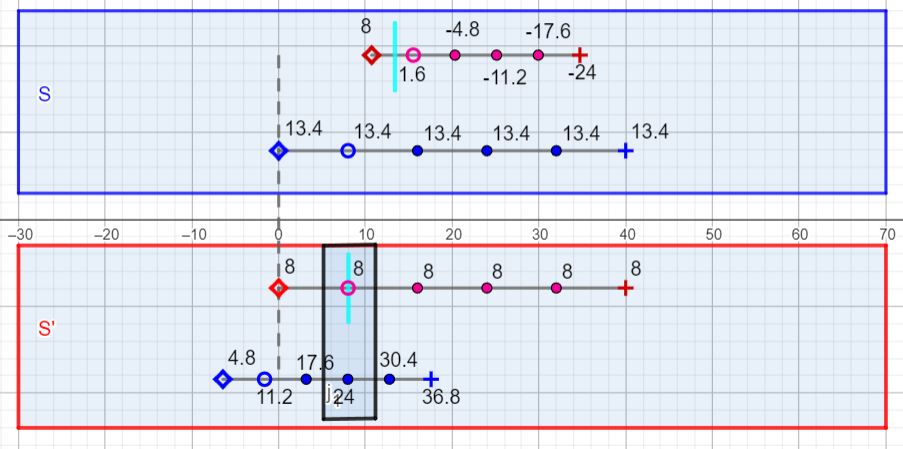
At t' = 8 seconds (and x' = 8 ls) in S' the signal arrives at the second clock (hollow red circle) and this coincides with the blue clock at that location showing t = 24 seconds.
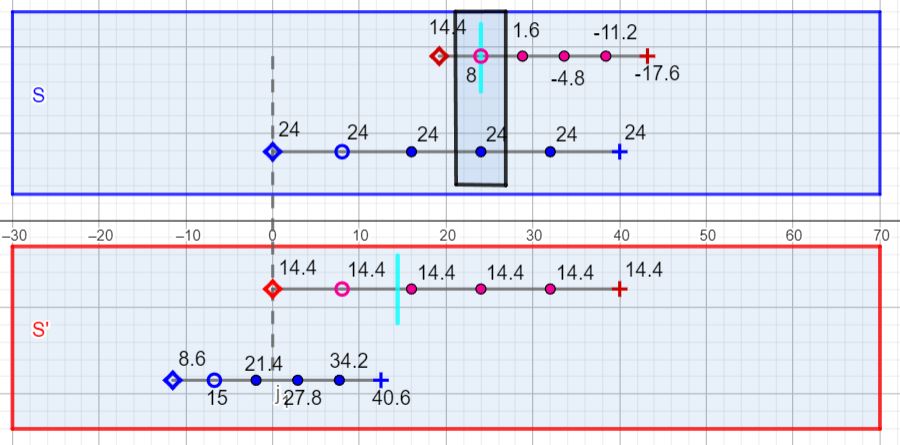
At t = 24 seconds (and x = 24 ls) in S the signal arrives at the second clock (hollow red circle) and this coincides with the red clock at that location showing t' = 8 seconds, agreeing with the reading seen by S' for that event.
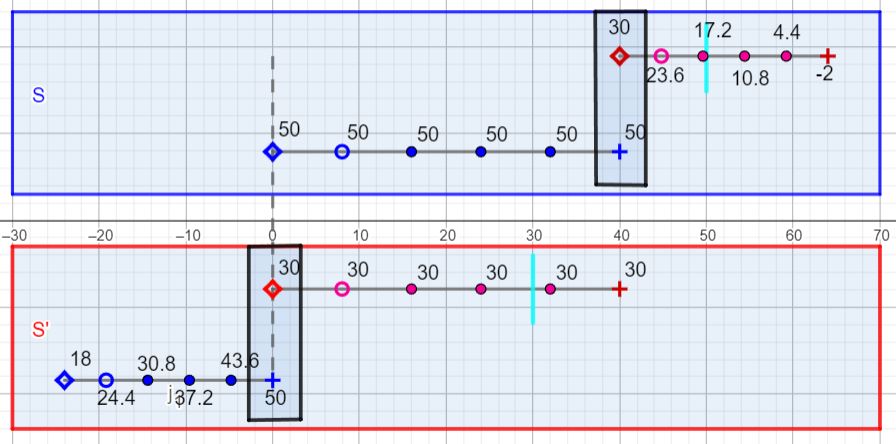
At t = 50 in S and x = 40 in S the observer at the origin of S' (the red diamond) arrives at the blue clock indicated by a plus symbol and the time on her clock indicates 30 seconds at that location for that event. Both observers agree that t=50 and t'=30. However blue measures red to have travelled 40 light seconds and red measure the distance travelled by the blue plus sign clock as 24 light seconds. They differ about the distance due to length contraction.
All measurement are those made by clocks local to any given event and these are the times obtained when performing a Lorentz transformation.
No additions or subtractions due to light travel times are involved.
and thus if I wanted to also find out when the obsever in S' perceives
the event, I'd then also have to do extra calculations to figure out
how long it takes light to get from that event to the observer.
Short answer: Yes.




