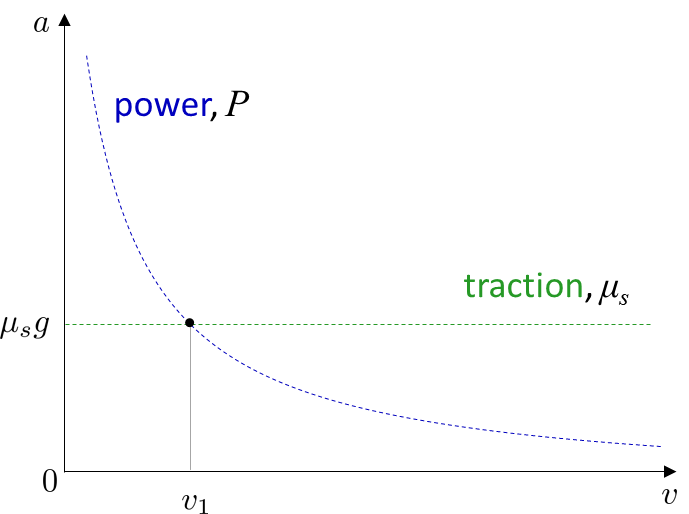
I am currently trying to understand a certain relation between power $P$, velocity $v$ and acceleration $a$.
We are looking at a car driving along a horizontal road and at static friction, and how static friction translates to movement with the cars tires. We are given the maximum static friction coefficient $\mu$ which is $0.6$.
The question I was given is, "at which velocity $v$ will the maximum possible acceleration $a$ be limited by the power of the engine $P$?"
I know that we can write $P(t) = F(t)v(t)$ and therefore $P(t) = ma(t)v(t)$ and with that $v(t) = \cfrac{P(t)}{ma(t)}$
I don't quite understand the relation of the given quantities however. It says, that $v_1 = \frac{P}{ma_{max}}$ is the velocity at which the acceleration is limited by the static friction, where $a_{max} = \mu \cdot g$. And then, that above $v_1$ the acceleration will be limited by the power, and that only then we can bring the entirety of the cars power onto the street without the wheels spinning through.
My questions now:
- What exactly happens up to $v_1$ ?
- Why can we suddenly neglect the static friction once we have hit this threshold velocity of $v_1$? Why can we only bring the full power of the engine onto the street there?
It all makes somewhat sense to me, in an intuitive way, having driven a car many times already, but I am just so damn blind in translating this into these forumlas. I am sure that the answers are probably right in front of my eyes and that I just can't really interpret them yet due to a lack of experience and knowledge and intuition about physics. I would be really happy if someone could elaborate on this in a clear way.