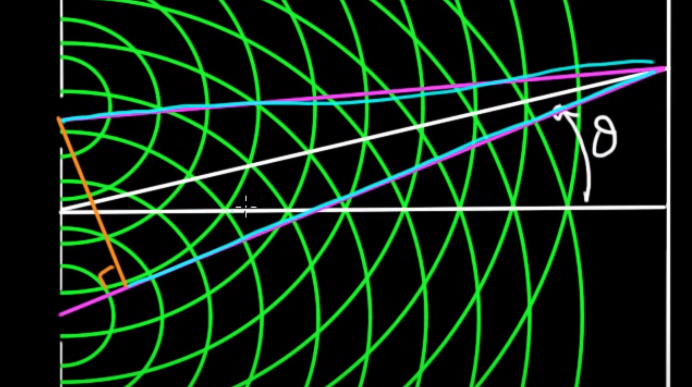
After preparing for my physics test I came across the equation for calculating peaks and valleys of light in double slit experiment - $N\lambda = dsin\theta$. But, looking at how it is proven. I'm wondering if it's accurate.
Because the proof is, as far as I understand, using the fact that on large distances(compared to distance between slits(d)), 2 lines connecting slits will look perpendicular. So, by drawing a line perpendicular to one, the residual distance should be wavelength.
This picture should help.
Here we see the right triangle on the left. But, shouldn't that triangle be the approximation?
I'm concerned about this because, as we move toward more slits, we see something called diffraction grating. Shouldn't this diffraction grating come into effect here too, if our results aren't accurate? Or are our results just slightly innacurate, such that while diffraction grating happens there the real result is actually very close?
Thanks for help. I hope you understand what I'm curious about!