Usually when we work with the path integral, we specify boundary data on spacelike hypersurfaces. But, at least formally, we can also write down path integrals where the boundary has timelike parts. Indeed, there are respectable-looking papers [1,2] proposing that amplitudes can be assigned even in these cases. However, considering such path integrals leads me to the following paradox:
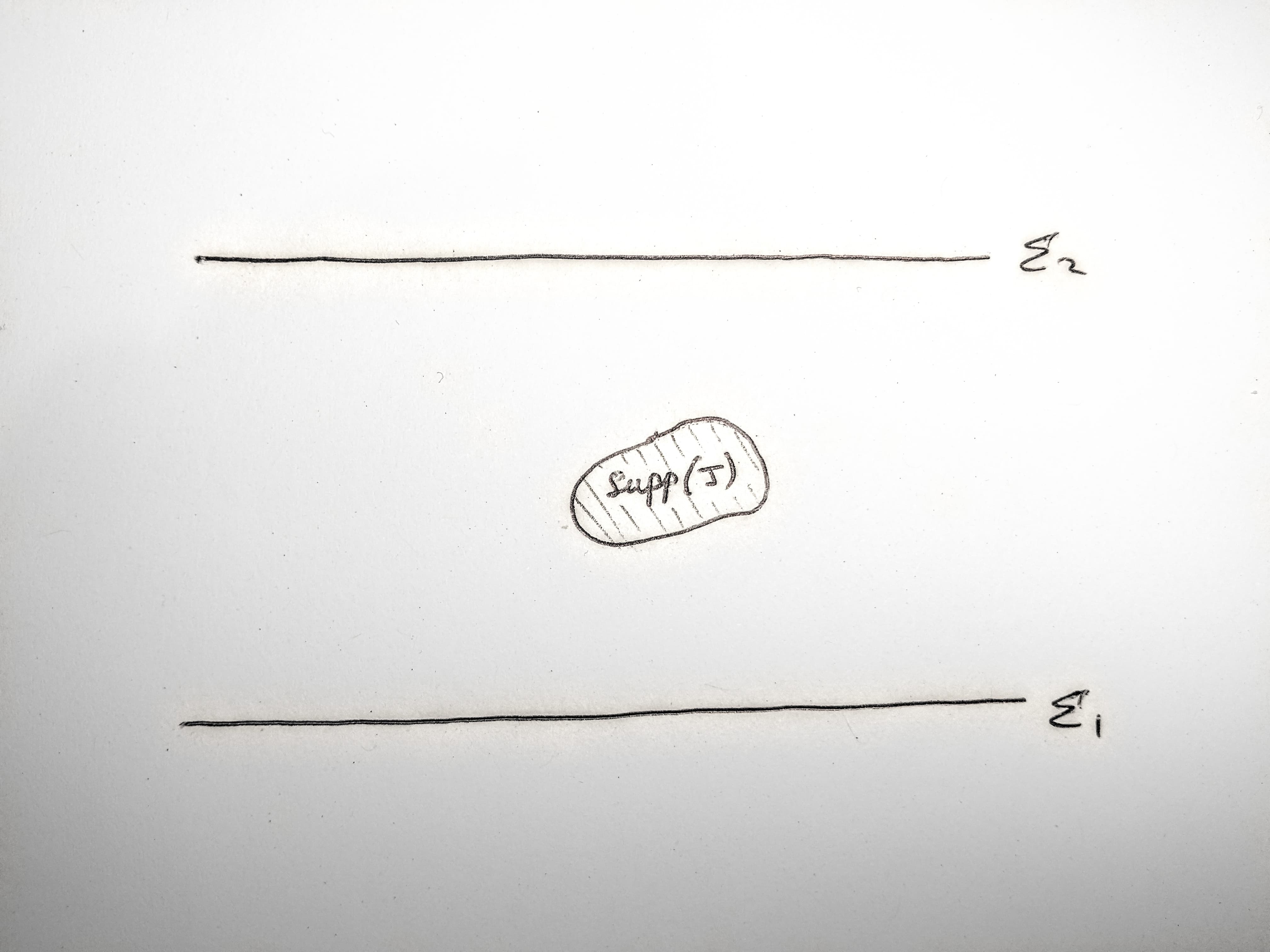
Fix a globally hyperbolic spacetime $(\mathcal{M},g)$. Take it to be 1+1D, to make the diagrams easier. Let $\Sigma_1,\Sigma_2$ be Cauchy surfaces, with $\Sigma_2$ lying to the future of $\Sigma_1$. Suppose QFT amplitudes are given by the path integral with some external source $J$:
$$\langle \phi_2|U_{1\to 2}|\phi_1\rangle = \int_{\phi_{\Sigma_i}=\phi_i} D\phi e^{i\left(S[\phi]+\int dV J(x)\phi(x)\right)}.$$
Suppose further that supp$(J)$ is compact and lies between $\Sigma_1$ and $\Sigma_2$. This setup is depicted in Fig. 1:
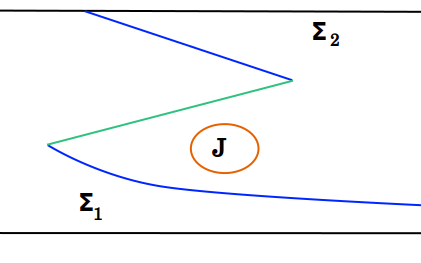
Let's fix an initial state on $\Sigma_1$ and evolve it to $\Sigma_2$. Choose an arbitrary point $p\in\Sigma_2$, and let $\Sigma_2^L$ be the part of $\Sigma_2$ lying to the left of $p$. Now construct a surface $\Sigma$ lying between $\Sigma_1$ and $\Sigma_2$, such that 1) The causal domain of $\Sigma$ is the full spacetime, 2) supp$(J)$ lies entirely in the region between $\Sigma$ and $\Sigma_2$ , and 3) $\Sigma_2^L\subset\Sigma$. Note: we don't require $\Sigma$ to be spacelike everywhere. This is depicted in Fig. 2.
 Note: the middle surface has a typo: it should be labelled $\Sigma$, not $\Sigma_2$
Note: the middle surface has a typo: it should be labelled $\Sigma$, not $\Sigma_2$
Now, by the sewing/gluing property of the path integral, the overall evolution $\Sigma_1\to\Sigma_2$ equals the composition of the evolutions $\Sigma_1\to\Sigma$ and $\Sigma\to\Sigma_2$.$^\dagger$. Evolving $\Sigma_1\to\Sigma$ doesn't depend on $J$, since $J=0$ in this region. Then, evolving $\Sigma\to\Sigma_2$ doesn't change the state on $\Sigma_2^L$. Hence we conclude that the state on $\Sigma_2^L$ doesn't depend on $J$ in any way.
But this can't be right! Since we're allowing $\Sigma$ to have timelike parts, we can make $\Sigma_2^L$ as big as we like - in particular, part of it can lie in the causal future of supp$(J)$, where the state should surely depend on $J$.
What was my mistake?
$\dagger$ The reader might worry about calling e.g. $\Sigma_1\to\Sigma$ an "evolution" if $\Sigma$ isn't spacelike, and hence can't be interpreted as a constant-time slice. But regardless of nomenclature, the path integral does assign complex numbers to field data on $\Sigma_1\cup\Sigma$, and to field data on $\Sigma\cup\Sigma_2$, which sew/glue in the usual way.