A simple and direct answer to the question would be to say that the timelike boundary can't be the issue, because the same paradox can be constructed using a combination of past and future spacelike boundaries:
The full answer is that a timelike boundary is perfectly reasonable, and neither of these situations are paradoxes, as long as you're careful.
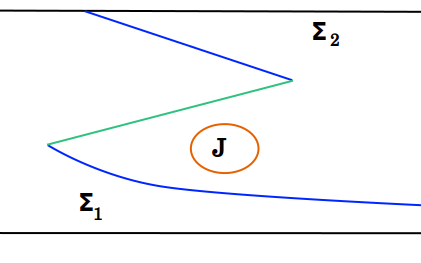
The path integral is a function of the boundary conditions. When you're sewing / composing the two regions, the boundary $\Sigma$ between those two regions stops being a boundary, and becomes just more points internal to the region. So to compose them you need to integrate over all the possible field states on $\Sigma$, with the integrand being the product of the amplitudes for each of the two regions:
$$ \langle\phi_2|U_{1\to2}|\phi_1\rangle = \int \mathcal D \phi(\Sigma)\langle\phi_2|U_{\Sigma\to2}|\phi_\Sigma\rangle\langle\phi_\Sigma|U_{1\to\Sigma}|\phi_1\rangle $$
That's how the influence of $J$ on $\Sigma$ interacts with the influence of $\Sigma$ on $\Sigma_2^L$. This does involve the influence going "backwards" from the second region to the first region, but that's fine. Something like that has to happen given the spacetime diagram, and as you said, it works fine formally if you just think of it in terms of "fields in, amplitudes out".
To take a concrete situation, say that $|\phi_1\rangle$ is a vacuum state, and $J$ produces some photons which will pass through $\Sigma_2^L$. So $U_{\Sigma\to2}$ tells us that there is a large amplitude for $\phi_\Sigma$ to have photons passing through it (informally speaking). If instead $J$ were 0, there would be a large amplitude for $\phi_\Sigma$ to be undisturbed. And if $U_{1\to\Sigma}$ has a boundary condition where photons are coming through $\Sigma$, it will give large amplitudes for photons passing through $\Sigma_2^L$. Otherwise, if $\Sigma$ is undisturbed, there will be large amplitudes for vacuum. So when you perform the integral over all the possible boundary conditions, whether there's a large value for vacuum or for photons at $\Sigma_2^L$ depends on whether there is a large amplitude vacuum or for photons at $\Sigma$. Which in turn depends on $J$.
The timelike part of the boundary is acting like a weird mixture of initial-value and final-value. But the integral of the product of amplitudes is symmetrical, so it doesn't really care which one comes before the other, and doesn't care whether they have a causally messy relationship, like in this case.
One thing to note is that $U_{1\to\Sigma}$ and $U_{\Sigma\to2}$ aren't necessarily unitary, despite the naming. One other thing to note is that you can specify fields on $\Sigma$ that are actually inconsistent with each other, which should cause the path integral to spit out infinitesimal amplitudes in those cases.