Your question is based on an assumption that the vacuum is empty, and matters
(including particles) are things we placed in the empty vacuum.
But the Casimir effect shows that the vacuum is not empty but a dynamical medium.
This led to an emergence point of view of elementary particles: they are quantized collective motions of the vacuum medium.
In one approach, we regard the vacuum as a collection of qubits.
(ie the space is a qubit ocean.) If those qubit form a string-net liquid,
then the quantized collective motions of qubits can give rise to photons, electrons, etc.
So
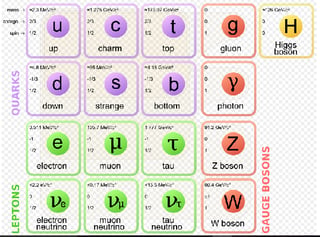
elementary particles, like photons and electrons, are not elementary in the sense that there are underlying theories, such as the quantum qubit model on lattice, from which they can be derived as an effective approximation (see for example our paper arXiv:hep-th/0302201).
Under such an emergence picture, if we examine
elementary particles closely, we see the qubits that form the whole space.
The question weather elementary particles are point-like or not do not make sense within the emergence approach.
The string-net condensation provides a unified origin for gauge interactions and Fermi statistics:
Both elementary gauge bosons (such as photons, gluons) and elementary fermions (such as electrons, quarks) can emerge as quasi-particles in a quantum spin model on lattice
if the quantum spin model has a "string-net condensed state"
as its ground state. An comparison between the string-net approach and the
superstring approach can be found here.
There is a falsifiable prediction from the string-net theory:
all fermions (elementary or composite) must carry gauge charges (see our paper cond-mat/0302460).
The standard model contain composite fermions that are neutral for $U(1)\times SU(2)\times SU(3)$ gauge theory. So according to the string-net theory, the
standard model is incomplete. The correct model should contain extra gauge theory, such as a $Z_2$ gauge theory. So the string-net theory predicts extra
discrete gauge theory and new cosmic strings associated with the new discrete gauge theory.
The emergence approach may also produce (linear) quantum gravity from quantum spin models (see our paper arXiv:0907.1203).
However, the emergence approach (such as the string-net theory), so far, fail to produce the chiral coupling between the $SU(2)$ weak interaction and the fermions.