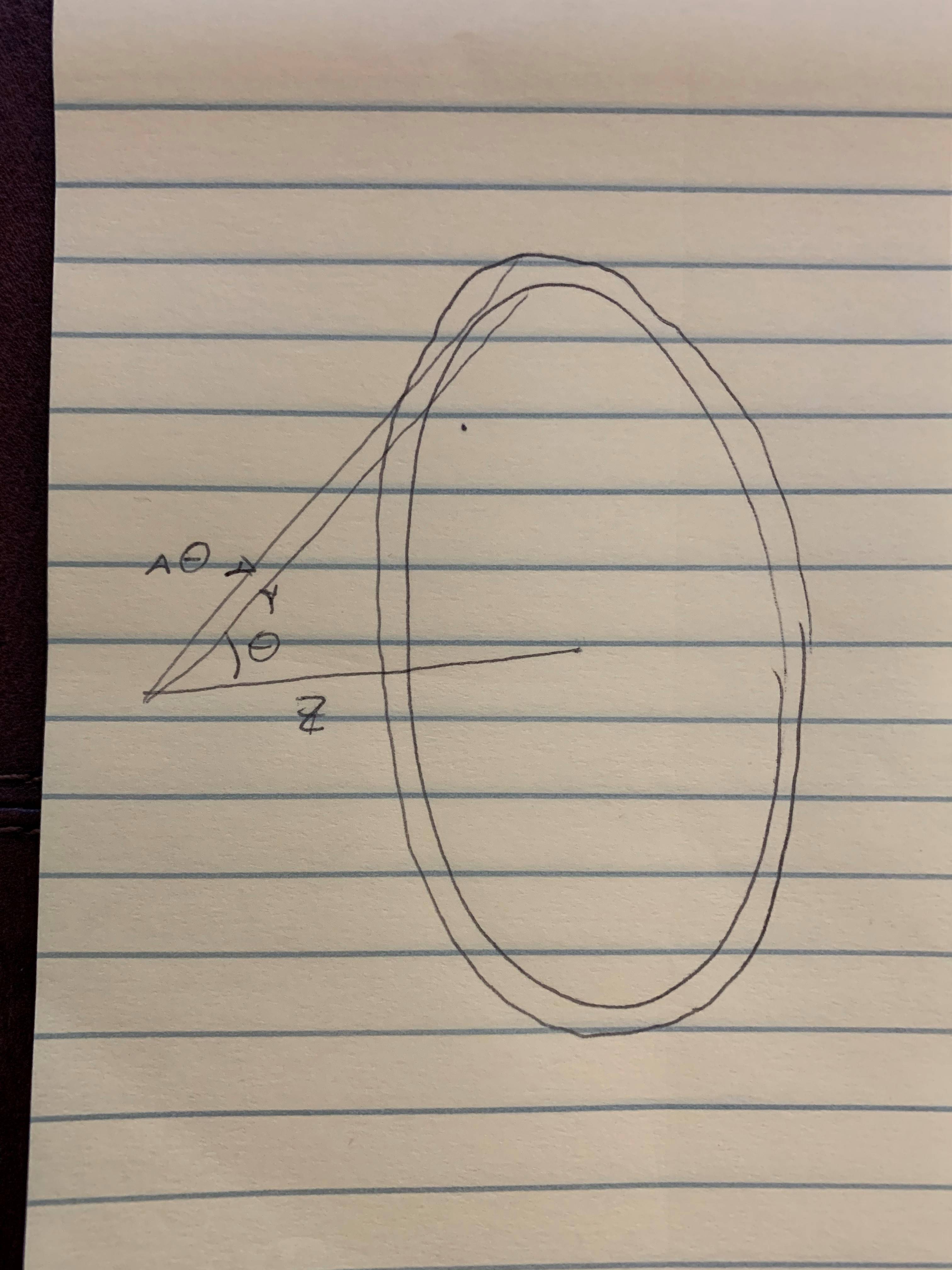In general, the field felt at a particular distance from a particular charge distribution is the product of two factors:
- How much charge you see in a particular direction (i.e. a small angular area), and
- How far away that charge is.
The second factor is relatively simple - the electric field has a $1/r^2$ dependence, so as you move further away from a charge distribution, it will always shrink by $1/r^2$. The first factor is more interesting.
Let's examine how each of these quantities changes when you move further away from a few example charge distributions:
Point charge:
For a point charge, the amount of charge that you see in any particular direction is constant. The point charge is either in the angular cone that you choose, or it isn't, no matter how far away you are. As we already said, moving further away from the charge distribution gives you an automatic $1/r^2$ decrease in the field magnitude. So, multiplying these two factors together gives you $(\text{constant})\times\frac{1}{r^2}\propto 1/r^2$ dependence on distance.
Line charge:
For a line charge, the total charge on a given segment of the line is proportional to the length of the line segment. As you move further away from a line charge, the length of the line segment that is enclosed in a given angular cone increases linearly with distance (you can think of it as the arc length subtended by that angle, given by $s=r\theta$). Since moving further away causes the length of the line enclosed by a given angle to increase, then the total charge within a given angle increases linearly with distance. As before, the second factor gives you a $1/r^2$ dependence, so, in total, we have $r\times1/r^2\propto 1/r$ dependence for this distribution.
Sheet of charge:
For a sheet of charge, the total charge on a given patch of the sheet is proportional to the area of the patch. As you move further away from the sheet, the area enclosed by each angular cone grows quadratically with distance (we already saw that the width of the region enclosed by the cone grows linearly with distance, and area is proportional to squared width). This means that the total charge enclosed by a given angular cone also increases quadratically with distance. So we have $r^2\times 1/r^2\propto\text{constant}$ dependence on distance.
So, ultimately, the constant field as a function of distance is due to two competing factors exactly cancelling each other out for a very particular configuration of charge. The further away you move, the more charge you see in any given direction, but the further away that charge is.