Energy Band of pn junction in thermal equilibrium (Zero bias)

Question

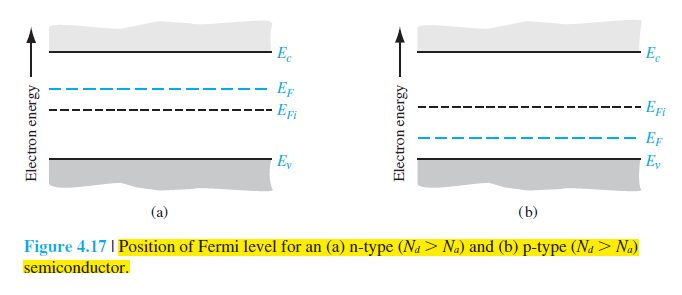
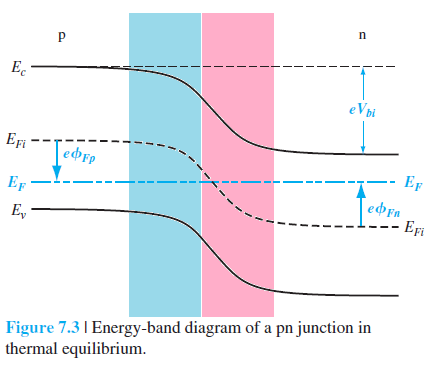
Consider the following diagrams:

Question:

1. Why in thermal equilibrium—the Fermi energy level is constant throughout the entire system? I know the following explanation A gradient in the Fermi level is the driving force for carrier motion:

$$F_n = \frac{D_n}{k_BT} n \frac{\mathrm{d}E_F}{\mathrm{d}x}$$

In equilibrium (zero bias), $F_n = 0$ and therefore $E_F=\text{const}$. but don't get the derivation due to following question.

1. In First diagram why the $E_v$ elevates? Is that mean that the highest energy state in valence band of p-type semiconductor is increase?if yes How? Consider that I know the explanation that you have to make that fermi level in equillibrium condition constant throughout the entire system.
2. See the second figure $E_{Fi}$ in both the figure are equal(atleast in figure) As I joint them How $E_{Fi}$ get increased in p type ,I mean it's condition for intrinsic.

3.They get the expression $$V_{bi}=|\phi_{Fp}|+|\phi_{Fn}|.$$(Is that in figure the $E_{Fi}$ of p-side is equal to $E_c$ of n side if yes How?)

Answer 1

In the first figure it is important to note that the junction between the two materials has a region of negative charge density (in light blue below) and positive charge density (in light red below):

The potential energy of an electron is thus higher on the left side of the junction than the right. That is what is plotted on the graph. An electron at the top of the valence band on the the left side will have more energy than one on the right hand side.

To understand why there is non-zero charge density at the junction, you must understand that p-type materials have a high concentration of positive charge carriers (holes) and n-type materials have a high concentration of negative charge carriers (electrons). It is important to note that for an isolated p-type material there is an immobile negative ion for every positive charge carrier, and vice versa for n-type material, so that overall they are electrically neutral (zero net charge density).

When you bring the two materials into contact, then charge carriers will naturally diffuse from one material to the other. The diffusion of positive charge carriers from p-type to n-type and negative charge carriers from n-type to p-type is entropic in origin, similar to why gas in a container prefers to occupy the entire volume and not, say, some particular half.

The charge transfer driven by entropy causes an electric potential difference between the p-type and n-type materials. This potential difference acts to prevent further diffusion of charge between the two materials. When this electrical force balances the entropic force, the pn-junction is at equilbrium.