15
$\begingroup$

This is really bothering me for a long time, because the math is easy to do, but it's still unintuitive for me.

I understand the "law of the lever" and I can do the math and use the torques, or conservation of energy. or whatever... And I can see that a lever can amplify a force you apply to it if you apply a force on the longer side of the beam.

If I were to look at the molecular lever and see what actually happens when I push on the lever, and I give acceleration to the molecules, how does it actually happen that more force is transmitted to the other side?

Thank you all

p.s I'm looking just for an explanation in terms of forces and acceleration, it's clear to me how to do this in terms of energy or torques

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ Here is a mental model that might help. Think of a series of balls connected in a straight line by very rigid springs floating in space with no external forces acting on it. When it is in its 'rest state' there are no stresses on it. Now give the ball at one end of the assembly a push at right angles from the line. When you do that the spring connecting it to the next ball bends a bit - transmitting the force to the next ball as it tries to straighten out the line. From there to the next ball, and so on. $\endgroup$
    – Snowhare
    Commented Mar 29, 2012 at 1:26
  • $\begingroup$ There is not "more force" transmitted to the other side... regardless of looking at molecules, it is the torque that causes acceleration, not force. $\endgroup$
    – Chris Gerig
    Commented Mar 29, 2012 at 5:55
  • 2
    $\begingroup$ @Chris: No. Emphatically no. It is always force which causes acceleration, and there is more force, just acting over a shorter distance (thereby conserving energy ala $W = \int \vec{F} \cdot d\vec{s}$). It is, however, the torque equation which shows you what the coefficient is. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 29, 2012 at 15:41
  • $\begingroup$ Ah...I see. You question comes down to "What is the origin of the forces that let the bar (or indeed any solid) maintain it's shape?", which means that @BenjaminFranz's comment is the core of a good answer. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 29, 2012 at 17:18
  • $\begingroup$ @BenjaminFranz so it's the electromagnetic forces between molecules that actually generate the extra force? i.e these molecular bonds do not allow the bar to bend and thus create an extra force? $\endgroup$
    – fiftyeight
    Commented Mar 29, 2012 at 17:24

6 Answers 6

Reset to default
8
$\begingroup$

I agree with Benjamin Franz that the ball-and-spring model of a solid is helpful and that when a solid exerts a contact force the bonds between the atoms are distorted in that region. If you take a beam, clamp down its ends, and then apply a force to it off-center, the bonds on the short side are distorted more than the bonds on the long side. Therefore, more force is exerted on the clamp that is closer to the applied force. The diagram below illustrates this: enter image description here

Improve this answer
$\endgroup$
14
  • $\begingroup$ Although the springs from the longer side are less distorted there is a higher quantity of them, so why wouldn't the net distortion equal or be greater than the distortion from the short side? What's the reason? $\endgroup$
    – bruno
    Commented Nov 5, 2014 at 10:59
  • 2
    $\begingroup$ This answer does not explain the phenomenon. For an explanation see my answer of the following question $\endgroup$
    – rmhleo
    Commented Jun 6, 2016 at 14:05
  • $\begingroup$ I don't think this answer answers the question sufficiently. $\endgroup$
    – John Alexiou
    Commented Jul 12, 2017 at 17:49
  • $\begingroup$ @21Brunoh from the point of view of the mass on the right, the only "springs" it would care about are those attached directly to the "balls" its in contact with. Say that the mass on the right has a mass of 2M, and the mass on the left has a mass of M. The rod is floating in space. I think (I'm almost sure) that at small enough distances, the force between bonded atoms in a rigid body is proportional to the distance between them. We want to apply the force (put the triangle) in such a place that the deformation of the rod (between any two particles) on the right side is twice as much... $\endgroup$
    – joshuaronis
    Commented Nov 6, 2019 at 3:30
  • 1
    $\begingroup$ @21Brunoh I agree that the longer part in the vertical direction stretched the same. However, there are more molecules in the longer part. So, if the longer part stretched the same in total than the shorter part, the displacement between individual adjacent molecules in the shorter part must be greater, and thus they must be feeling a greater force from the particles around them, and exerting a greater force on the clamp. $\endgroup$
    – joshuaronis
    Commented Jan 3, 2020 at 20:27
5
$\begingroup$

Even though @Noah's answer provides some insight, I would have to disagree with the statement that "the bonds on the short side are distorted more". The "net" distortion is probably the same on each side.

I will provide a similar figure to @Noah's. There are two black rectangles, that would represent the two weights, but for now let's assume they are rigid. They are also at the same height with the same dimensions.

lever

As you press the rectangles against the beam, the knee (fulcrum) will prevent translation so deformation will occur at the tips. Assuming we keep the same height between the rectangles, the vertical deformation at each of the beam's tips will be the same.

However, the force required in the left rectangle for such deformation will be less than the force required by the rectangle on the right. To give some intuition on why this happens I have drawn the scenario where you would have to bend the longer portion of the beam (L1) and the shorter portion (L2):

enter image description here

I believe that by experience you already know that in the second case (shorter beam) it's much harder to bend the beam and a greater force needs to be applied (*). So if you combine these two cases and return to the original example, you probably understand that indeed a greater force needs to be applied at L2 compared to L1.

If we think in terms of weights instead of two black rectangles, you can now see why a small force at the tip of L1, would require a larger force at L2 to balance out the system, deforming the same amount at each end. In a typical real-life situation the lever would not be as deformed. Nevertheless, the bending action and inherent geometry of the lever still explains this phenomenon.

  • (*) Bonus: If you are wondering why a smaller beam is more difficult to bend compared to a longer one, you can study the beam using a ball-spring model at the atomic level. Thinking of the beam in terms of springs, the longer side has an equivalent axial spring that is much longer than the combined axial springs from shorter side. If you recall from Physics, a shorter spring is much harder to pull than a longer one. You can try it yourself, but the explanation for this is a whole other topic. As you "push" the weight in the longer side of the beam (or black rectangle), inevitably it bends the beam in such a way that "springs" near the top extend and the springs near the bottom compress (see bending moment for more about this). This means there is internal stress, due to axial forces, similar to how you would push a spring. Below you have a rough picture explaining the existence of an axial force (F1). The weight (F) is decomposed into a perpendicular force to the beam (F2) and an axial force (F1). Note that this is an oversimplification, since the internal axial force distribution varies along the cross section.

    enter image description here

  • Extra: A recent discussion with @DS led me to remember that this is a similar situation to force amplification in fluids: what transfers the force from the smaller piston and amplifies it to the larger piston is the pressure and not the actual force. Here it's similar: what amplifies the force is the bending stress inside the beam, promoted by the push at the longer side of the lever and transmitted to the shorter side.

    Regarding why pressure/ stress is transmitted and not force that is probably related to the fact that energy is what is transmitted and not force. Assuming no losses to heat, there is energy conservation (either in the bending of the lever or in the piston example): even though the force is amplified, the distance (to the weight) is decreased so their product ("energy/ work") is the same at either side of the system. If someone wants to add some comments on this part I would greatly appreciate.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Nice! Although you don't need energy :) $\endgroup$
    – joshuaronis
    Commented Jan 3, 2020 at 21:04
  • $\begingroup$ I don't think this answered the question. He wanted a molecular view. $\endgroup$
    – Tough questions
    Commented Feb 27 at 10:01
  • $\begingroup$ In that case wouldn’t the the portion directly touching the fulcrum at the bottom be moved towards the shorter side as the shorter spring applies more force and vice versa for the top $\endgroup$
    – Harrychink
    Commented Nov 9 at 1:08
3
$\begingroup$

There are two fairly straight forward ways to understand this:

  • As a problem in "statics" involving forces and torques on the lever.
  • In terms of conservation on energy between the work done by the person operating the lever and on the load lifted.

Setup

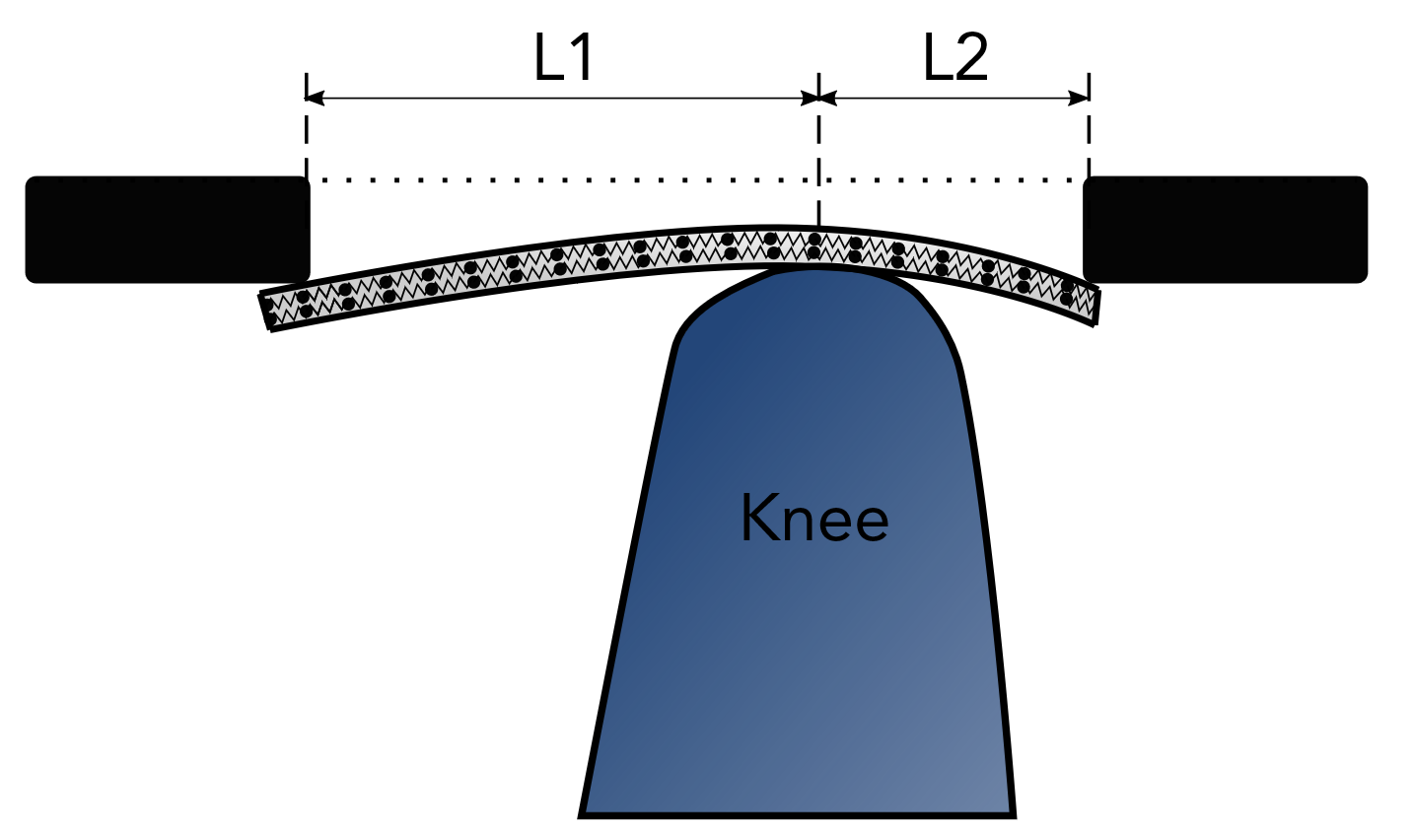
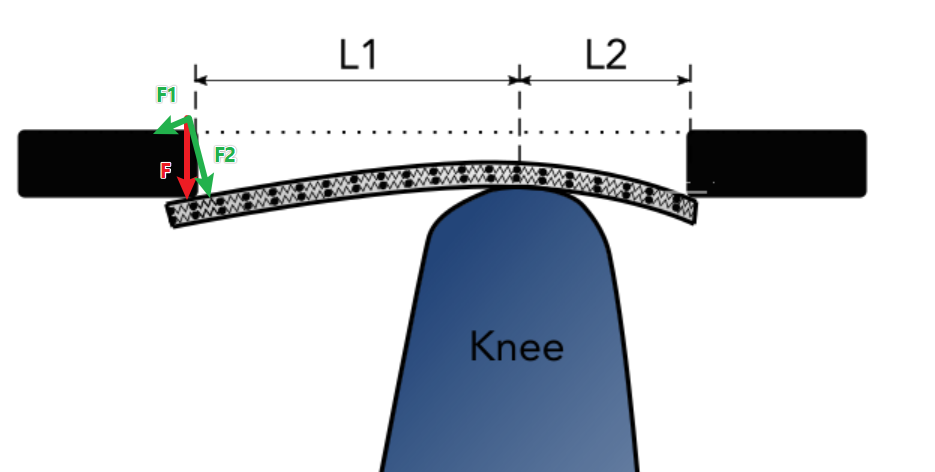
We will, for simplicity, consider the situation where the lever is essentially horizontal (showing that the results hold at other angles is left as an exercise), and will treat the lever as a straight bar of length $l = l_1 + l_2$. Three forces act of the bar, the applied force $F_a$ acts downward atdistance 0, the fulcrum force $F_f$ acts upward at distance $l_1$, and the load $F_l$ acts downward at distance l.

Note that so far I have not said anything about the ratio $l_1/l_2$.

Statics

We require that $\sum F_i = 0$ and $\sum \tau_i = 0$ (the sum of the forces and the sum of the torques acting on the bar are zero). I'll measure the torques around the fulcrum.

$$ -F_a + F_f - F_l = 0 $$ $$ F_a \cdot l_1 + F_f \cdot 0 -F_l \cdot l_2 = 0 $$

Immediately we can see that the system is underconstrained and we have one free parameter; that the weight of the load, so we'll express $F_a$ and $F_f$ in terms of $F_l$.

From the torque equation we get $F_a = \frac{l_2}{l_1} F_l$, and plugging that into the forces equation we get $F_f = (1 + \frac{l_2}{l_1}) F_l$.

Energy concerns

The best case is that the machine wastes no energy; we assume this case.

While the bar moves through a small angle $\alpha$ near the horizontal the applied force moves through a distance $-\alpha \cdot l_1$, and the loaded end through a distance $\alpha \cdot l_2$, computing the work done my each end we get

$$ W_a = -F_a \alpha l_1 $$ $$ W_l = F_l \alpha l_2 $$

By assumption these must add to zero, so

$$ F_a = \frac{l_2}{l_1} F_l $$

as before.

Conclusions

If the load is on the short end then $l_2 < l_1$ and $\frac{l_2}{l_1} < 1$ and you require less force to lift the load, but the load moves a shorter distance.

If the load is on the long end then $l_2 > l_1$ and $\frac{l_2}{l_1} > 1$ and you require more force to lift the load, but the load moves a longer distance.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ This is a good answer, especially the statics part, but the thing that's mostly bothering me is what actually creates the extra force that raises the object and "amplifies" the force I'm doing, Is it the forces between molecules that keep the shape of the bar that actually amplify the force, what helped me in your answer is putting the fulcrum itself into the picture, because it creates some constraint for the system that got me thinking about the molecules that keep the shape of the bar. $\endgroup$
    – fiftyeight
    Commented Mar 29, 2012 at 17:23
  • $\begingroup$ it is the forces between molecules, which are limited only by the breaking strength of the material (which isn't infinite--- you can't move the whole Earth). The transmission of forces conserves the energy, not the force. Force is not a conserved quantity. $\endgroup$
    – Ron Maimon
    Commented Mar 29, 2012 at 17:39
  • $\begingroup$ @fiftyeight: Forces need not be conserved. No one needs to create an extra force. Similar things happen in hydraulics--you can amplify a force in one piston by connecting it to a smaller piston. $\endgroup$
    – Manishearth
    Commented Mar 30, 2012 at 3:12
  • $\begingroup$ The presence of the earth is significant. @Ron One can move the whole earth by jumping -- just not very much. $\endgroup$
    – Peter Morgan
    Commented Mar 30, 2012 at 14:10
  • $\begingroup$ @Peter: The presence of something to push against is significant--that is why you have to include the Fulcrum force for a complete analysis. IN any case, we have both answered the wrong question. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 30, 2012 at 15:34
3
$\begingroup$

The problem presented itself to me a long time ago as "How does the weight hanging from one end of a horizontal rod know how far away are the pivot and the other weight?" This, I found, could be answered only by allowing the rod some thickness, in which it could have an internal structure.

Using an structure consisting of a pin-jointed lattice of struts and ties, the law $F_1x_1=F_2x_2$ emerges simply by applying the force equilibrium condition at each pin joint.

I attach a simplified (possibly over-simplified) version of the argument. enter image description here

This may be criticised because I have given the two sides of the rod differently proportioned structures. The argument works very similarly, and yields the same result, if there is the same lattice structure both sides of the pivot, only more units of the lattice on the long side of the rod.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Why l present this approach, inelegant though it might be considered, is that it derives what is essentially a statics principle using forces and fixed separations, without bringing in movement. I hope that it complements the slicker treatments using work or virtual work. $\endgroup$
    – Philip Wood
    Commented Dec 26, 2021 at 15:28
1
$\begingroup$

It is all relative to the pivot point in the lever, and to energy expended, not the force applied. If the pivot point is one quarter of the levers length, from the bottom of the lever, and you apply a force F, to the top of lever, to move the top through adistance D, the result will be that the bottom of the lever will move through a third of the distance of the top. (IE 3/4 of length divided by 1/4 of length about pivot point). The energy expended at the top of the lever is FxD. Since energy in, equals energy out, and the bottom of the lever moves only 1/3 of D, then the force that is exerted at the bottom of the lever is 3D. (IE 3 times the force applied at the top of the lever) but it has been exerted over a shorter distance. Hope that this is what you are looking for, and hope I have made it clear. It is 60 years since I was taught this.

Improve this answer
$\endgroup$
1
  • $\begingroup$ See my answer, lever can be understood either in terms of energy or in terms of forces. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 29, 2012 at 17:06
1
$\begingroup$

I take dmckee's answer to be flawed because it doesn't mention the earth.

At the coarsest level, the earth accelerates down while the large object accelerates up. At the level of Newtonian mechanics, every action has an equal and opposite reaction.

In more detail, the center of mass of the earth, the fulcrum, the lever, the person who pushes, and the large object, taken together as a single composite system, stays motionless (or, rather, at constant velocity), but the positions and velocities of the five internal components relative to each other are changed by the actions of the contact forces (which we can take ultimately to be non-contact gravitational, electromagnetic and nuclear forces, and an understanding of the constitution of matter does ultimately require QM) that act between them. At this level of modeling, the earth's acceleration (in the model) will be slightly different (and the same as the acceleration of the fulcrum), because part of the person who pushes is also accelerating downwards, and the acceleration of the various parts of the lever would have to be taken into account.

At increasing levels of detail, each of the five components is also composite. I can bend my arm to exert a downward force because I can adjust the internal geometry of my arm relative to another part using chemical energy (which again we can take to be ultimately electromagnetic and nuclear energy, and QM).

Although you tagged this QM, it can be understood moderately well in terms of classical mechanics and EM. The constitution of matter was a concern for late 19C Natural Philosophers, but everything was enough under control that they barely noticed that they were sweeping troubles under the carpet until Planck.

Improve this answer
$\endgroup$
1
  • $\begingroup$ thank you, the QM mechanics tag is a mistake, I didn't mean to put it, I was mainly interested in the EM and Classical Mechanics parts. $\endgroup$
    – fiftyeight
    Commented Mar 30, 2012 at 17:39

Not the answer you're looking for? Browse other questions tagged or ask your own question.