Elastic collision between a point and a rotating solid

Question

Consider a pointwise particle with mass $m_1$ and speed $v_1$, which collides with a solid $S$ of mass $m_2$, rotational inertia matrix $J$, speed $v_2$, and rotational velocity $\omega_2$. I denote by $r$ the vector between the centrum of inertia of the solid and the collision point, and by $n$ the vector normal to the boundary of the solid at the collision point. The collision is elastic. I think that these data are enough to obtain the velocities and angular momentum of the solid after the collision. The thing is that I'm unable to write down correctly the conservation of momemtum, and to obtain the right solution. I was also unable to find a good answer to this on internet... Could you help me please?

I should probably mention that I'm doing mathematics, and I have not had a physics class for a long time ago...

That might be obvious, but this is in a nonrelativistic context, though the Lorentzian problem also interest me.

Answer 1

The two bodies exchange (scalar) momentum $p$ along the contact normal $\boldsymbol{n}$, and through the contact point $\boldsymbol{r}$ (relative to the center of mass).

Here is a schematic of the situation

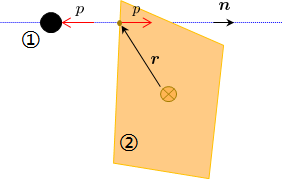
Linear momentum is inherently balanced because whatever momentum vector $p\, \boldsymbol{n}$ is added to (2) it is subtracted from (1).

$$ m_1 \boldsymbol{v}_1 + m_2 \boldsymbol{v}_2 = \left( m_1 \boldsymbol{v}_1 -p \boldsymbol{n} \right) + \left(m_2 \boldsymbol{v}_2 + p \boldsymbol{n} \right) $$

$$ m_1 \boldsymbol{v}_1 + m_2 \boldsymbol{v}_2 = m_1 \boldsymbol{v}_1^\star + m_2 \boldsymbol{v}_2^\star $$

where $\square^\star$ are the values after the impact.

Similar argument can be made for angular momentum, as the vector $\boldsymbol{r} \times p\, \boldsymbol{n}$ is added to (2) and subtracted from (1).

$$ \boldsymbol{r} \times m_1 \boldsymbol{v}_1 + \mathbf{J}_2 \boldsymbol{\omega}_2 = \left( \boldsymbol{r}\times m_1 \boldsymbol{v}_1 - \boldsymbol{r} \times p\,\boldsymbol{n} \right) + \left( \mathbf{J}_2 \boldsymbol{\omega} + \boldsymbol{r}\times p\,\boldsymbol{n} \right) $$

$$ \boldsymbol{r} \times m_1 \boldsymbol{v}_1 + \mathbf{J}_2 \boldsymbol{\omega}_2 = \boldsymbol{r} \times m \boldsymbol{v}_1^\star + \boldsymbol{r} \times \mathbf{J}_2 \boldsymbol{\omega}_2^\star $$

The problem boils down to finding the one scalar momentum exchange value $p$ (usually called the impulse). This is accomplished by the law of contacts, relating the bounce relative speed to the impact relative speed. Notice I said speed and not velocity, as only the velocity along the normal $\boldsymbol{n}$ is important. The law states that $v_{\rm bounce} =-\epsilon\, v_{\rm impact}$, where $\epsilon$ is the coefficient of restitution.

Here velocities are transformed from the center of mass to the contact point and projected along the contact normal.

$$ \begin{aligned} v_{\rm impact} & = \boldsymbol{n} \cdot \left( \boldsymbol{v}_1 - \boldsymbol{v}_2 + \boldsymbol{r} \times \boldsymbol{\omega}_2 \right) \\ v_{\rm bounce} & = \boldsymbol{n} \cdot \left( \boldsymbol{v}_1^\star - \boldsymbol{v}_2^\star + \boldsymbol{r} \times \boldsymbol{\omega}_2^\star \right) \end{aligned}$$

and from the conservation of momentum you have

$$ \left. \begin{aligned} m_1 \boldsymbol{v}_1^\star & = m_1 \boldsymbol{v}_1 - p\,\boldsymbol{n} \\ m_2 \boldsymbol{v}_2^\star & = m_2 \boldsymbol{v}_2 + p\,\boldsymbol{n} \\ \mathbf{J}_2 \boldsymbol{\omega}_2^\star &= \mathbf{J}_2 \boldsymbol{\omega} + (\boldsymbol{r} \times \boldsymbol{n}) p \end{aligned} \; \right\} \; \begin{aligned} \boldsymbol{v}_1^\star & = \boldsymbol{v}_1 - \tfrac{1}{m_1} p \boldsymbol{n} \\ \boldsymbol{v}_2^\star & = \boldsymbol{v}_2 + \tfrac{1}{m_2} p \boldsymbol{n} \\ \boldsymbol{\omega}_2^\star &= \boldsymbol{\omega}_2 + \mathbf{J}_2^{-1} ( \boldsymbol{r} \times \boldsymbol{n} )p \end{aligned} $$

The final equation compiled from the above and to be solved for $p$ is

$$ \boldsymbol{n} \cdot \left( - \tfrac{1}{m_1} \boldsymbol{n} - \tfrac{1}{m_2} \boldsymbol{n} + \boldsymbol{r}\times \mathbf{J}_2^{-1} ( \boldsymbol{r} \times \boldsymbol{n} ) \right) p = -(1+\epsilon)\, \boldsymbol{n} \cdot \left( \boldsymbol{v}_1 - \boldsymbol{v}_2 + \boldsymbol{r}\times \boldsymbol{\omega}_2 \right) $$

$$ \boxed{ p = \frac{(1+\epsilon)\, \boldsymbol{n} \cdot \left( \boldsymbol{v}_1 - \boldsymbol{v}_2 + \boldsymbol{r}\times \boldsymbol{\omega}_2 \right)}{ \tfrac{1}{m_1} + \tfrac{1}{m_2} -\boldsymbol{n} \cdot \left( \boldsymbol{r}\times \mathbf{J}_2^{-1} ( \boldsymbol{r} \times \boldsymbol{n} ) \right)} } $$

Now back substitute $p$ in the expressions above for $\boldsymbol{v}_1^\star$, $\boldsymbol{v}_2^\star$ and $\boldsymbol{\omega}^\star$.

_{Note that $\cdot$ is the vector dot product, and $\times$ the vector cross product. Also note that $\boldsymbol{n}\cdot\boldsymbol{n}=1$.}